Answered step by step
Verified Expert Solution
Question
1 Approved Answer
1. (b) (I) (II) (ii) Classify the following first order differential equations: dy 3 + 2xy = x dx (1 mark) (x-4xy-2y2. + -2x)
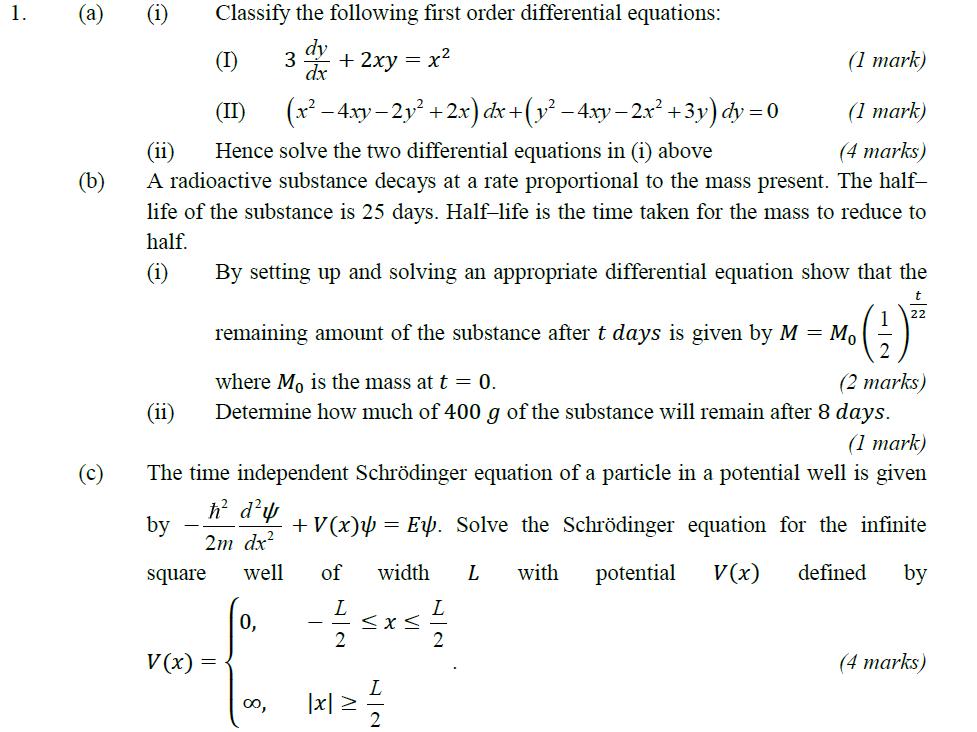
1. (b) (I) (II) (ii) Classify the following first order differential equations: dy 3 + 2xy = x dx (1 mark) (x-4xy-2y2. + -2x) dx + (y-4xy-2x+3y) dy =0 (1 mark) (4 marks) Hence solve the two differential equations in (i) above A radioactive substance decays at a rate proportional to the mass present. The half- life of the substance is 25 days. Half-life is the time taken for the mass to reduce to half. By setting up and solving an appropriate differential equation show that the remaining amount of the substance after t days is given by M = Mo where Mo is the mass at t = 0. (2 marks) (ii) Determine how much of 400 g of the substance will remain after 8 days. (1 mark) The time independent Schrdinger equation of a particle in a potential well is given h dy 2m dx by square +V(x) = E. Solve the Schrdinger equation for the infinite well of width L with potential V(x) defined by L L - 0, V(x) = 2 00, |x| 22 (4 marks)
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


