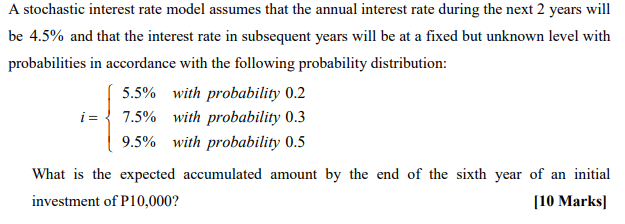
1. Basketball. Suppose that against a certain opponent the number of points the MIT

basketaball team scores is normally distributed with unknown mean ? and unknown variance, ?2. Suppose that over the course of the last 10 games between the two teams MIT

scored the following points:

59, 62, 59, 74, 70, 61, 62, 66, 62, 75

(a) Compute a 95% t-confidence interval for ?. Does 95% confidence mean that the

probability ? is in the interval you just found is 95%?

(b) Now suppose that you learn that ?2 = 25. Compute a 95% z-confidence interval for

?. How does this compare to the interval in (a)?

(c) Let X be the number of points scored in a game. Suppose that your friend is a

confirmed Bayesian with a priori belief ? ? N(60, 16) and that X ? N(?, 25). He computes

a 95% probability interval for ?, given the data in part (a). How does this interval compare

to the intervals in (a) and (b)?

(d) Which of the three intervals constructed above do you prefer? Why?

2. The volume in a set of wine bottles is known to follow a N(, 25) distribution. You

take a sample of the bottles and measure their volumes. How many bottles do you have to

sample to have a 95% confidence interval for with width 1?

3. Suppose data x1, . . . , xn are i.i.d. and drawn from N(, ?2), where and ? are unknown.

Suppose a data set is taken and we have n = 49, sample mean x = 92 and sample standard

deviation s = 0.75.

Find a 90% confidence interval for .

4. You do poll to see what fraction p of the population supports candidate A over

candidate B.

(a) How many people do you need to poll to know p to within 1% with 95% confidence.

(b) Let p be the fraction of the population who prefer candidate A. If you poll 400 people,

how many have to prefer candidate A to so that the 90% confidence interval is entirely

above p = 0.5

11. Suppose we have 8 teams labeled T1, . . . , T8. Suppose they are ordered by placing

their names in a hat and drawing the names out one at a time.

(a) How many ways can it happen that all the odd numbered teams are in the odd

numbered slots and all the even numbered teams are in the even numbered slots?

(b) What is the probability of this happening?

12. (Taken from the book by Dekking et. al. problem 4.9) The space shuttle has 6 O-rings

(these were involved in the Challenger disaster). When launched at 81? F, each O-ring has

a probability of failure of 0.0137 (independent of whether other O-rings fail).

(a) What is the probability that during 23 launches no O-ring will fail, but that at least

one O-ring will fail during the 24th launch of a space shuttle?

(b) What is the probability that no O-ring fails during 24 launches

2. (70 p) A company producing electric relays has three manufacturing plants (plant 1, plant 2, plant 3) producing 50, 30, and 20 percent, respectively, of its product. Suppose that the probability that a relay manufactured by plant 1 is defective is 0.02, the probability that a relay manufactured by plant 2 is defective is 0.05, and the probability that a relay manufactured by plant 3 is defective is 0.01. If a relay produced by this company is randomly selected and found to be defective, what is the probability that it was manufactured by plant 2?A stochastic interest rate model assumes that the annual interest rate during the next 2 years will be 4.5% and that the interest rate in subsequent years will be at a fixed but unknown level with probabilities in accordance with the following probability distribution: 5.5% with probability 0.2 7.5% with probability 0.3 9.5% with probability 0.5 What is the expected accumulated amount by the end of the sixth year of an initial investment of P10,000? [10 Marks]For the matrices in parts a and b, determine whether P is a stochastic matrix. If P is not a stochastic matrix, explain why not. 0 0.3 a. P = 1 0.7 b. P = 0.1 1.1 0.9 - 0.1 a. Choose the correct answer below. O A. The matrix is a stochastic matrix because its rows are all probability vectors. O B. The matrix is not a stochastic matrix because at least one of its columns is not a probability vector. O C. The matrix is not a stochastic matrix because at least one of its rows is not a probability vector. O D. The matrix is a stochastic matrix because its columns are all probability vectors.More on Matrices; Application EXERCISE6 (6 points) Difficulty: Moderate Theory: A vector with nonnegative entries is called a probability vector if the sum of its entries is 1. A square matrix is called right stochastic matrix if its rows are probability vectors; a square matrix is called a left stochastic matrix if its columns are probability vectors; and a square matrix is called a doubly stochastic matrix if both the rows and the columns are probability vectors. **Write a MATLAB function function P=stochastic (A) which accepts a square matrix A with nonnegative entries as an input. The output will be a stochastic matrix P as defined below. You should use the row vectors S1=sum(A, 1) and S2=transpose(sum(A,2)) in your code along with the conditional "if" statements. You could also employ a logical function all. **First, the function has to check whether a matrix A contains both a zero column and a zero row. If yes, the output has to be a message "A is not stochastic and cannot be scaled to stochastic". (Meaning: it is neither right- nor left-stochastic and cannot be scaled to either of them.) The outputs in this case are S1, S2, and P = [ ]. **Then, the function checks whether a matrix A is: (1) doubly stochastic, (2) only left stochastic, (3) only right stochastic, or (4) neither left nor right stochastic but can be scaled to stochastic. 6