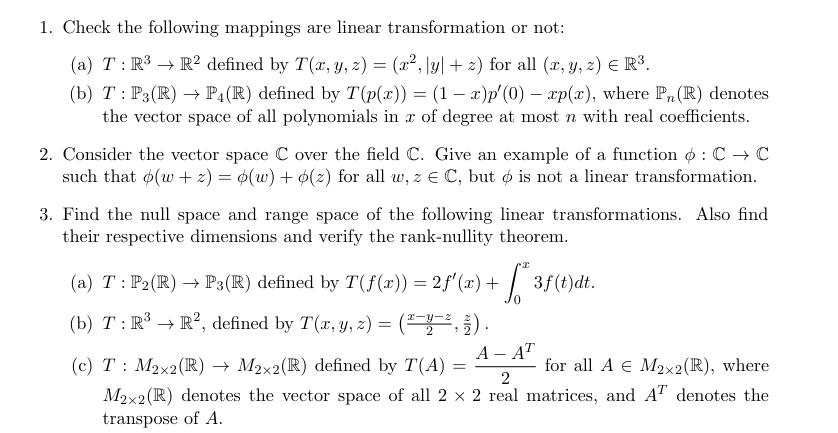
1. Check the following mappings are linear transformation or not: (a) T: R3 R2 defined by T(x, y, z) = (x, |y| + z) for all (x, y, z) = R. (b) T: P3(R) P4(R) defined by T(p(x)) = (1-x)p' (0) xp(x), where PR (R) denotes the vector space of all polynomials in x of degree at most n with real coefficients. 2. Consider the vector space C over the field C. such that (w+) = (w)+(z) for all w, z Give an example of a function : C C C, but is not a linear transformation. 3. Find the null space and range space of the following linear transformations. Also find their respective dimensions and verify the rank-nullity theorem. x (a) T : P2(R) P3(R) defined by T(f(x)) = 2f'(x) + [* 3(t)dt. (b) T: R3 R2, defined by T(x, y, z) = (, }) . (, A-AT = for all A M2x2(R), where 2 (c) T M2x2(R) M22 (R) defined by T(A) M22 (R) denotes the vector space of all 2 2 real matrices, and AT denotes the transpose of A.