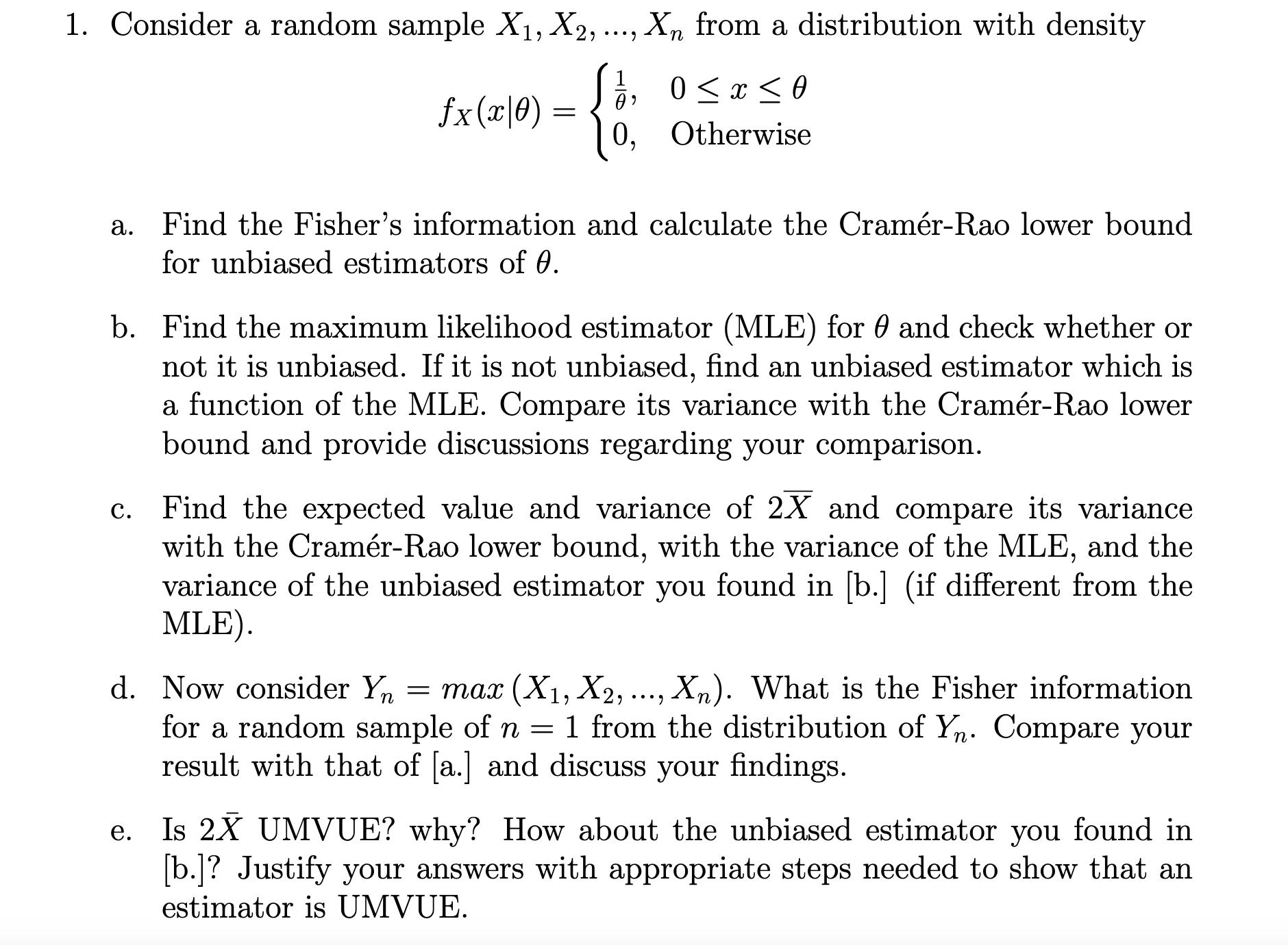
1. Consider a random sample X1, X2, ..., Xn from a distribution with density fx(x|0) = {6 2 0 x 0 0, Otherwise a. Find the Fisher's information and calculate the Cramr-Rao lower bound for unbiased estimators of 0. b. Find the maximum likelihood estimator (MLE) for 0 and check whether or not it is unbiased. If it is not unbiased, find an unbiased estimator which is a function of the MLE. Compare its variance with the Cramr-Rao lower bound and provide discussions regarding your comparison. C. Find the expected value and variance of 2X and compare its variance with the Cramr-Rao lower bound, with the variance of the MLE, and the variance of the unbiased estimator you found in [b.] (if different from the MLE). Yn d. Now consider Y = max (X1, X2, ..., Xn). What is the Fisher information for a random sample of n = 1 from the distribution of Y. Compare your result with that of [a.] and discuss your findings. e. Is 2X UMVUE? why? How about the unbiased estimator you found in [b.]? Justify your answers with appropriate steps needed to show that an estimator is UMVUE.