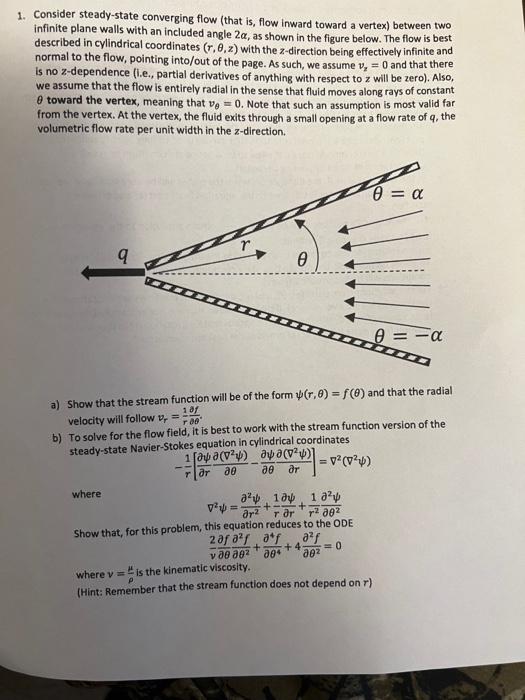
1. Consider steady-state converging flow (that is, flow inward toward a vertex) between two infinite plane walls with an included angle 2, as shown in the figure below. The flow is best described in cylindrical coordinates (r,,z) with the z-direction being effectively infinite and normal to the flow, pointing into/out of the page. As such, we assume vz=0 and that there is no z-dependence (i.e., partial derivatives of anything with respect to z will be zero). Also, we assume that the flow is entirely radial in the sense that fluid moves along rays of constant toward the vertex, meaning that v=0. Note that such an assumption is most valid far from the vertex. At the vertex, the fluid exits through a small opening at a flow rate of q, the volumetric flow rate per unit width in the z-direction. a) Show that the stream function will be of the form (r,)=f() and that the radial velocity will follow vr=r1f. b) To solve for the flow field, it is best to work with the stream function version of the steady-state Navier-Stokes equation in cylindrical coordinates r1[r(2)r(2)]=2(2) where 2=r22+r1r+r2122 Show that, for this problem, this equation reduces to the ODE v2f22f+44f+422f=0 where v= is the kinematic viscosity. (Hint: Remember that the stream function does not depend on r ) F()==qf where is half the wedge angle and q is the flow rate. Introduce the above definitions to come up with a dimensionless ODE that should be third-order in F(). d) Assuming no-slip conditions at the walls, provide two boundary conditions, stating them in terms of both vr() and F(). To solve the third-order equation in part (c), one additional condition is required. Based on the information already provided in this problem, state one additional condition that can be used to fully specify solutions. (Hint: The final condition does not need to be a boundary condition or require consideration of limits or limiting behavior) e) In the limit that 0, the distance between the plates will be very small and we obtain the lubrication limit. Apply this limit to the equation from part (c) and provide a solution. f) For the Reynold's number defined as Re=v2qa, obtain a solution to the equation from part (c) in the creeping flow limit. g) Find a solution to the equation from part (c) in the inviscid flow limit. In obtaining this solution, do not apply the no-slip boundary conditions (a solution utilizing these conditions will be impossible anyway). h) For the solutions from parts (e), (f), and (g), determine if the flows are irrotational (i.e., the vorticity is zero everywhere). If there are flows that are not irrotational, in what limit (e.g., values of r or ) will they become approximately irrotational? in this limit, which solution from parts (e), (f), or (g) provides the best approximation