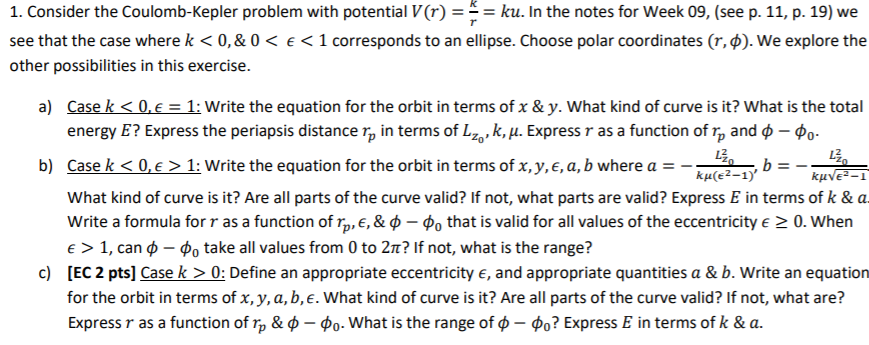
1. Consider the Coulomb-Kepler problem with potential V(r) = = = ku. In the notes for Week 09, (see p. 11, p. 19) we see that the case where k < 0, & 0 < < 1 corresponds to an ellipse. Choose polar coordinates (r, p). We explore the other possibilities in this exercise. a) Case k < 0, = 1: Write the equation for the orbit in terms of x & y. What kind of curve is it? What is the total energy E? Express the periapsis distance rp in terms of L,k, u. Express r as a function of rand -- L kue-1 b) Case k < 0, > 1: Write the equation for the orbit in terms of x, y, e, a, b where a = b ku(e-1)' What kind of curve is it? Are all parts of the curve valid? If not, what parts are valid? Express E in terms of k & a. Write a formula for r as a function of p, , & $ that is valid for all values of the eccentricity > 0. When > 1, can take all values from 0 to 2? If not, what is the range? c) [EC 2 pts] Case k > 0: Define an appropriate eccentricity E, and appropriate quantities a & b. Write an equation for the orbit in terms of x, y, a, b, e. What kind of curve is it? Are all parts of the curve valid? If not, what are? Express r as a function of rp & -o. What is the range of $-o? Express E in terms of k & a. Bro