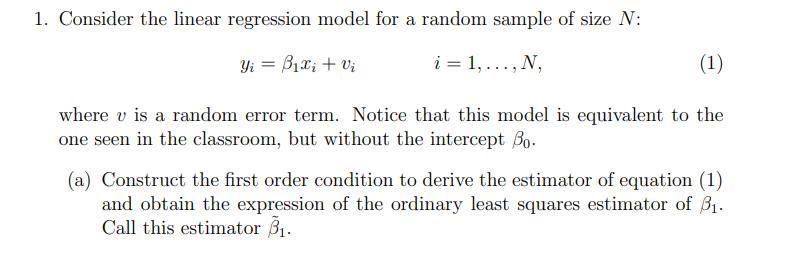
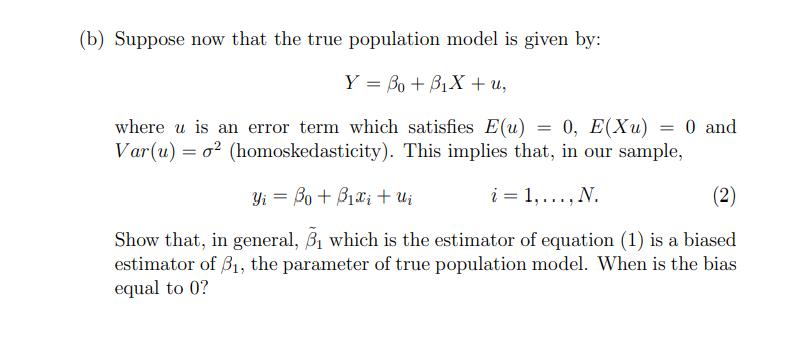
1. Consider the linear regression model for a random sample of size N: Yi = Bii ti i = 1, ..., N, (1) where vis a random error term. Notice that this model is equivalent to the one seen in the classroom, but without the intercept Bo. (a) Construct the first order condition to derive the estimator of equation (1) and obtain the expression of the ordinary least squares estimator of 3. Call this estimator 3. (b) Suppose now that the true population model is given by: Y = Bo + BiX +u, where u is an error term which satisfies E(u) 0, E(Xu) = 0 and Var(u) = o (homoskedasticity). This implies that, in our sample, Yi Bo + xi + Uj i = 1,..., N. (2) Show that, in general, 3 which is the estimator of equation (1) is a biased estimator of 3, the parameter of true population model. When is the bias equal to 0? = (c) Find the variance of B. Is a consistent estimator of uy? [Prove it is, or prove it is not].