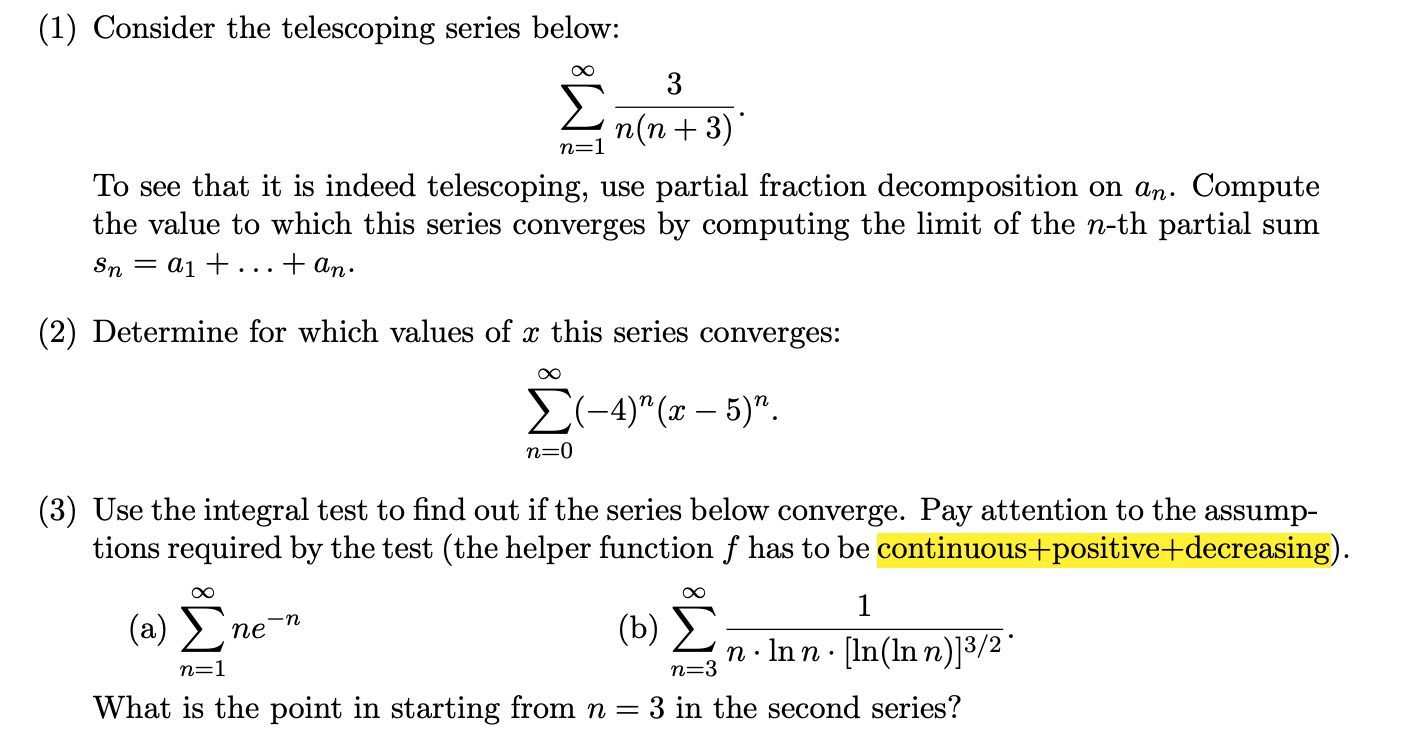
(1) Consider the telescoping series below: 3 n(n + 3)* n=1 To see that it is indeed telescoping, use partial fraction decomposition on an. Compute the value to which this series converges by computing the limit of the n-th partial sum Sn = a1 + ... +An (2) Determine for which values of x this series converges: (-4)" ( - 5)". n=0 Use the integral test to find out if the series below converge. Pay attention to the assump- tions required by the test (the helper function f has to be continuous+positive+decreasing). (b) () -n ne What is the point in starting from n = n=1 n=3 1 n ln n [ln(ln n)]/2 : 3 in the second series?