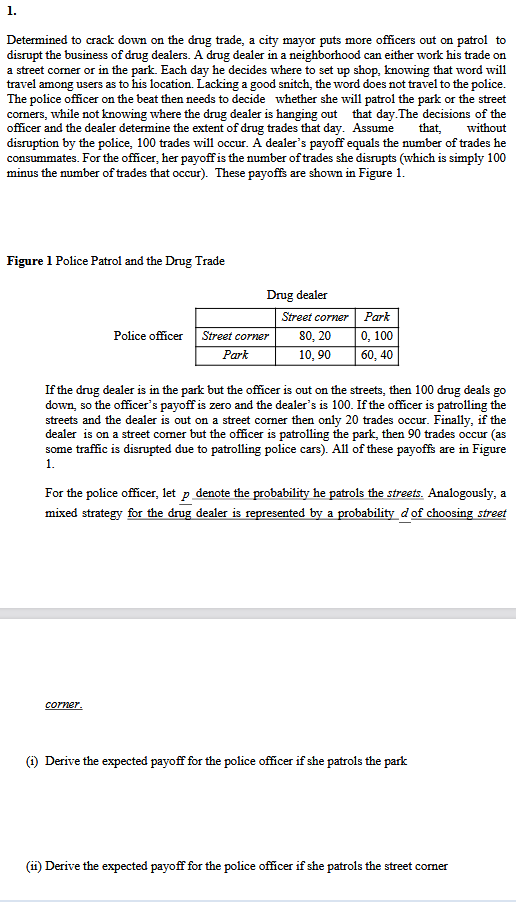
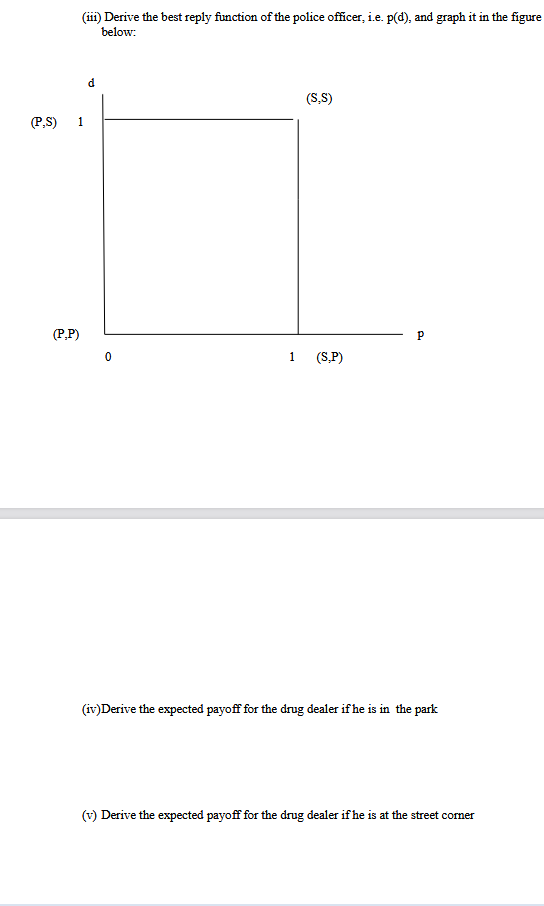
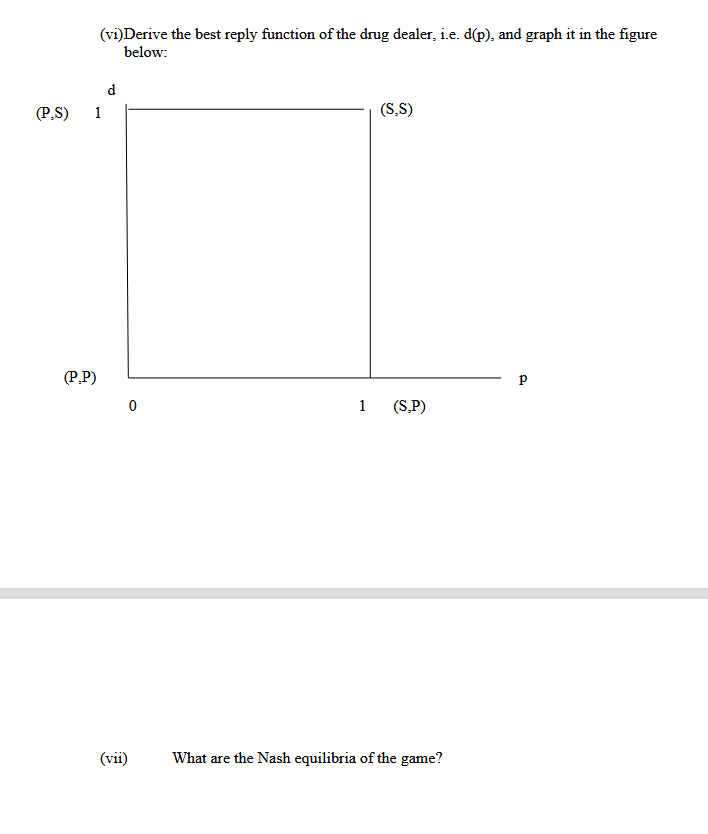
1. Determined to crack down on the drug trade, a city mayor puts more officers out on patrol to disrupt the business of drug dealers. A drug dealer in a neighborhood can either work his trade on a street corner or in the park. Each day he decides where to set up shop, knowing that word will travel among users as to his location. Lacking a good snitch, the word does not travel to the police. The police officer on the beat then needs to decide whether she will patrol the park or the street corners, while not knowing where the drug dealer is hanging out that day.The decisions of the officer and the dealer determine the extent of drug trades that day. Assume that, without disruption by the police, 100 trades will occur. A dealer's payoff equals the number of trades he consummates. For the officer, her payoff is the number of trades she disrupts (which is simply 100 minus the number of trades that occur). These payoffs are shown in Figure 1. Figure 1 Police Patrol and the Drug Trade Drug dealer Street corner Park Street corner 80,20 0.100 Park 10,90 60,40 Police officer If the drug dealer is in the park but the officer is out on the streets, then 100 drug deals go down, so the officer's payoff is zero and the dealer's is 100. If the officer is patrolling the streets and the dealer is out on a street corner then only 20 trades occur. Finally, if the dealer is on a street comer but the officer is patrolling the park, then 90 trades occur (as some traffic is disrupted due to patrolling police cars). All of these payoffs are in Figure 1. For the police officer, let p denote the probability he patrols the streets. Analogously, a mixed strategy for the drug dealer is represented by a probability d of choosing street corner. (1) Derive the expected payoff for the police officer if she patrols the park () Derive the expected payoff for the police officer if she patrols the street comer (11) Derive the best reply function of the police officer, i.e.pd), and graph it in the figure below: d (SS) (P.S) 1 (P.P) 0 1 (SP) (iv)Derive the expected payoff for the drug dealer if he is in the park (1) Derive the expected payoff for the drug dealer if he is at the street corner (vi)Derive the best reply function of the drug dealer, i.e. d(p), and graph it in the figure below: d P.S) 1 (SS) (P.P) 0 1 (SP) (vii) What are the Nash equilibria of the game? 1. Determined to crack down on the drug trade, a city mayor puts more officers out on patrol to disrupt the business of drug dealers. A drug dealer in a neighborhood can either work his trade on a street corner or in the park. Each day he decides where to set up shop, knowing that word will travel among users as to his location. Lacking a good snitch, the word does not travel to the police. The police officer on the beat then needs to decide whether she will patrol the park or the street corners, while not knowing where the drug dealer is hanging out that day.The decisions of the officer and the dealer determine the extent of drug trades that day. Assume that, without disruption by the police, 100 trades will occur. A dealer's payoff equals the number of trades he consummates. For the officer, her payoff is the number of trades she disrupts (which is simply 100 minus the number of trades that occur). These payoffs are shown in Figure 1. Figure 1 Police Patrol and the Drug Trade Drug dealer Street corner Park Street corner 80,20 0.100 Park 10,90 60,40 Police officer If the drug dealer is in the park but the officer is out on the streets, then 100 drug deals go down, so the officer's payoff is zero and the dealer's is 100. If the officer is patrolling the streets and the dealer is out on a street corner then only 20 trades occur. Finally, if the dealer is on a street comer but the officer is patrolling the park, then 90 trades occur (as some traffic is disrupted due to patrolling police cars). All of these payoffs are in Figure 1. For the police officer, let p denote the probability he patrols the streets. Analogously, a mixed strategy for the drug dealer is represented by a probability d of choosing street corner. (1) Derive the expected payoff for the police officer if she patrols the park () Derive the expected payoff for the police officer if she patrols the street comer (11) Derive the best reply function of the police officer, i.e.pd), and graph it in the figure below: d (SS) (P.S) 1 (P.P) 0 1 (SP) (iv)Derive the expected payoff for the drug dealer if he is in the park (1) Derive the expected payoff for the drug dealer if he is at the street corner (vi)Derive the best reply function of the drug dealer, i.e. d(p), and graph it in the figure below: d P.S) 1 (SS) (P.P) 0 1 (SP) (vii) What are the Nash equilibria of the game