1. EBV is considering an investment in Softco, an early-stage software company. If Softco can execute on its business plan, then EBV estimates it would be ve years until a successful exit, when Softco would have about $75M in revenue, a 20 percent operating margin, a tax rate of 40 percent, and approximately $75M in capital. Subsequent to a successful exit, EBV believes that Softco could enjoy seven more years of rapid growth. To make the transaction work, EBV believes that the exit value must be at least $400M. How does this compare with the reality-check DCF? How much must the baseline assumptions change to justify this valuation?
2. Softco, the company valued in Exercise 11.1, is expected to have the following business at exit: Softco provides business process integration software and services for corporations across a broad range of enterprise markets. Its main product is the Softco business process integration software platform together with packaged applications and content, where it expects to derive 75 percent of its revenue. In addition, the company expects to earn the remainder of its revenue from mainframe outsourcing and midrange systems management.
Use whatever resources you want to identify at least two comparable companies for Softco and to estimate a relative valuation



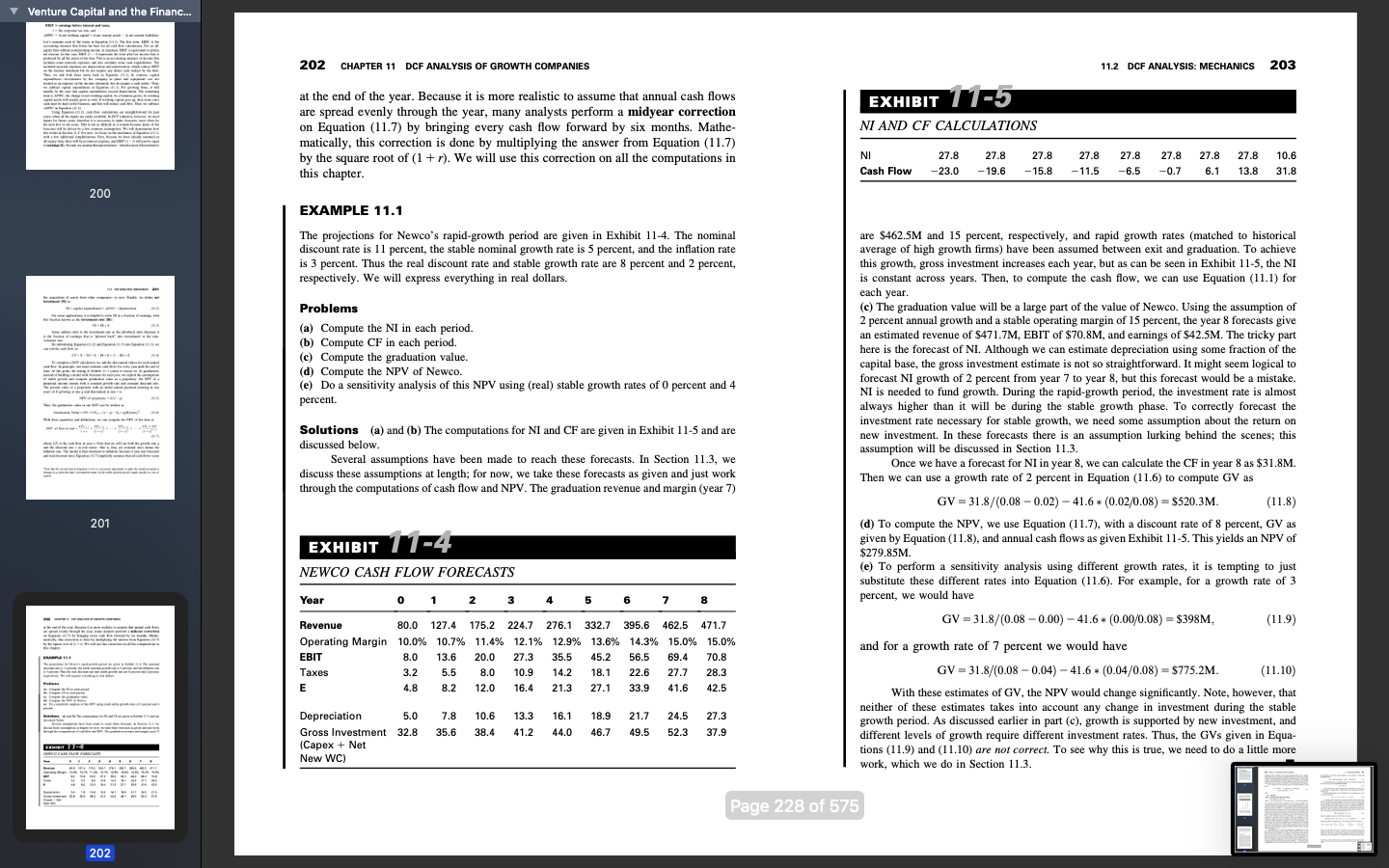
V Venture Capital and the Financ... 196 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.1 DCF ANALYSIS: CONCEPTS 197 books. Professor Damodaran's website, http://pages.stern.nyu.edu/adamodar/, is an excellent source for current data that can be used as inputs and comparisons for valuation problems. 194 11.1 DCF ANALYSIS: CONCEPTS figure out how long rapid growth will be sustained from that point. Although this may seem to be a daunting exercise, even a cursory treatment can provide useful insights into the determinants of long-run success for the business and can also help investors to better understand the dynamics of a company's industry. How can we estimate the typical length of a rapid-growth period? We can get some hints by looking at historical data. Exhibit 11-2 compares the revenue growth of newly listed public companies to that of their respective industries in the years following their IPOs. The key variable in Exhibit 11-2 is the industry-adjusted revenue growth rate. To obtain this rate, we start by computing the revenue growth rate for each industry. Then, for each firm, we subtract the appropriate industry rate from the firm's growth rate.' We compute the "years since IPO" as the total number of years since the company first appeared in the S&P database, a comprehensive source of all firms that filed financial statements with the SEC. The middle line of Exhibit 11-2 shows the median industry-adjusted growth rate, the top line gives the 75th percentile, and the bottom line gives the 25th percentile. In the first full year after their IPO, firms DCF ANALYSIS OF GROWTH COMPANIES VC-backed companies go through many stages of development. In earlier chapters, we focused on the stages corresponding to rounds of VC investment; all these stages effectively occur during the "childhood" of a company. We refer to this childhood as the venture period. For a successful VC-backed company, an IPO exit marks the beginning of its adolescence, with a rapid-growth period still to come. Usually, it is only after many more years that the company will reach maturity and settle down to a stable-growth period. Exhibit 11-1 gives a schematic example of these periods, with some appropriate milestones. One of these milestones, which we call graduation, marks the transition from the rapid-growth period to the stable- growth period. At time zero the initial VC investment-we need to estimate an exit value for the end of the venture period. The venture period is T years long, where T typically varies between three and seven years. The exit value will be based on forecasts for the rapid-growth and stable-growth periods. Thus, in estimating the exit value, we are imagining the company T years into the future and trying to EXHIBIT TEZ REVENUE GROWTH COMPARED TO INDUSTRY AVERAGES, PLOTTED AS A FUNCTION OF "YEARS SINCE IPO" 70.0% EXHIBIT 111 75th percentile - median 25th percentile 195 PHASES OF GROWTH 3 to 10 years long: ends when company enters period of stable growth and return on capital and operating margin approach industry averages Revenue Growth-Industry Average 3 to 7 years long: ends with IPO or acquisition In perpetuity return on new investment close to the cost of capital -20.0% Years since IPO exit graduation SOURCE: Wharton Research Data Systems (WRDS), S&P Compustat. Venture Period Rapid- Growth Period Stable- Growth Period Initial VC investment 'Industries are defined by the first three digits of the Standard Industrial Classification (SIC) code. These codes can be viewed at http://www.osha.gov/pls/imis/sic_manual.html. 196 V Venture Capital and the Financ... 198 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.2 DCF ANALYSIS: MECHANICS 199 To do this, we abstract from the actual capital structure and assume that the firm is all-equity financed. Indeed, the assumption of all-equity financing is very reason- able for VC-backed companies. Exhibit 11-3 shows the mean and median per- centage of debt in the capital structure of VC-backed companies in each of the 15 years subsequent to their IPOs. For each firm, we compute the enterprise value as the market value of equity plus the book value of debt and then compute the percentage of debt in this enterprise value: 196 grow much faster than their industry: in year 1, the median industry-adjusted growth rate is 14.7 percent, and the 75th percentile is 57.1 percent. By the fifth year after the IPO, however, this median is almost exactly 0; thus, as measured by revenue growth, we can say that the typical firm reaches "maturity" within five years after the IPO. Also, although some firms continue to grow faster than their industry average beyond year 5, the overall distribution of industry-adjusted growth rates is nearly symmetric around zero. This symmetry demonstrates that the five-year-old public firms are fairly representative of their industries. Revenue growth at the industry average is not the only signal that a company has entered a stable-growth period. A good analyst should also consider the company's return on capital (R) and operating margins. During the rapid-growth phase, we would expect a company to be earning R above the cost of capital (r), even if these returns are not expected to be realized until several years in the future. Furthermore, the rapid-growth phase is often characterized by operating margins lower than industry averages, as companies scale up their production and price aggressively to gain market share. In the stable-growth phase, both R and operating margins should settle down to industry averages. EXHIBIT 1173 LEVERAGE OF VC-BACKED FIRMS Years Since IPO Mean Median 11.2 DCF ANALYSIS: MECHANICS 4.7% 4.0% 5.7% 6.8% 7.2% 8.1% 8.2% 11.1% 8.7% 10.6% 11.0% 11.8% 12.4% 11.0% 7.7% 11.0% 1.2% 1.9% 2.8% 3.8% 3.9% 4.4% 5.1% 6.0% 5.6% 6.2% 6.0% 6.4% 8.9% 7.8% 4.8% 6.4% 197 SOURCE: Michael Roberts, Wharton. DCF analysis is the gold standard of valuation. If done properly with accurate inputs a big "if"-a DCF model will produce the "correct" valuation of a firm. For this reason, most investment bankers, financial analysts, and academics make DCF analysis a centerpiece of their valuation work. Although there are many different types of DCF models, the simple capital structure of most VC portfolio companies renders moot many of these differences, so we will be able to con- centrate on the key concepts common to all types. All DCF models have two key inputs: Discount rates (the "D" part) and cash flows (the "CF" part). Discount rates for venture capital were discussed at length in Chapter 4. In this chapter, we need discount rates for public companies, so the cost of venture capital is not directly applicable. There are several options for estimating discount rates for public companies. The simplest option used in this chapter-is to just use the average cost of capital for the company's industry. The Industry Statistics worksheet of the DCF.xls spreadsheet provides this data for 100 different industries. A more complex alternative is to use a smaller group of comparable companies. This alternative will be discussed in Chapter 12. We focus most of this section on the computation of cash flow. The concept of cash flow is designed to pierce the accounting veil used for financial reporting and taxes so that we are left with the cash that is actually generated by the business. Also, we want to compute the cash flows generated by all the assets of the firm, irrespective of the types of claims (equity, debt, preferred stock), on those assets. We see from these data that even 15 years after their IPOs, VC-backed firms still have only a mean debt percentage of 11.0 and a median percentage of 6.4. During the rapid-growth phase in the first few years after the IPOs of these firms, their percentages are even lower. Thus, we conclude that the simplifying assumption of all-equity financing is close to the truth for the vast majority of VC-backed firms. Throughout our analysis, we analyze only operating assets, income, and expenses. Nonoperating assets would include excess cash, marketable securities, or Page 224 of 575 198 Venture Capital and the Financ... 200 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.2 DCF ANALYSIS: MECHANICS 201 the acquisition of assets from other companies is zero. Finally, we define net investment (NI) as NI = capital expenditures + ANWC - depreciation. (11.2) anything else that is unrelated to the revenue-producing business of the company. Nonoperating assets can comprise a significant fraction of the asset base of mature companies, and disentangling operating from nonoperating assets can require deep analysis of accounting statements. In this instance, we are fortunate to be analyzing companies with short histories, so both the capital structure and asset base are rela- tively simple, and we can focus our attention on the operating side of the business. For a company with only operating assets, the standard definition of cash flow is CF = EBIT(1 t) + depreciation + amortization (11.1) - capital expenditures - NWC where 198 For some applications, it is helpful to write NI as a fraction of earnings, with this fraction known as the investment rate (IR): NI = IR * E. (11.3) Some authors refer to the investment rate as the plowback ratio (because it is the fraction of earnings that is "plowed back" into investment) or the rein- vestment rate. By substituting Equation (11.2) and Equation (11.3) into Equation (11.1), we can rewrite cash flow as CF = E - NI = E-IR *E= (1 - IR) * E. (11.4) To complete a DCF calculation, we add the discounted values for each annual cash flow. In principle, one must estimate cash flows for every year until the end of time. At this point, the timing of Exhibit 11-1 comes to rescue us. At graduation, instead of building a model with forecasts for each year, we exploit the assumptions of stable growth and compute graduation value as a perpetuity: the NPV of a perpetual income stream with a constant growth rate and constant discount rate. The present value of a perpetuity with an initial annual payment starting in one year) of X growing at rate g and discounted at rater is NPV of perpetuity = x/r-g). (11.5) Thus, the graduation value in our DCF can be written as 199 CF = cash flow, EBIT = earnings before interest and taxes, t = the corporate tax rate, and ANWC = A net working capital = A net current assets - A net current liabilities. Let's examine each of the terms in Equation (11.1). The first term, EBIT, is the accounting measure that forms the base for all cash flow calculations. For an all- equity firm without nonoperating income or expenses, EBIT is equivalent to pretax net income. In this case, EBIT (1 t) represents the total after-tax income that is produced by all the assets of the firm. This is an accounting measure of income that includes some noncash expenses and also excludes some cash expenditures. The included noncash expenses are depreciation and amortization, which reduce EBIT on the income statement but do not require any direct cash outlays by the firm. Thus, we add both these items back in Equation (11.1). In contrast, capital expenditures investments by the company in plant and equipment are not treated as an expense on the income statement, but do require a cash outlay. Thus, we subtract capital expenditures in Equation (11.1). For growing firms, it will usually be the case that capital expenditures exceed depreciation. The remaining item is ANWC, the change in net working capital. As a business grows, its working capital needs will usually grow as well. If working capital goes up, then some extra cash must be kept in the business, and this will reduce cash flow. Thus, we subtract ANWC in Equation (11.1). Using Equation (11.1), cash flow calculations are straightforward for past years, when all the inputs are easily available. In DCF valuation, however, we need inputs for future years, therefore it is necessary to make forecasts, most often for the next five or ten years. This is not as difficult as it sounds because many of the forecasts will be driven by a few common assumptions. We will demonstrate how this works in Section 11.3. For now, we focus on the mechanics of Equation (11.1), with a few additional simplifications. First, because we have already assumed an all-equity firm, there will be no interest expense, and EBIT (1 - 1) will just be equal to earnings (E). Second, we assume that amortization which is most often related to Graduation Value = GV = CFs+1/(r-g) - E* (g/R(new))? (11.6) With these quantities and definitions, we can compute the NPV of the firm as NPV of firm at exit = CFT+1 + CFT+2 + ... + CFT+ + ... + CFs + GV 1+ (1+r) (1 + r)" (1 + r)S- (11.7) where CF, is the cash flow in year n. Note that we will use both the growth rate g and the discount rater in real termsthat is, they are nominal rates minus the inflation rate. The model is thus invariant to inflation, because it uses real forecasts and real discount rates. Equation (11.7) implicitly assumes that all cash flows occur "Note that the second term in Equation (11.6) is a necessary adjustment to make the model invariant to changes in g when the firm's investment return (in the stable growth period) equals exactly its cost of capital. Page 226 of 575 200 V Venture Capital and the Financ... 202 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.2 DCF ANALYSIS: MECHANICS 203 at the end of the year. Because it is more realistic to assume that annual cash flows are spread evenly through the year, many analysts perform a midyear correction on Equation (11.7) by bringing every cash flow forward by six months. Mathe- matically, this correction is done by multiplying the answer from Equation (11.7) by the square root of (1 + r). We will use this correction on all the computations in this chapter. EXHIBIT 1 -5 NI AND CF CALCULATIONS Cash Flow 27.8 -23.0 27.8 -19.6 27.8 -15.8 27.8 -11.5 27.8 -6.5 27.8 -0.7 27.8 6.1 27.8 13.8 10.6 31.8 200 EXAMPLE 11.1 The projections for Newco's rapid-growth period are given in Exhibit 11-4. The nominal discount rate is 11 percent, the stable nominal growth rate is 5 percent, and the inflation rate is 3 percent. Thus the real discount rate and stable growth rate are 8 percent and 2 percent, respectively. We will express everything in real dollars. Problems (a) Compute the NI in each period. (b) Compute CF in each period. (c) Compute the graduation value. (d) Compute the NPV of Newco. (e) Do a sensitivity analysis of this NPV using (real) stable growth rates of 0 percent and 4 percent. Solutions (a) and (b) The computations for NI and CF are given in Exhibit 11-5 and are discussed below. Several assumptions have been made to reach these forecasts. In Section 11.3, we discuss these assumptions at length; for now, we take these forecasts as given and just work through the computations of cash flow and NPV. The graduation revenue and margin (year 7) are $462.5M and 15 percent, respectively, and rapid growth rates (matched to historical age of high growth firms) have been assumed between exit and graduation. To achieve this growth, gross investment increases each year, but as can be seen in Exhibit 11-5, the NI is constant across years. Then, to compute the cash flow, we can use Equation (11.1) for each year. (c) The graduation value will be a large part of the value of Newco. Using the assumption of 2 percent annual growth and a stable operating margin of 15 percent, the year 8 forecasts give an estimated revenue of $471.7M, EBIT of $70.8M, and earnings of $42.5M. The tricky part here is the forecast of NI. Although we can estimate depreciation using some fraction of the capital base, the gross investment estimate is not so straightforward. It might seem logical to forecast NI growth of 2 percent from year 7 to year 8, but this forecast would be a mistake. NI is needed to fund growth. During the rapid-growth period, the investment rate is almost always higher than it will be during the stable growth phase. To correctly forecast the investment rate necessary for stable growth, we need some assumption about the return on new investment. In these forecasts there is an assumption lurking behind the scenes; this assumption will be discussed in Section 11.3. Once we have a forecast for NI in year 8, we can calculate the CF in year 8 as $31.81 Then we can use a growth rate of 2 percent in Equation (11.6) to compute GV as GV = 31.8/(0.08 -0.02) - 41.6* (0.02/0.08) = $520.3M. (11.8) (d) To compute the NPV, we use Equation (11.7), with a discount rate of 8 percent, GV as given by Equation (11.8), and annual cash flows as given Exhibit 11-5. This yields an NPV of $279.85M. (e) To perform a sensitivity analysis using different growth rates, it is tempting to just substitute these different rates into Equation (11.6). For example, for a growth rate of 3 percent, we would have GV = 31.8/(0.08 -0.00) - 41.6 (0.00/0.08) = $398M, (11.9) 201 EXHIBIT 11-4 NEWCO CASH FLOW FORECASTS 0 1 2 3 Year 4 5 6 7 8 Revenue Operating Margin EBIT Taxes 80.0 10.0% 8.0 3.2 4.8 127.4 10.7% 13.6 5.5 8.2 175.2 11.4% 20.0 8.0 12.0 224.7 12.1% 27.3 10.9 16.4 276.1 332.7 12.9% 13.6% 35.5 45.2 14.2 18.1 21.3 27.1 395.6 14.3% 56.5 22.6 33.9 462.5 15.0% 69.4 27.7 41.6 471.7 15.0% 70.8 28.3 42.5 and for a growth rate of 7 percent we would have GV = 31.8/(0.08 -0.04) - 41.6 (0.04/0.08) = $775.2M. (11.10) With these estimates of GV, the NPV would change significantly. Note, however, that neither of these estimates takes into account any change in investment during the stable growth period. As discussed earlier in part (c), growth is supported by new investment, and different levels of growth require different investment rates. Thus, the GVs given in Equa- tions (11.9) and (11.10) are not correct. To see why this is true, we need to do a little more work, which we do in Section 11.3. 24.5 27.3 Depreciation 5.0 Gross Investment 32.8 (Capex + Net New WC) 7.8 35.6 10.6 38.4 13.3 41.2 16.1 44.0 18.9 46.7 21.7 49.5 52.3 Page 228 of 575 202 V Venture Capital and the Financ... 196 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.1 DCF ANALYSIS: CONCEPTS 197 books. Professor Damodaran's website, http://pages.stern.nyu.edu/adamodar/, is an excellent source for current data that can be used as inputs and comparisons for valuation problems. 194 11.1 DCF ANALYSIS: CONCEPTS figure out how long rapid growth will be sustained from that point. Although this may seem to be a daunting exercise, even a cursory treatment can provide useful insights into the determinants of long-run success for the business and can also help investors to better understand the dynamics of a company's industry. How can we estimate the typical length of a rapid-growth period? We can get some hints by looking at historical data. Exhibit 11-2 compares the revenue growth of newly listed public companies to that of their respective industries in the years following their IPOs. The key variable in Exhibit 11-2 is the industry-adjusted revenue growth rate. To obtain this rate, we start by computing the revenue growth rate for each industry. Then, for each firm, we subtract the appropriate industry rate from the firm's growth rate.' We compute the "years since IPO" as the total number of years since the company first appeared in the S&P database, a comprehensive source of all firms that filed financial statements with the SEC. The middle line of Exhibit 11-2 shows the median industry-adjusted growth rate, the top line gives the 75th percentile, and the bottom line gives the 25th percentile. In the first full year after their IPO, firms DCF ANALYSIS OF GROWTH COMPANIES VC-backed companies go through many stages of development. In earlier chapters, we focused on the stages corresponding to rounds of VC investment; all these stages effectively occur during the "childhood" of a company. We refer to this childhood as the venture period. For a successful VC-backed company, an IPO exit marks the beginning of its adolescence, with a rapid-growth period still to come. Usually, it is only after many more years that the company will reach maturity and settle down to a stable-growth period. Exhibit 11-1 gives a schematic example of these periods, with some appropriate milestones. One of these milestones, which we call graduation, marks the transition from the rapid-growth period to the stable- growth period. At time zero the initial VC investment-we need to estimate an exit value for the end of the venture period. The venture period is T years long, where T typically varies between three and seven years. The exit value will be based on forecasts for the rapid-growth and stable-growth periods. Thus, in estimating the exit value, we are imagining the company T years into the future and trying to EXHIBIT TEZ REVENUE GROWTH COMPARED TO INDUSTRY AVERAGES, PLOTTED AS A FUNCTION OF "YEARS SINCE IPO" 70.0% EXHIBIT 111 75th percentile - median 25th percentile 195 PHASES OF GROWTH 3 to 10 years long: ends when company enters period of stable growth and return on capital and operating margin approach industry averages Revenue Growth-Industry Average 3 to 7 years long: ends with IPO or acquisition In perpetuity return on new investment close to the cost of capital -20.0% Years since IPO exit graduation SOURCE: Wharton Research Data Systems (WRDS), S&P Compustat. Venture Period Rapid- Growth Period Stable- Growth Period Initial VC investment 'Industries are defined by the first three digits of the Standard Industrial Classification (SIC) code. These codes can be viewed at http://www.osha.gov/pls/imis/sic_manual.html. 196 V Venture Capital and the Financ... 198 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.2 DCF ANALYSIS: MECHANICS 199 To do this, we abstract from the actual capital structure and assume that the firm is all-equity financed. Indeed, the assumption of all-equity financing is very reason- able for VC-backed companies. Exhibit 11-3 shows the mean and median per- centage of debt in the capital structure of VC-backed companies in each of the 15 years subsequent to their IPOs. For each firm, we compute the enterprise value as the market value of equity plus the book value of debt and then compute the percentage of debt in this enterprise value: 196 grow much faster than their industry: in year 1, the median industry-adjusted growth rate is 14.7 percent, and the 75th percentile is 57.1 percent. By the fifth year after the IPO, however, this median is almost exactly 0; thus, as measured by revenue growth, we can say that the typical firm reaches "maturity" within five years after the IPO. Also, although some firms continue to grow faster than their industry average beyond year 5, the overall distribution of industry-adjusted growth rates is nearly symmetric around zero. This symmetry demonstrates that the five-year-old public firms are fairly representative of their industries. Revenue growth at the industry average is not the only signal that a company has entered a stable-growth period. A good analyst should also consider the company's return on capital (R) and operating margins. During the rapid-growth phase, we would expect a company to be earning R above the cost of capital (r), even if these returns are not expected to be realized until several years in the future. Furthermore, the rapid-growth phase is often characterized by operating margins lower than industry averages, as companies scale up their production and price aggressively to gain market share. In the stable-growth phase, both R and operating margins should settle down to industry averages. EXHIBIT 1173 LEVERAGE OF VC-BACKED FIRMS Years Since IPO Mean Median 11.2 DCF ANALYSIS: MECHANICS 4.7% 4.0% 5.7% 6.8% 7.2% 8.1% 8.2% 11.1% 8.7% 10.6% 11.0% 11.8% 12.4% 11.0% 7.7% 11.0% 1.2% 1.9% 2.8% 3.8% 3.9% 4.4% 5.1% 6.0% 5.6% 6.2% 6.0% 6.4% 8.9% 7.8% 4.8% 6.4% 197 SOURCE: Michael Roberts, Wharton. DCF analysis is the gold standard of valuation. If done properly with accurate inputs a big "if"-a DCF model will produce the "correct" valuation of a firm. For this reason, most investment bankers, financial analysts, and academics make DCF analysis a centerpiece of their valuation work. Although there are many different types of DCF models, the simple capital structure of most VC portfolio companies renders moot many of these differences, so we will be able to con- centrate on the key concepts common to all types. All DCF models have two key inputs: Discount rates (the "D" part) and cash flows (the "CF" part). Discount rates for venture capital were discussed at length in Chapter 4. In this chapter, we need discount rates for public companies, so the cost of venture capital is not directly applicable. There are several options for estimating discount rates for public companies. The simplest option used in this chapter-is to just use the average cost of capital for the company's industry. The Industry Statistics worksheet of the DCF.xls spreadsheet provides this data for 100 different industries. A more complex alternative is to use a smaller group of comparable companies. This alternative will be discussed in Chapter 12. We focus most of this section on the computation of cash flow. The concept of cash flow is designed to pierce the accounting veil used for financial reporting and taxes so that we are left with the cash that is actually generated by the business. Also, we want to compute the cash flows generated by all the assets of the firm, irrespective of the types of claims (equity, debt, preferred stock), on those assets. We see from these data that even 15 years after their IPOs, VC-backed firms still have only a mean debt percentage of 11.0 and a median percentage of 6.4. During the rapid-growth phase in the first few years after the IPOs of these firms, their percentages are even lower. Thus, we conclude that the simplifying assumption of all-equity financing is close to the truth for the vast majority of VC-backed firms. Throughout our analysis, we analyze only operating assets, income, and expenses. Nonoperating assets would include excess cash, marketable securities, or Page 224 of 575 198 Venture Capital and the Financ... 200 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.2 DCF ANALYSIS: MECHANICS 201 the acquisition of assets from other companies is zero. Finally, we define net investment (NI) as NI = capital expenditures + ANWC - depreciation. (11.2) anything else that is unrelated to the revenue-producing business of the company. Nonoperating assets can comprise a significant fraction of the asset base of mature companies, and disentangling operating from nonoperating assets can require deep analysis of accounting statements. In this instance, we are fortunate to be analyzing companies with short histories, so both the capital structure and asset base are rela- tively simple, and we can focus our attention on the operating side of the business. For a company with only operating assets, the standard definition of cash flow is CF = EBIT(1 t) + depreciation + amortization (11.1) - capital expenditures - NWC where 198 For some applications, it is helpful to write NI as a fraction of earnings, with this fraction known as the investment rate (IR): NI = IR * E. (11.3) Some authors refer to the investment rate as the plowback ratio (because it is the fraction of earnings that is "plowed back" into investment) or the rein- vestment rate. By substituting Equation (11.2) and Equation (11.3) into Equation (11.1), we can rewrite cash flow as CF = E - NI = E-IR *E= (1 - IR) * E. (11.4) To complete a DCF calculation, we add the discounted values for each annual cash flow. In principle, one must estimate cash flows for every year until the end of time. At this point, the timing of Exhibit 11-1 comes to rescue us. At graduation, instead of building a model with forecasts for each year, we exploit the assumptions of stable growth and compute graduation value as a perpetuity: the NPV of a perpetual income stream with a constant growth rate and constant discount rate. The present value of a perpetuity with an initial annual payment starting in one year) of X growing at rate g and discounted at rater is NPV of perpetuity = x/r-g). (11.5) Thus, the graduation value in our DCF can be written as 199 CF = cash flow, EBIT = earnings before interest and taxes, t = the corporate tax rate, and ANWC = A net working capital = A net current assets - A net current liabilities. Let's examine each of the terms in Equation (11.1). The first term, EBIT, is the accounting measure that forms the base for all cash flow calculations. For an all- equity firm without nonoperating income or expenses, EBIT is equivalent to pretax net income. In this case, EBIT (1 t) represents the total after-tax income that is produced by all the assets of the firm. This is an accounting measure of income that includes some noncash expenses and also excludes some cash expenditures. The included noncash expenses are depreciation and amortization, which reduce EBIT on the income statement but do not require any direct cash outlays by the firm. Thus, we add both these items back in Equation (11.1). In contrast, capital expenditures investments by the company in plant and equipment are not treated as an expense on the income statement, but do require a cash outlay. Thus, we subtract capital expenditures in Equation (11.1). For growing firms, it will usually be the case that capital expenditures exceed depreciation. The remaining item is ANWC, the change in net working capital. As a business grows, its working capital needs will usually grow as well. If working capital goes up, then some extra cash must be kept in the business, and this will reduce cash flow. Thus, we subtract ANWC in Equation (11.1). Using Equation (11.1), cash flow calculations are straightforward for past years, when all the inputs are easily available. In DCF valuation, however, we need inputs for future years, therefore it is necessary to make forecasts, most often for the next five or ten years. This is not as difficult as it sounds because many of the forecasts will be driven by a few common assumptions. We will demonstrate how this works in Section 11.3. For now, we focus on the mechanics of Equation (11.1), with a few additional simplifications. First, because we have already assumed an all-equity firm, there will be no interest expense, and EBIT (1 - 1) will just be equal to earnings (E). Second, we assume that amortization which is most often related to Graduation Value = GV = CFs+1/(r-g) - E* (g/R(new))? (11.6) With these quantities and definitions, we can compute the NPV of the firm as NPV of firm at exit = CFT+1 + CFT+2 + ... + CFT+ + ... + CFs + GV 1+ (1+r) (1 + r)" (1 + r)S- (11.7) where CF, is the cash flow in year n. Note that we will use both the growth rate g and the discount rater in real termsthat is, they are nominal rates minus the inflation rate. The model is thus invariant to inflation, because it uses real forecasts and real discount rates. Equation (11.7) implicitly assumes that all cash flows occur "Note that the second term in Equation (11.6) is a necessary adjustment to make the model invariant to changes in g when the firm's investment return (in the stable growth period) equals exactly its cost of capital. Page 226 of 575 200 V Venture Capital and the Financ... 202 CHAPTER 11 DCF ANALYSIS OF GROWTH COMPANIES 11.2 DCF ANALYSIS: MECHANICS 203 at the end of the year. Because it is more realistic to assume that annual cash flows are spread evenly through the year, many analysts perform a midyear correction on Equation (11.7) by bringing every cash flow forward by six months. Mathe- matically, this correction is done by multiplying the answer from Equation (11.7) by the square root of (1 + r). We will use this correction on all the computations in this chapter. EXHIBIT 1 -5 NI AND CF CALCULATIONS Cash Flow 27.8 -23.0 27.8 -19.6 27.8 -15.8 27.8 -11.5 27.8 -6.5 27.8 -0.7 27.8 6.1 27.8 13.8 10.6 31.8 200 EXAMPLE 11.1 The projections for Newco's rapid-growth period are given in Exhibit 11-4. The nominal discount rate is 11 percent, the stable nominal growth rate is 5 percent, and the inflation rate is 3 percent. Thus the real discount rate and stable growth rate are 8 percent and 2 percent, respectively. We will express everything in real dollars. Problems (a) Compute the NI in each period. (b) Compute CF in each period. (c) Compute the graduation value. (d) Compute the NPV of Newco. (e) Do a sensitivity analysis of this NPV using (real) stable growth rates of 0 percent and 4 percent. Solutions (a) and (b) The computations for NI and CF are given in Exhibit 11-5 and are discussed below. Several assumptions have been made to reach these forecasts. In Section 11.3, we discuss these assumptions at length; for now, we take these forecasts as given and just work through the computations of cash flow and NPV. The graduation revenue and margin (year 7) are $462.5M and 15 percent, respectively, and rapid growth rates (matched to historical age of high growth firms) have been assumed between exit and graduation. To achieve this growth, gross investment increases each year, but as can be seen in Exhibit 11-5, the NI is constant across years. Then, to compute the cash flow, we can use Equation (11.1) for each year. (c) The graduation value will be a large part of the value of Newco. Using the assumption of 2 percent annual growth and a stable operating margin of 15 percent, the year 8 forecasts give an estimated revenue of $471.7M, EBIT of $70.8M, and earnings of $42.5M. The tricky part here is the forecast of NI. Although we can estimate depreciation using some fraction of the capital base, the gross investment estimate is not so straightforward. It might seem logical to forecast NI growth of 2 percent from year 7 to year 8, but this forecast would be a mistake. NI is needed to fund growth. During the rapid-growth period, the investment rate is almost always higher than it will be during the stable growth phase. To correctly forecast the investment rate necessary for stable growth, we need some assumption about the return on new investment. In these forecasts there is an assumption lurking behind the scenes; this assumption will be discussed in Section 11.3. Once we have a forecast for NI in year 8, we can calculate the CF in year 8 as $31.81 Then we can use a growth rate of 2 percent in Equation (11.6) to compute GV as GV = 31.8/(0.08 -0.02) - 41.6* (0.02/0.08) = $520.3M. (11.8) (d) To compute the NPV, we use Equation (11.7), with a discount rate of 8 percent, GV as given by Equation (11.8), and annual cash flows as given Exhibit 11-5. This yields an NPV of $279.85M. (e) To perform a sensitivity analysis using different growth rates, it is tempting to just substitute these different rates into Equation (11.6). For example, for a growth rate of 3 percent, we would have GV = 31.8/(0.08 -0.00) - 41.6 (0.00/0.08) = $398M, (11.9) 201 EXHIBIT 11-4 NEWCO CASH FLOW FORECASTS 0 1 2 3 Year 4 5 6 7 8 Revenue Operating Margin EBIT Taxes 80.0 10.0% 8.0 3.2 4.8 127.4 10.7% 13.6 5.5 8.2 175.2 11.4% 20.0 8.0 12.0 224.7 12.1% 27.3 10.9 16.4 276.1 332.7 12.9% 13.6% 35.5 45.2 14.2 18.1 21.3 27.1 395.6 14.3% 56.5 22.6 33.9 462.5 15.0% 69.4 27.7 41.6 471.7 15.0% 70.8 28.3 42.5 and for a growth rate of 7 percent we would have GV = 31.8/(0.08 -0.04) - 41.6 (0.04/0.08) = $775.2M. (11.10) With these estimates of GV, the NPV would change significantly. Note, however, that neither of these estimates takes into account any change in investment during the stable growth period. As discussed earlier in part (c), growth is supported by new investment, and different levels of growth require different investment rates. Thus, the GVs given in Equa- tions (11.9) and (11.10) are not correct. To see why this is true, we need to do a little more work, which we do in Section 11.3. 24.5 27.3 Depreciation 5.0 Gross Investment 32.8 (Capex + Net New WC) 7.8 35.6 10.6 38.4 13.3 41.2 16.1 44.0 18.9 46.7 21.7 49.5 52.3 Page 228 of 575 202










