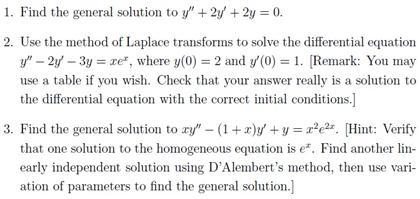
1. Find the general solution to y" + 2y + 2y = 0. 2. Use the method of Laplace transforms to solve the differential equation y" - 2y - 3y = xe, where y(0) = 2 and y'(0) = 1. [Remark: You may use a table if you wish. Check that your answer really is a solution to the differential equation with the correct initial conditions.] 3. Find the general solution to ry" - (1+x)y' + y = xe. [Hint: Verify that one solution to the homogeneous equation is e. Find another lin- early independent solution using D'Alembert's method, then use vari- ation of parameters to find the general solution.]