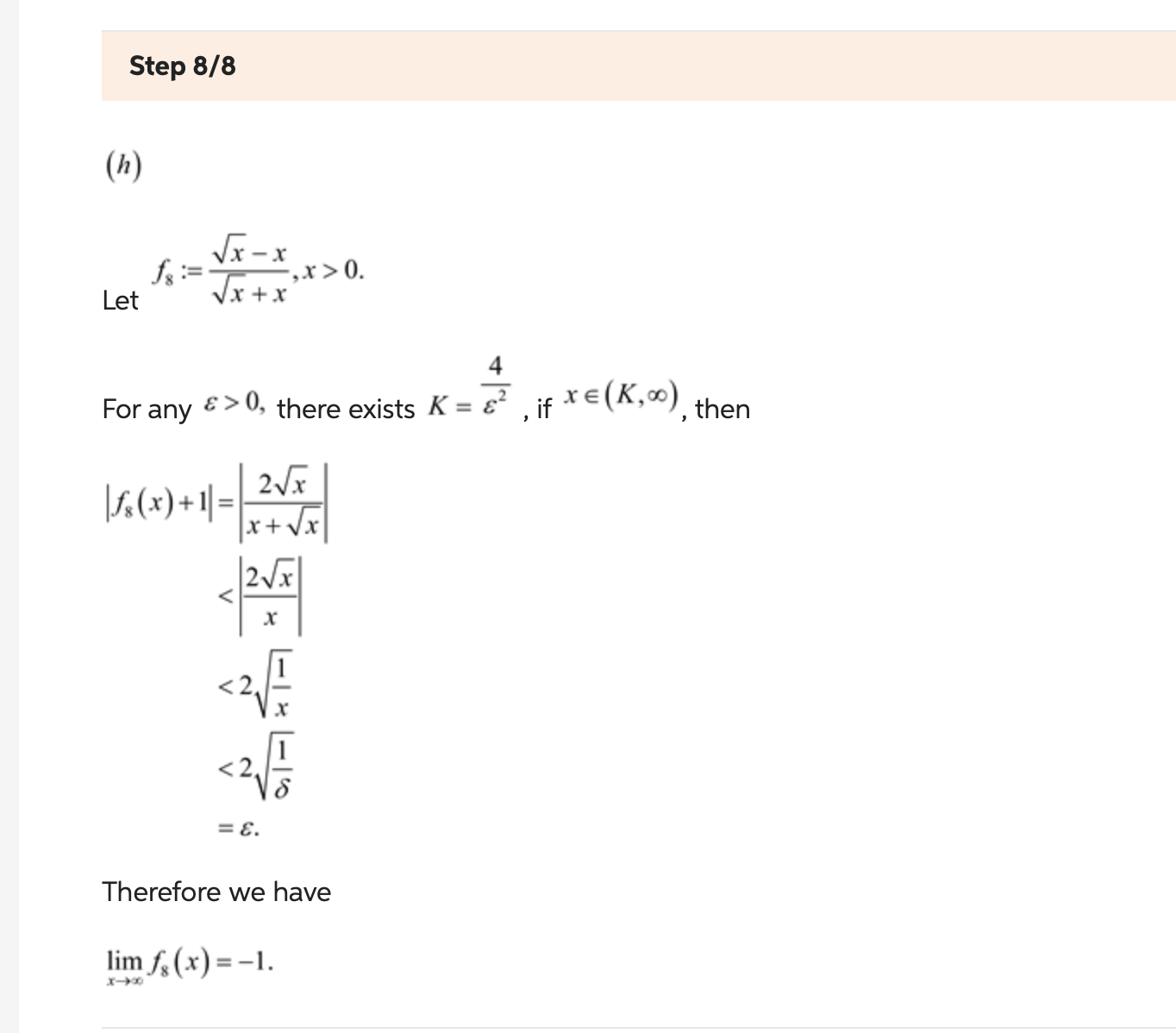
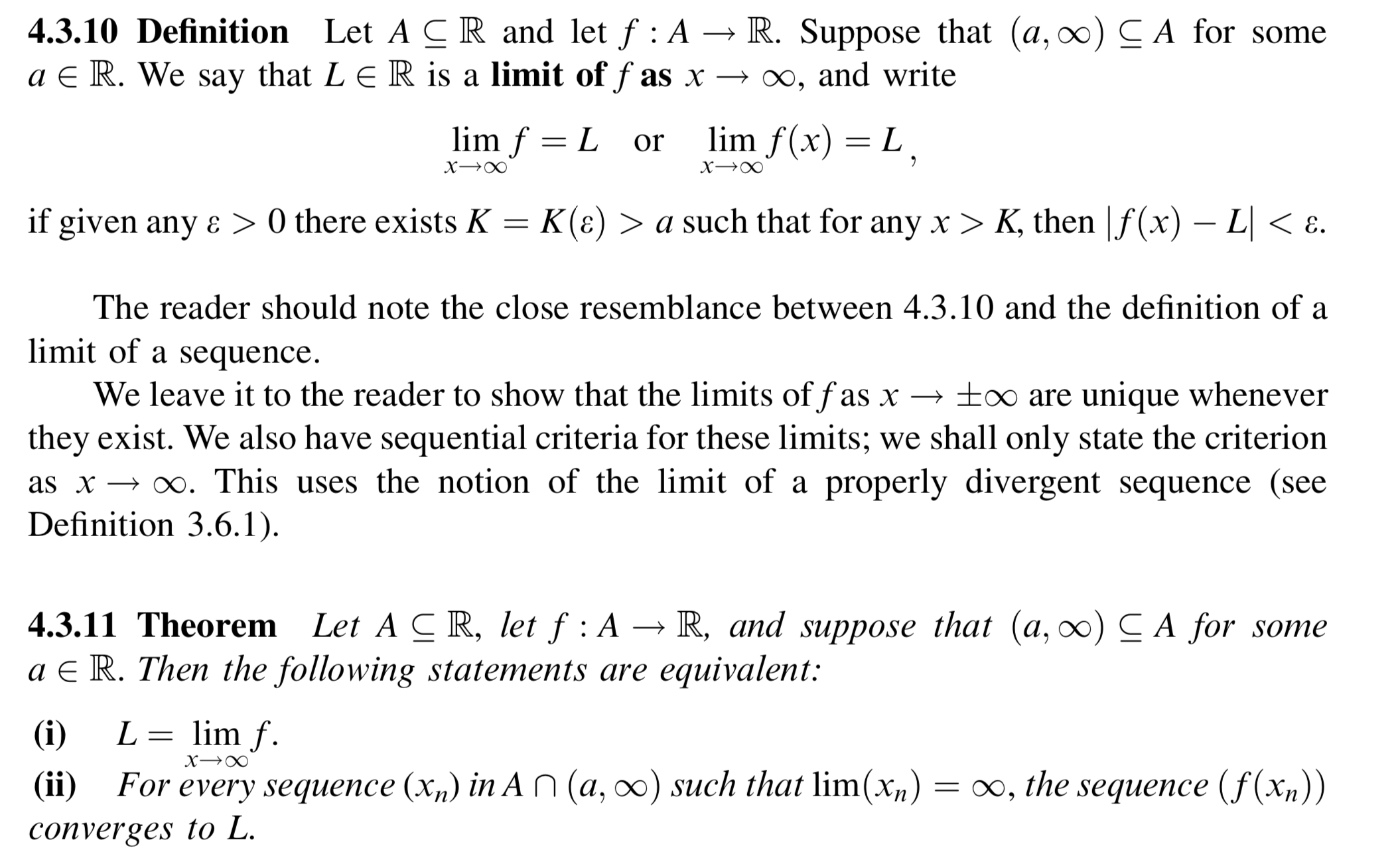
1. For the following limits, either a) evaluate them and rigorously show their convergence using any of the methods we discussed or b) rigorously show they do not exist: x3. 3+1 where A = (0,). x i) lim x ii) lim -xx1/3+x x1/3-x where A = (-, 0). (h) Step 8/8 x-x fs= ,x>0. Let x+x E For any &> 0, there exists K = &, if x = (K,), ther |fs(x)+1|= 2x s(x) + 1 = x + x a such that for any x > K, then |(x) L| < . The reader should note the close resemblance between 4.3.10 and the definition of a limit of a sequence. We leave it to the reader to show that the limits of fas x oo are unique whenever they exist. We also have sequential criteria for these limits; we shall only state the criterion as x . This uses the notion of the limit of a properly divergent sequence (see Definition 3.6.1). 4.3.11 Theorem Let A CR, let f : A >> R, and suppose that (a,) CA for some a = R. Then the following statements are equivalent: (i) L = lim f. X-X For every sequence (x) in An (a, ) such that lim(xn) = , the sequence (f(xn)) (ii) converges to L.