Question: 1. Given the differential equation y' = y 3y + 2 (a) Find all equilibrium points, and sketch the phase line. (b) Classify the

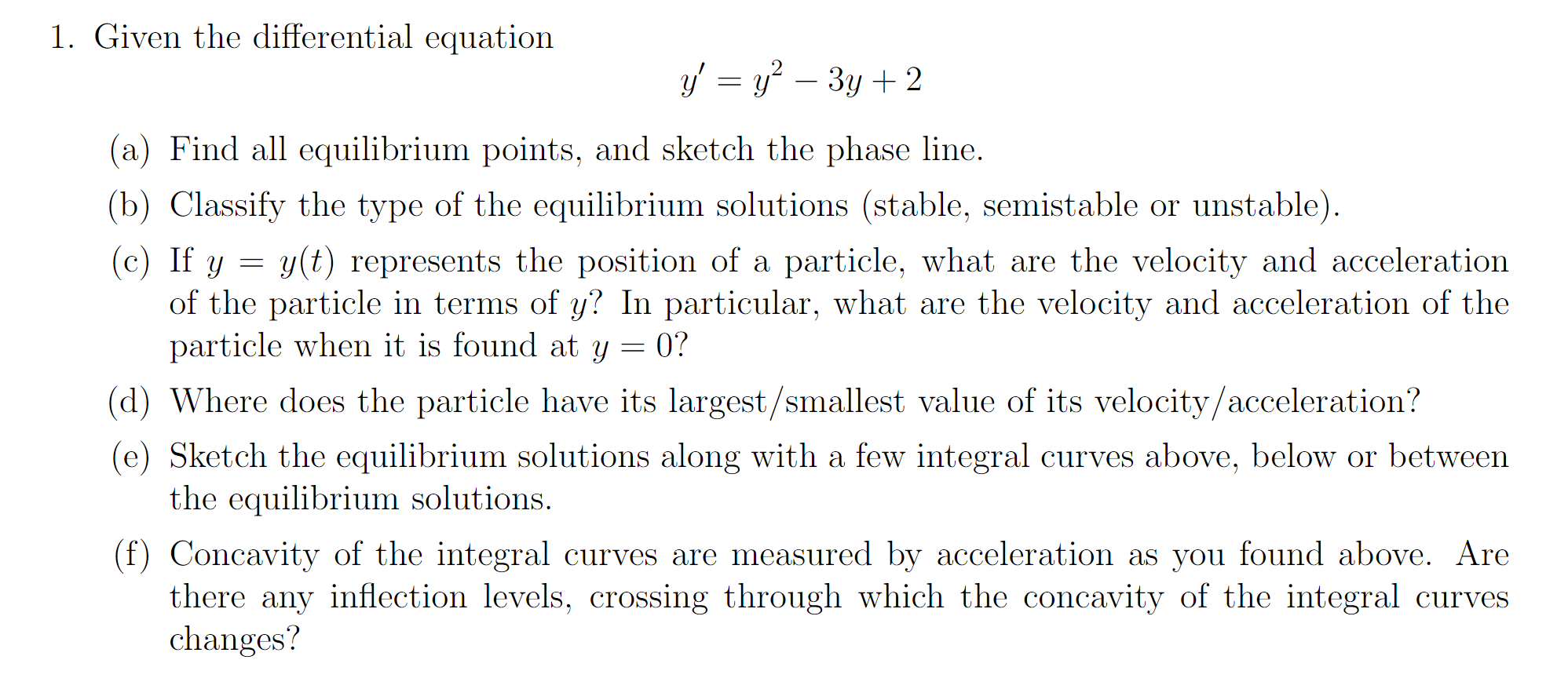
1. Given the differential equation y' = y 3y + 2 (a) Find all equilibrium points, and sketch the phase line. (b) Classify the type of the equilibrium solutions (stable, semistable or unstable). = (c) If y y(t) represents the position of a particle, what are the velocity and acceleration of the particle in terms of y? In particular, what are the velocity and acceleration of the particle when it is found at y = 0? (d) Where does the particle have its largest/smallest value of its velocity/acceleration? (e) Sketch the equilibrium solutions along with a few integral curves above, below or between the equilibrium solutions. (f) Concavity of the integral curves are measured by acceleration as you found above. Are there any inflection levels, crossing through which the concavity of the integral curves changes?

Step by Step Solution

★★★★★

3.49 Rating (152 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

A white college receptionist is fired when it is found that she told a black college applicant that the applications for admissions are distinguished by race by the notation of a small RH in the...

A direction field for the differential equation y = x sin y is shown. (a) Sketch the graphs of the solutions that satisfy the given initial conditions. (i) y (0) = 1 (ii) y (0) = 2 (iii) y (0) = π...

A direction field for the differential equation y = y(1 1/4y2) is shown. (a) Sketch the graphs of the solutions that satisfy the given initial conditions. (i) y(0) = 1 (ii) y(0) = 1 (iii) y(0) = 3...

The number 0 is a critical point of the autonomous differential equation dx/dt = x n , where n is a positive integer. For what values of n is 0 asymptotically stable? Semi-stable? Unstable? Repeat...

Efflux time for draining a conical tank (Fig. 7B.10), a conical tank, with dimensions given in the figure, is initially filled with a liquid. The liquid is allowed to drain out by gravity. Determine...

A particle of mass m moves in one dimension and is subject to a restoring force which is proportional to its displacement x and a damping force which is proportional to its velocity. Derive the...

Sketch the direction field of the differential equation. Then use it to sketch a solution curve that passes through the given point. 11. y' =y - 2r. (1.0) 13. y' = y + xy, (0. 1) 12. y' = 1- xy. (0....

A two-dimensional steady velocity field is given by u = x2 y2, v = 2xy. Find the streamline pattern and sketch a few lines. [Hint: The differential equation is exact.]

Using principles from physics it can be shown that when a cable is hung between two poles, it takes the shape of a curve y = f(x) that satisfies the differential equation where p is the linear...

Consider the differential equation as a model for a fish population, where is measured in weeks and is a constant. (a) Use a CAS to draw direction fields for various values of c. (b) From your...

In Problems a to c, a function f is given with domain (-, ), Indicate where f is uncreasing and where it is concave down. a. f(x) = 3x - x2 b. f(x) = x9 c. f(x) = x3 - 3x + 3

The reaction A B, r = k(T)CA, AHR = -25,000 cal/mole is to be run in aqueous solution at atmospheric pressure. (a) If the reactor is adiabatic and To 300 K, what is the highest CAo for the reactor...

Lisette's employer provides her with an insurance plan that requires her to obtain approval hospital for surgical procedures. Which variation of health coverage does this illustrate? employee...

A chemical reaction is and the products are In the law of conservation of mass, the total mass The reactants are of the reactants must equal Chemical reactions are described by which represents...

About the trajectory of an idealized projectile Given that the air resistance is negligible, the trajectory of a projectile is a combination of horizontal motion with constant velocity and vertical...

Suppose that a fish population t days from now can be modeled by an exponential function P(t) = Ae". Suppose that the fish population doubled in 90 days. By how much will the fish have multiplied...

The circuit shown in figure 1.1 has the following element values: V= 12V, R1=2.2 k, R2=1k, R3=3.3k. Apply Kirchhoffs voltage and current laws to analyse the circuit shown in figure 1.1 and determine...

The decibel level of sound in a quiet office is 10-10 watts/m2. (a) What is the corresponding sound intensity in decibels? (b) How much more intense is this sound than the least audible sound a human...

How does replacing the word reviewed with scrutinized inform your understanding of the manager and her or his actions? The manager reviewed each resume and added notes before passing it on to the...

4. Consider the IVP t y +1 y(0) = yo- (a) Without solving, determine all values of yo for which the IVP is certain to have a unique solution. (b) Solve the IVP for all such values of yo and detemine...

Study the relationship between the values of the parameter b in the differential equation dy /dt = y2 + by + I and the equilibrium points of the equation and their stability. (a) Show that for lbl >...

I have literally posted the complete assignment information, can I please have some help with these two problems? MAT 375 Module Two Guided Activity: (Continuous) Dynamical Systems Our textbook has a...

HOMEWORK NOT for TUTOR: dont submit if you dont do full THEM OR BAD RATE And in full explanation show all your work Find the volume of the solid that results from rotating the region bounded by y =...

UNIVERISTY HELPP IN HERE NOT for TUTOR: dont submit if you dont do full THEM OR BAD RATE And in full explanation show all your work Solve the following system of nonlinear equations:x^2 + y^2 = 9 x^2...

CAN YOU HELPP ME WITH MY HOMEWORK NOT for TUTOR: dont submit if you dont do full THEM OR BAD RATE And in full explanation show all your work Solve the following system of nonlinear equations:x^2 +...