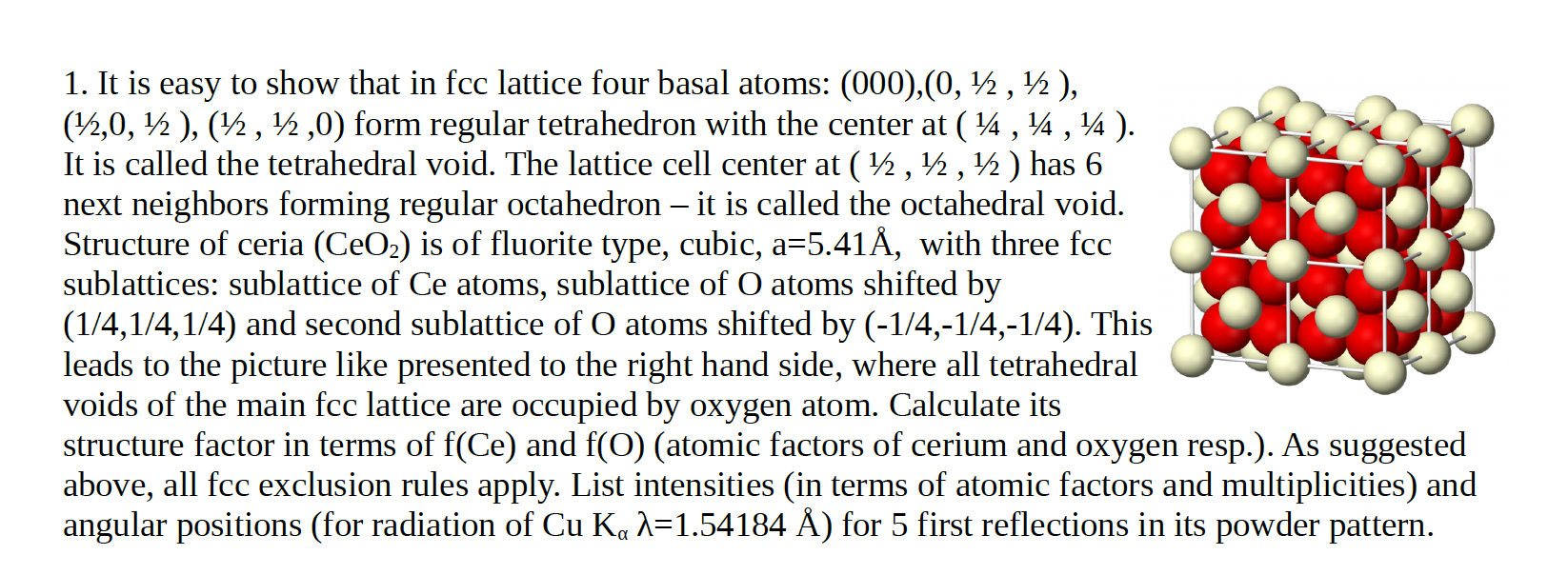
1. It is easy to show that in fcc lattice four basal atoms: (000),(0, , ), (,0, ), (, ,0) form regular tetrahedron with the center at ( , ). It is called the tetrahedral void. The lattice cell center at ( , , ) has 6 next neighbors forming regular octahedron it is called the octahedral void. Structure of ceria (CeO) is of fluorite type, cubic, a=5.41, with three fcc sublattices: sublattice of Ce atoms, sublattice of O atoms shifted by (1/4,1/4,1/4) and second sublattice of O atoms shifted by (-1/4,-1/4,-1/4). This leads to the picture like presented to the right hand side, where all tetrahedral voids of the main fcc lattice are occupied by oxygen atom. Calculate its structure factor in terms of f(Ce) and f(O) (atomic factors of cerium and oxygen resp.). As suggested above, all fcc exclusion rules apply. List intensities (in terms of atomic factors and multiplicities) and angular positions (for radiation of Cu Ka =1.54184 ) for 5 first reflections in its powder pattern.