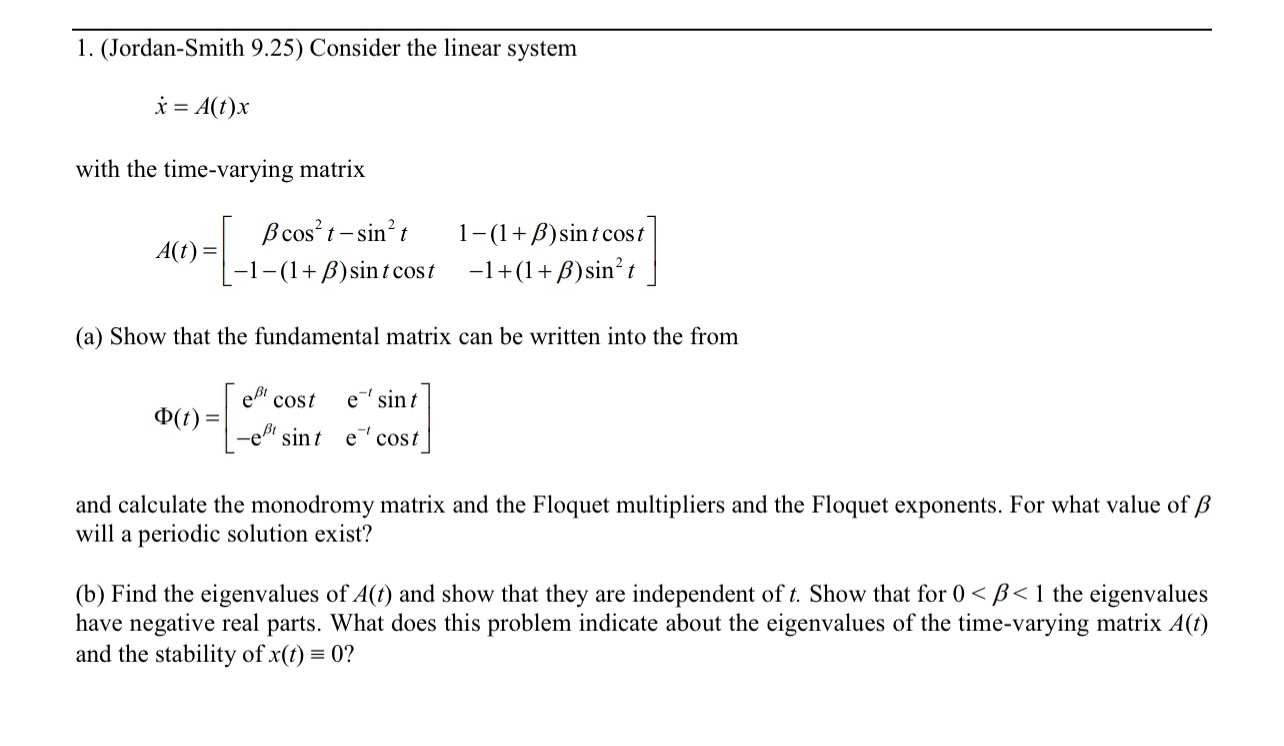
1. (Jordan-Smith 9.25) Consider the linear system x = A(t)x with the time-varying matrix A(t)= cost-sin t -1-(1+B) sint cost 1-(1+B) sint cost -1+(1+B) sin t (a) Show that the fundamental matrix can be written into the from (t)= eBi cost e' sint sint ecost and calculate the monodromy matrix and the Floquet multipliers and the Floquet exponents. For what value of B will a periodic solution exist? (b) Find the eigenvalues of A(t) and show that they are independent of t. Show that for 0