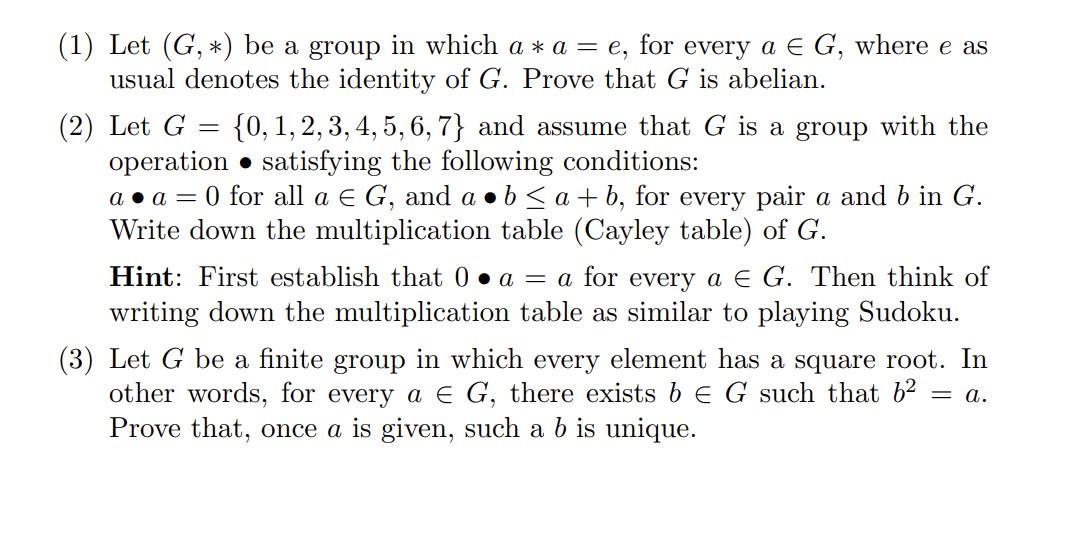
(1) Let (G, *) be a group in which a * a = e, for every a E G, where e as usual denotes the identity of G. Prove that G is abelian. (2) Let G {0, 1, 2, 3, 4, 5, 6, 7} and assume that G is a group with the operation satisfying the following conditions: a a=0 for all a E G, and a b a+b, for every pair a and b in G. Write down the multiplication table (Cayley table) of G. = Hint: First establish that 0 a = a for every a E G. Then think of writing down the multiplication table as similar to playing Sudoku. (3) Let G be a finite group in which every element has a square root. In other words, for every a E G, there exists be G such that 6