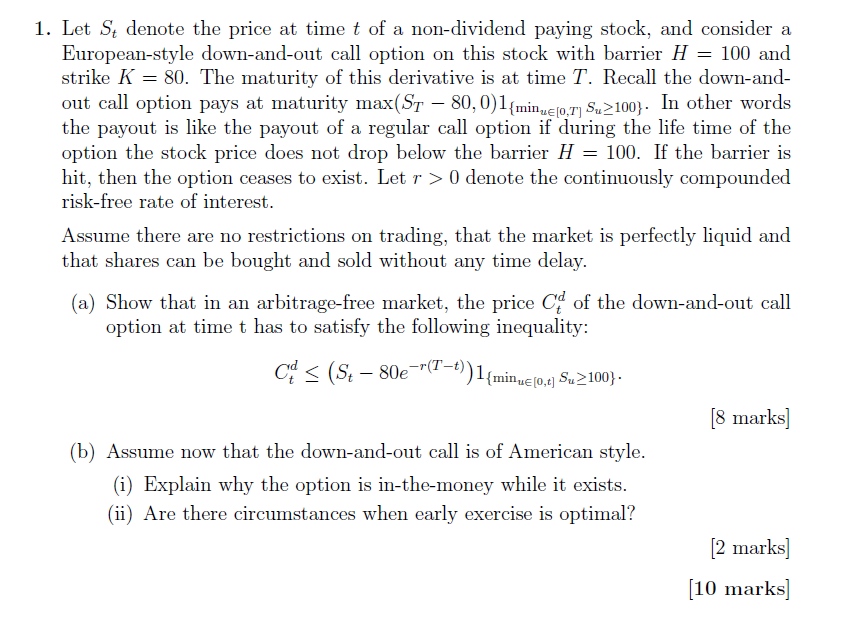
1. Let S denote the price at time t of a non-dividend paying stock, and consider a European-style down-and-out call option on this stock with barrier H = 100 and strike K = 80. The maturity of this derivative is at time T. Recall the down-and- out call option pays at maturity max(ST - 80,0)1 {mine (0,7) Su100). In other words the payout is like the payout of a regular call option if during the life time of the option the stock price does not drop below the barrier H = 100. If the barrier is hit, then the option ceases to exist. Let r> 0 denote the continuously compounded risk-free rate of interest. Assume there are no restrictions on trading, that the market is perfectly liquid and that shares can be bought and sold without any time delay. (a) Show that in an arbitrage-free market, the price Ca of the down-and-out call option at time t has to satisfy the following inequality: C (S - 80e-(T-)) 1{minu (0.t] Su100} . [8 marks] (b) Assume now that the down-and-out call is of American style. (i) Explain why the option is in-the-money while it exists. (ii) Are there circumstances when early exercise is optimal? [2 marks] [10 marks] 1. Let S denote the price at time t of a non-dividend paying stock, and consider a European-style down-and-out call option on this stock with barrier H = 100 and strike K = 80. The maturity of this derivative is at time T. Recall the down-and- out call option pays at maturity max(ST - 80,0)1 {mine (0,7) Su100). In other words the payout is like the payout of a regular call option if during the life time of the option the stock price does not drop below the barrier H = 100. If the barrier is hit, then the option ceases to exist. Let r> 0 denote the continuously compounded risk-free rate of interest. Assume there are no restrictions on trading, that the market is perfectly liquid and that shares can be bought and sold without any time delay. (a) Show that in an arbitrage-free market, the price Ca of the down-and-out call option at time t has to satisfy the following inequality: C (S - 80e-(T-)) 1{minu (0.t] Su100} . [8 marks] (b) Assume now that the down-and-out call is of American style. (i) Explain why the option is in-the-money while it exists. (ii) Are there circumstances when early exercise is optimal? [2 marks] [10 marks]