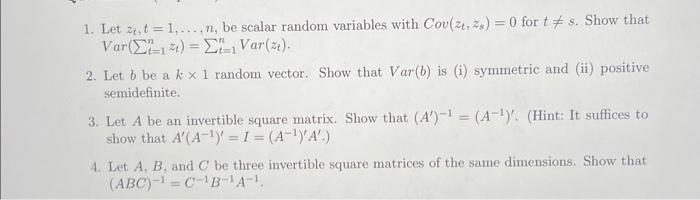
1. Let z,t = 1,...,n, be scalar random variables with Cov(zt, zs) = 0 for ts. Show that Var(-1)=E=1 Var(t). 2. Let b be a k x 1 random vector. Show that Var(b) is (i) symmetric and (ii) positive semidefinite. 3. Let A be an invertible square matrix. Show that (A)-1 = (A-). (Hint: It suffices to show that A'(A-)= I=(A-)'A'.) 4. Let A, B, and C be three invertible square matrices of the same dimensions. Show that (ABC)-=CB-1A-.