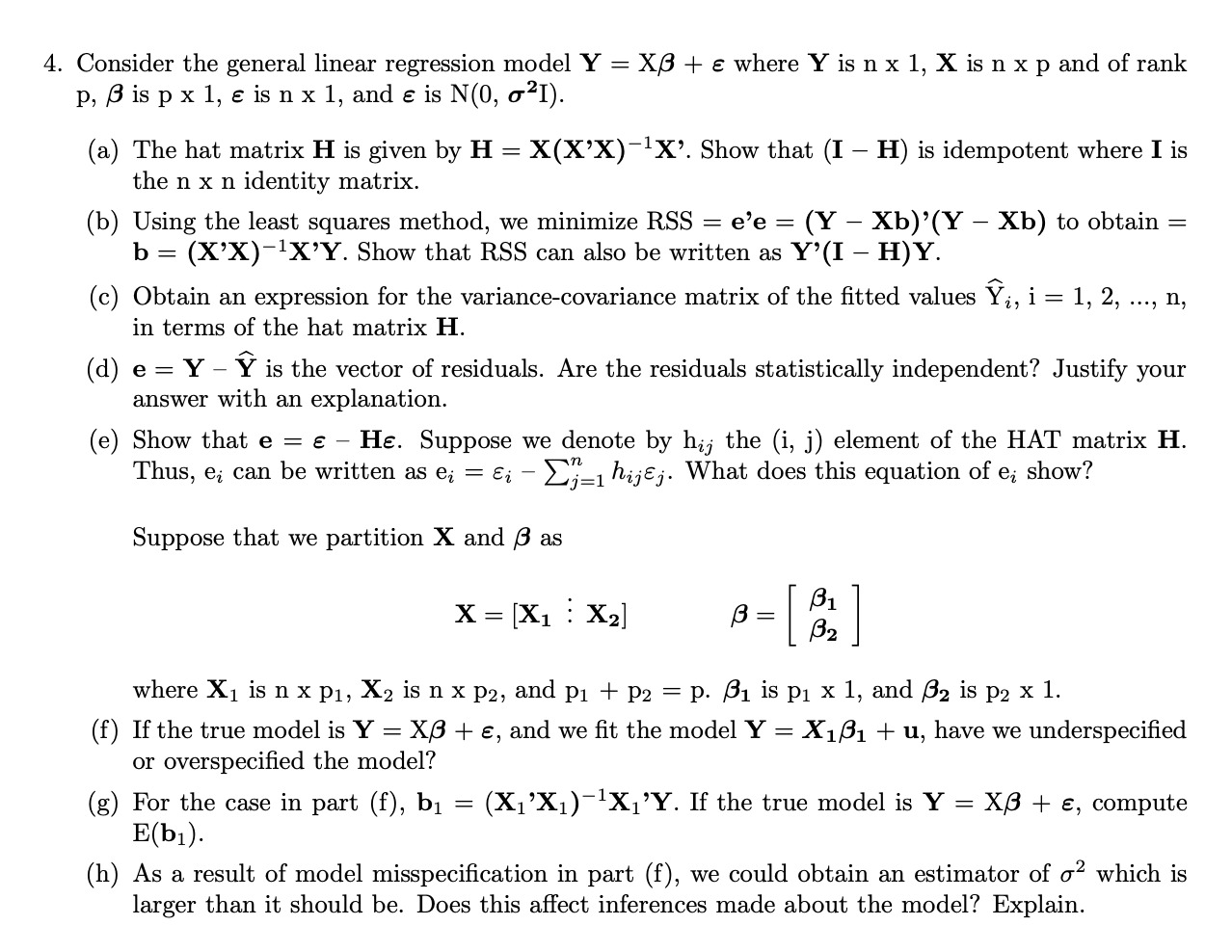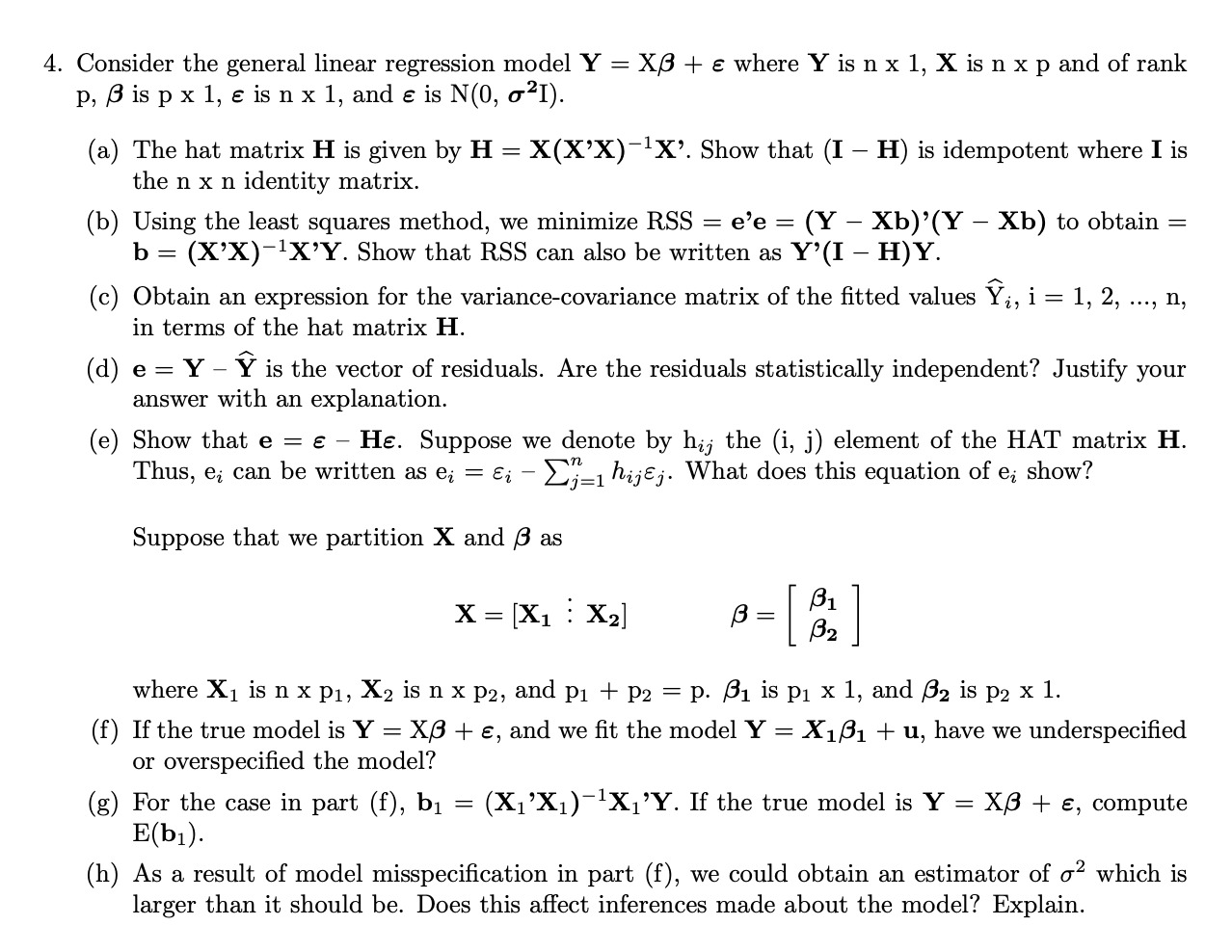

1 . People respond to incentives. Governments can alter incentives and, hence, behaviour with public policy. However, sometimes public policy generates unintended consequences by producing results that were not anticipated. Try to find an unintended consequence of each of the following public policies. a. To help the "working poor," the government raises the minimum wage to 625 per hour. b. To help the homeless, the government places rent controls on apartments restricting rent to 650 per month. C. To reduce its budget deficit and limit consumption of petrol, the government raises the tax on petrol by (1.00 per litre. d. To reduce the consumption of drugs, the government makes drugs illegal. e. To raise the population of a rare bird of prey, the government prohibits the killing of the birds and the collecting of their eggs. f. To improve the welfare of European sugar beet growers, the EU bans imports of sugar from South America. 2. Opportunity cost is what you give up to get an item. Since there is no such thing as a free lunch, what would likely be given up to obtain each of the items listed below? a. Susan can work full time or go to university. She chooses university. b. Susan can work full time or go to university. She chooses work. C. Farmer Jones has 100 hectares of land. He can plant wheat, which yields 5 tonnes per hectare, or he can plant potatoes, which yield 35 tonnes per hectare. He chooses to plant wheat. d. Farmer Jones has 100 hectares of land. He can plant wheat, which yields 5 tonnes per hectare, or he can plant potatoes, which yield 35 tonnes per hectare. He chooses to plant potatoes. e. In (a) and (b) above, and (c) and (d) above, which is the opportunity cost of which - college for work or work for college? Potatoes for wheat or wheat for potatoes?2. Model the topic using a differential equation. a) Draw any visuals (diagrams) that exemplify the model and facilitate understanding of the modeling process. Your visuals should represent the topic and help develop the differential equation. b) Write the differential equation that models your topic of choice. Define all the variables used in the model, and be sure to include initial conditions. c) Classify the differential equation, including the order and if it is linear or nonlinear. 3. Solve the differential equation using the techniques from this course. a) Provide a valid solution to the differential equation. Be sure to apply the initial conditions that you developed. b) Discuss the method used to solve the differential equation. Explain all steps in arriving at the solution, writing in paragraph form and in complete sentences. 4. Discuss the solution In context of the initial topic. Write the solution of the differential equation in terms of the initial topic you chose in Part 1. Describe how this could be useful for someone in the field from which the model was obtained. Include solution values or graphics to illustrate the utility and applicability of the solution in the context of the topic youchose. 5. Discuss what you learned from the modeling process. Write one to two paragraphs pertaining to what you can take away from the modeling process in general.4. Consider the general linear regression model Y = X3 + 5 where Y is n x 1, X is n x p and of rank p,ispx1,eisnx1,andeis N(D,a'21). (a) The hat matrix H is given by H = X(X'X)_1X'. Show that (I H) is idempotent where I is the n x n identity matrix. (b) Using the least squares method, we minimize RSS = e'e = (Y Xb)'(Y Xb) to obtain = b = (X'X)'1X'Y. Show that RSS can also be written as Y'(I H)Y. (c) Obtain an expression for the variancecovariance matrix of the tted values {1%, i = 1, 2, ..., n, in terms of the hat matrix H. (d) e = Y '1" is the vector of residuals. Are the residuals statistically independent? Justify your answer with an explanation. (e) Show that e = e He. Suppose we denote by hj the (i, j) element of the HAT matrix H. Thus, e1; can be written as e,' = 5, 23.1:1 hjej. What does this equation of ez- show? Suppose that we partition X and [3 as - 51 X = X I X = [ 1 2] 16 [ 32 whereX1 is nxpl, X2 is nxpg, and p1 + p2 = p. ,61 is p1 x 1, and 162 is pg 3: 1. (f) If the true model is Y = X + E, and we t the model Y = Xll + u, have we underspecied 0r overspecied the model? (g) For the case in part (f), bl = (Xl'X1)_1X1'Y. If the true model is Y = X + e, compute E(b1). (h) As a result of model misspecication in part (f), we could Obtain an estimator of 0'2 which is larger than it should be. Does this affect inferences made about the model? Explain. 1. Consider a perfectly competitive economy with two goods (I and y) and a single consumer. The consumer is endowed with s {> 0} units of thee which are supplied inelasticale to the labor market. Both goods (:1: and y} are produced by perfectly competitive rms using one unit of labor to produce one unit of good 3': or good 3;. The consumer chooses consumption of goods e and y to maximize his utility: U = olnm+lny, where lI] cm > {1:3 > U . 0 (16:1 5. ,33