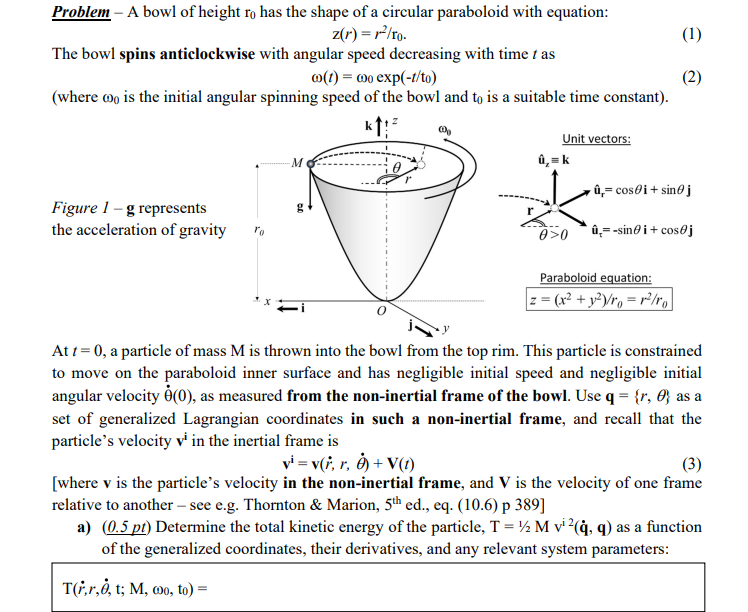
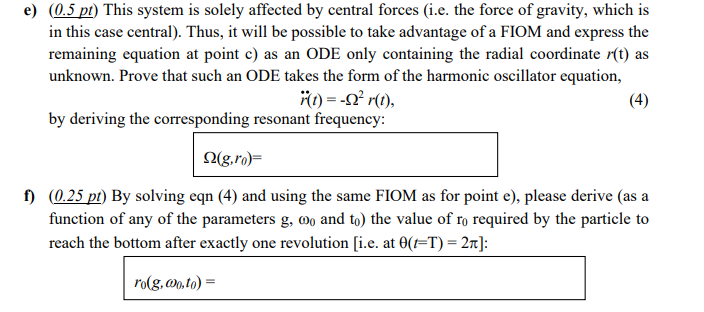
(1) Problem - A bowl of height ro has the shape of a circular paraboloid with equation: z(r) = r/ro. The bowl spins anticlockwise with angular speed decreasing with time t as o(t) = 00 exp(-1/to) (where wo is the initial angular spinning speed of the bowl and to is a suitable time constant). (2) 01 Unit vectors: =k M e ,= cosi + sinj Figure 1 - g represents the acceleration of gravity Po 0>0 =-sini + cos@j Paraboloid equation: z = (x2 + y2V/ro = r2/ro At t=0, a particle of mass M is thrown into the bowl from the top rim. This particle is constrained to move on the paraboloid inner surface and has negligible initial speed and negligible initial angular velocity (0), as measured from the non-inertial frame of the bowl. Use q = {r, 8; as a set of generalized Lagrangian coordinates in such a non-inertial frame, and recall that the particle's velocity v' in the inertial frame is vi = v(i, r, .) + V(1) (3) [where v is the particle's velocity in the non-inertial frame, and V is the velocity of one frame relative to another see e.g. Thornton & Marion, 5th ed., eq. (10.6) p 389] a) (0.5 pt) Determine the total kinetic energy of the particle, T = 1 M vi?(q, q) as a function of the generalized coordinates, their derivatives, and any relevant system parameters: T(',r,',t; M, 00, to) = b) (0.5 pt) Derive the system Lagrangian, La, q): L(1,r,, t; M, g, ro, m0, to) = Prove that L(, q) is equivalent to the corresponding Lagrangian in the inertial frame, plus a fictitious velocity-dependent, time-dependent potential Ufict(r,, t), by deriving Ufict(r,0, t; M, 00, to) c) (0.75 pt) Derive two Lagrange equations for the system in the non-inertial coordinates r and 0, respectively: dal/6)/dt = al/20 = dal/dr) /dt = allor= d) (0.5 pt) How many first integrals of motion (FIOM) does this system have? NFIOM = Please indicate all of them in the box below: e) (0.5 pt) This system is solely affected by central forces (i.e. the force of gravity, which is in this case central). Thus, it will be possible to take advantage of a FIOM and express the remaining equation at point c) as an ODE only containing the radial coordinate r(t) as unknown. Prove that such an ODE takes the form of the harmonic oscillator equation, Ft) = -12 r(t), (4) by deriving the corresponding resonant frequency: S2(g,ro)= f) (0.25 pt) By solving eqn (4) and using the same FIOM as for point e), please derive (as a function of any of the parameters g, 0, and to the value of ro required by the particle to reach the bottom after exactly one revolution [i.e. at 0(t=T) = 21]: ro(g,00,to) (1) Problem - A bowl of height ro has the shape of a circular paraboloid with equation: z(r) = r/ro. The bowl spins anticlockwise with angular speed decreasing with time t as o(t) = 00 exp(-1/to) (where wo is the initial angular spinning speed of the bowl and to is a suitable time constant). (2) 01 Unit vectors: =k M e ,= cosi + sinj Figure 1 - g represents the acceleration of gravity Po 0>0 =-sini + cos@j Paraboloid equation: z = (x2 + y2V/ro = r2/ro At t=0, a particle of mass M is thrown into the bowl from the top rim. This particle is constrained to move on the paraboloid inner surface and has negligible initial speed and negligible initial angular velocity (0), as measured from the non-inertial frame of the bowl. Use q = {r, 8; as a set of generalized Lagrangian coordinates in such a non-inertial frame, and recall that the particle's velocity v' in the inertial frame is vi = v(i, r, .) + V(1) (3) [where v is the particle's velocity in the non-inertial frame, and V is the velocity of one frame relative to another see e.g. Thornton & Marion, 5th ed., eq. (10.6) p 389] a) (0.5 pt) Determine the total kinetic energy of the particle, T = 1 M vi?(q, q) as a function of the generalized coordinates, their derivatives, and any relevant system parameters: T(',r,',t; M, 00, to) = b) (0.5 pt) Derive the system Lagrangian, La, q): L(1,r,, t; M, g, ro, m0, to) = Prove that L(, q) is equivalent to the corresponding Lagrangian in the inertial frame, plus a fictitious velocity-dependent, time-dependent potential Ufict(r,, t), by deriving Ufict(r,0, t; M, 00, to) c) (0.75 pt) Derive two Lagrange equations for the system in the non-inertial coordinates r and 0, respectively: dal/6)/dt = al/20 = dal/dr) /dt = allor= d) (0.5 pt) How many first integrals of motion (FIOM) does this system have? NFIOM = Please indicate all of them in the box below: e) (0.5 pt) This system is solely affected by central forces (i.e. the force of gravity, which is in this case central). Thus, it will be possible to take advantage of a FIOM and express the remaining equation at point c) as an ODE only containing the radial coordinate r(t) as unknown. Prove that such an ODE takes the form of the harmonic oscillator equation, Ft) = -12 r(t), (4) by deriving the corresponding resonant frequency: S2(g,ro)= f) (0.25 pt) By solving eqn (4) and using the same FIOM as for point e), please derive (as a function of any of the parameters g, 0, and to the value of ro required by the particle to reach the bottom after exactly one revolution [i.e. at 0(t=T) = 21]: ro(g,00,to)