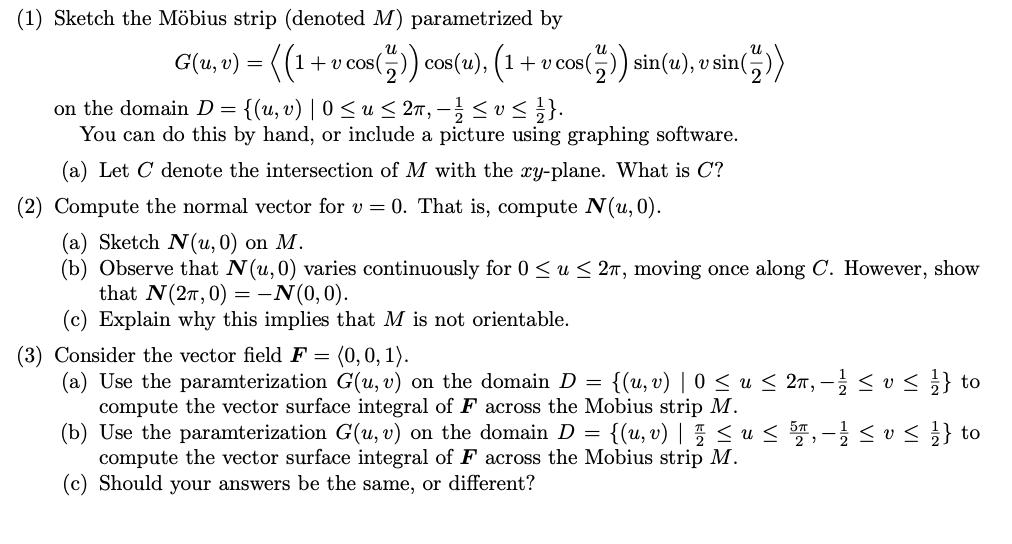
(1) Sketch the Mbius strip (denoted M) parametrized by G(u, v) = ((1 os()) cos(u), (1 + v cos()) sin(u), v sin()) 1+ v cos( on the domain D = {(u, v) |0 u 2, -1/2 v 1}. You can do this by hand, or include a picture using graphing software. (a) Let C denote the intersection of M with the xy-plane. What is C? (2) Compute the normal vector for v = 0. That is, compute N(u,0). (a) Sketch N(u,0) on M. (b) Observe that N(u,0) varies continuously for 0 u 2, moving once along C. However, show that N (2, 0) = -N(0,0). (c) Explain why this implies that M is not orientable. (3) Consider the vector field F = (0, 0, 1). (a) Use the paramterization G(u, v) on the domain D = {(u, v) | 0 u 2, - v 2} to compute the vector surface integral of F across the Mobius strip M. (b) Use the paramterization G(u, v) on the domain D = {(u, v)| u 5T, -1/2 v 2} to compute the vector surface integral of F across the Mobius strip M. (c) Should your answers be the same, or different?