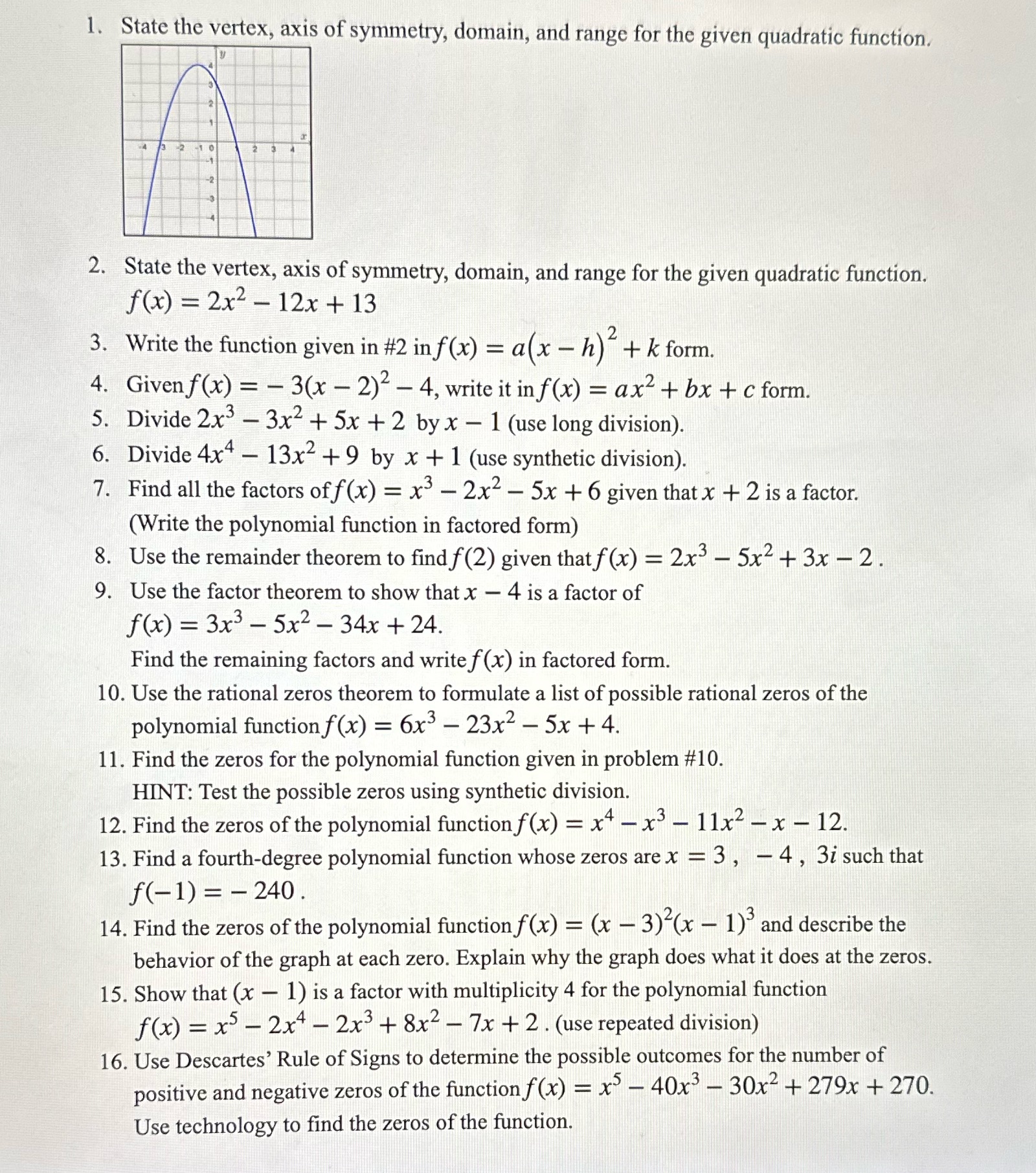
1. State the vertex, axis of symmetry, domain, and range for the given quadratic function. 4 1 13 2 -10 149 y 2. State the vertex, axis of symmetry, domain, and range for the given quadratic function. f(x)=2x - 12x + 13 1 3. Write the function given in #2 in f(x) = a (x - h) + k form. 4. Given f(x) = -3(x - 2)-4, write it in f(x) = ax+bx+c form. 5. Divide 2x-3x + 5x +2 by x - 1 (use long division). 6. Divide 4x4- 13x +9 by x + 1 (use synthetic division). 7. Find all the factors off(x) = x - 2x - 5x + 6 given that x + 2 is a factor. (Write the polynomial function in factored form) 8. Use the remainder theorem to find f(2) given thatf(x) = 2x 5x + 3x - 2. 9. Use the factor theorem to show that x - 4 is a factor of f(x) = 3x - 5x - 34x + 24. Find the remaining factors and write f(x) in factored form. 10. Use the rational zeros theorem to formulate a list of possible rational zeros of the polynomial function f(x) = 6x - 23x5x +4. 11. Find the zeros for the polynomial function given in problem #10. HINT: Test the possible zeros using synthetic division. 12. Find the zeros of the polynomial function f(x) = x4 -x-11x - x - 12. 13. Find a fourth-degree polynomial function whose zeros are x = 3, 4, 3i such that f(-1)=240. 14. Find the zeros of the polynomial function f(x) = (x 3)(x - 1) and describe the behavior of the graph at each zero. Explain why the graph does what it does at the zeros. 15. Show that (x - 1) is a factor with multiplicity 4 for the polynomial function f(x) = x 2x4 2x + 8x 7x + 2 . (use repeated division) - 16. Use Descartes' Rule of Signs to determine the possible outcomes for the number of positive and negative zeros of the function f(x) = x5 - 40x - 30x + 279x + 270. Use technology to find the zeros of the function.