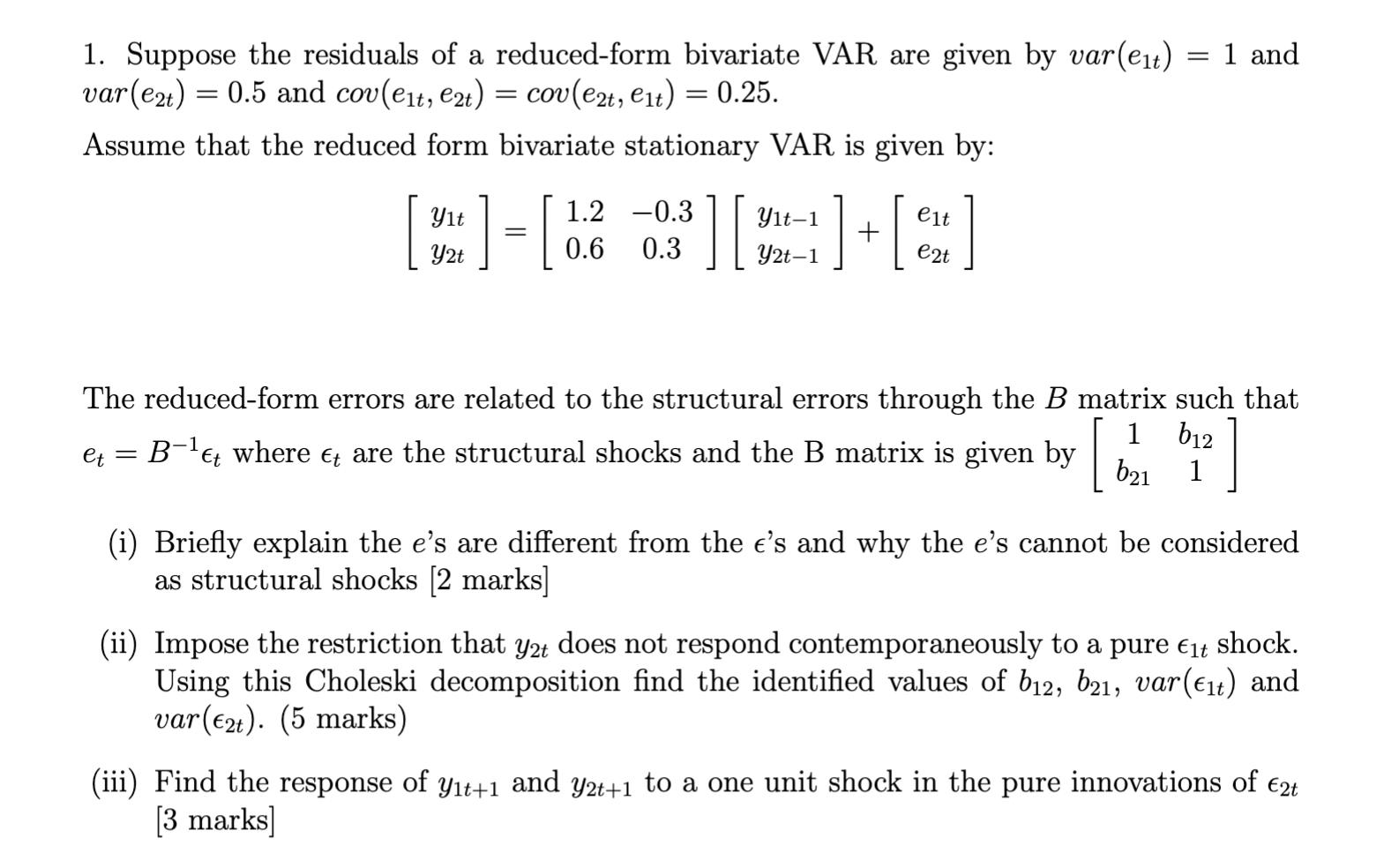
1. Suppose the residuals of a reduced-form bivariate VAR are given by var(et) var (e2t) = 0.5 and cov(e1t, e2t) = cov(e2t, e1t) = 0.25. Assume that the reduced form bivariate stationary VAR is given by: Y1t Y2t = [ 1.2 -0.3 0.6 0.3 ] [ Y1t-1 e1t + Y2t-1 e2t = : 1 and The reduced-form errors are related to the structural errors through the B matrix such that -1 et = Et Bt where t are the structural shocks and the B matrix is given by [ 1 b12 b21 1 ] (i) Briefly explain the e's are different from the e's and why the e's cannot be considered as structural shocks [2 marks] (ii) Impose the restriction that y2t does not respond contemporaneously to a pure t shock. Using this Choleski decomposition find the identified values of b12, b21, var(Et) and var (2t). (5 marks) (iii) Find the [3 marks] response of Y1t+1 and Y2t+1 to a one unit shock in the pure innovations of 2t