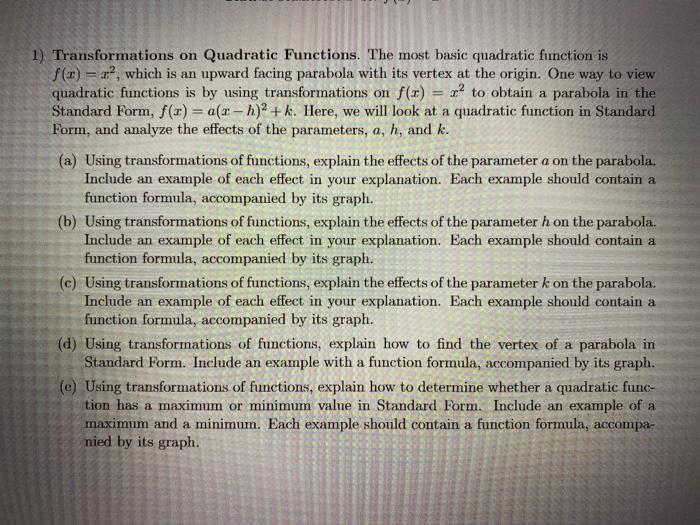
1) Transformations on Quadratic Functions. The most basic quadratic function is f(x) = x, which is an upward facing parabola with its vertex at the origin. One way to view quadratic functions is by using transformations on f(x) x to obtain a parabola in the Standard Form, f(x) = a(x-h)2+k. Here, we will look at a quadratic function in Standard Form, and analyze the effects of the parameters, a, h, and k. = (a) Using transformations of functions, explain the effects of the parameter a on the parabola. Include an example of each effect in your explanation. Each example should contain a function formula, accompanied by its graph. (b) Using transformations of functions, explain the effects of the parameter h on the parabola. Include an example of each effect in your explanation. Each example should contain a function formula, accompanied by its graph. (c) Using transformations of functions, explain the effects of the parameter k on the parabola. Include an example of each effect in your explanation. Each example should contain a function formula, accompanied by its graph. (d) Using transformations of functions, explain how to find the vertex of a parabola in Standard Form. Include an example with a function formula, accompanied by its graph. (e) Using transformations of functions, explain how to determine whether a quadratic func- tion has a maximum or minimum value in Standard Form. Include an example of a maximum and a minimum. Each example should contain a function formula, accompa- nied by its graph.