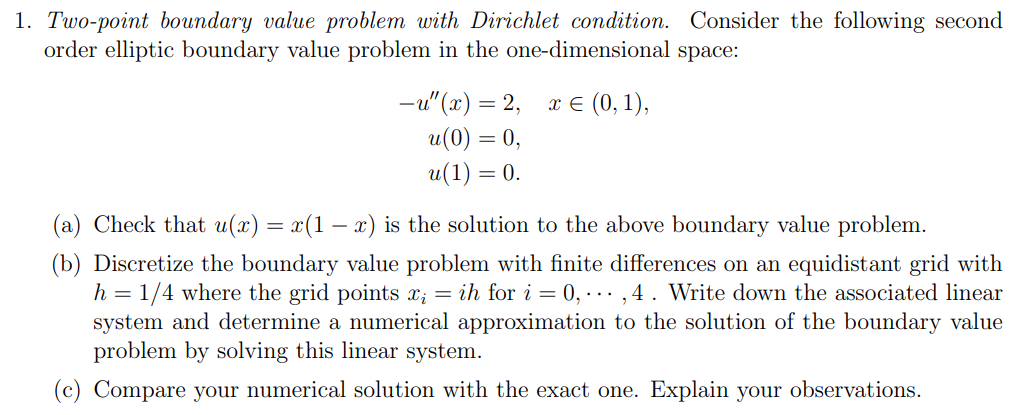
1. Two-point boundary value problem with Dirichlet condition. Consider the following second order elliptic boundary value problem in the one-dimensional space: - -u" (x) = 2, x = (0, 1), u(0) = 0, u(1) = 0. (a) Check that u(x) = x(1 x) is the solution to the above boundary value problem. (b) Discretize the boundary value problem with finite differences on an equidistant grid with h = 1/4 where the grid points x; = ih for i = 0,...,4. Write down the associated linear system and determine a numerical approximation to the solution of the boundary value problem by solving this linear system. (c) Compare your numerical solution with the exact one. Explain your observations.