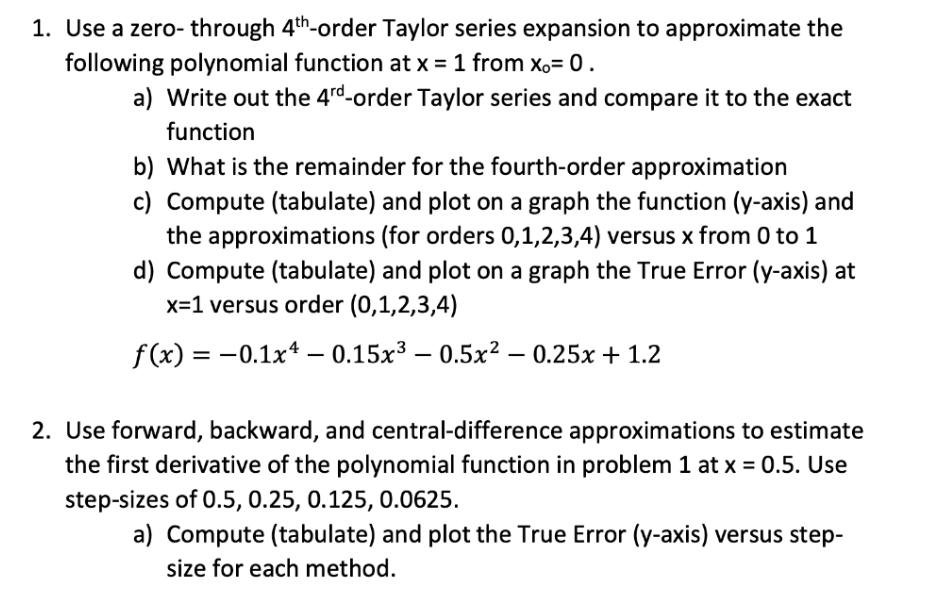
1. Use a zero- through 4th-order Taylor series expansion to approximate the following polynomial function at x = 1 from x= 0. a) Write out the 4rd-order Taylor series and compare it to the exact function b) What is the remainder for the fourth-order approximation c) Compute (tabulate) and plot on a graph the function (y-axis) and the approximations (for orders 0,1,2,3,4) versus x from 0 to 1 d) Compute (tabulate) and plot on a graph the True Error (y-axis) at x=1 versus order (0,1,2,3,4) f(x) = -0.1x4 -0.15x -0.5x -0.25x + 1.2 2. Use forward, backward, and central-difference approximations to estimate the first derivative of the polynomial function in problem 1 at x = 0.5. Use step-sizes of 0.5, 0.25, 0.125, 0.0625. a) Compute (tabulate) and plot the True Error (y-axis) versus step- size for each method.