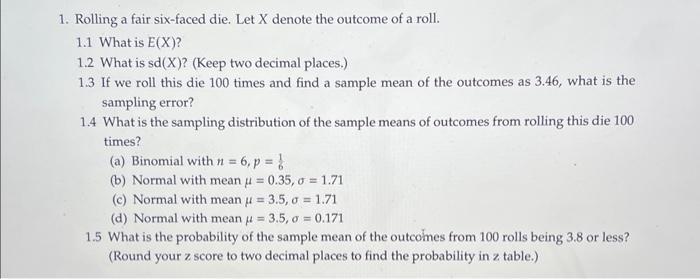
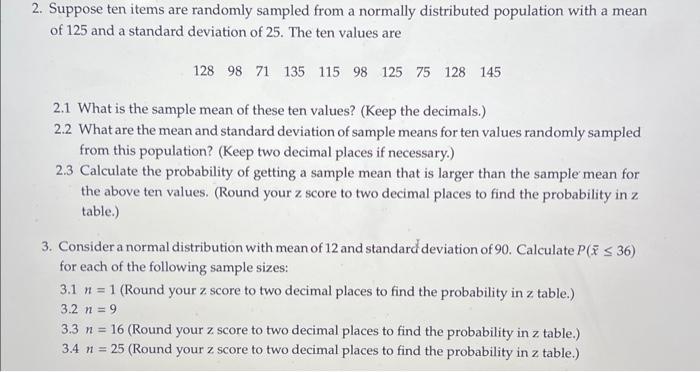
1. Rolling a fair six-faced die. Let X denote the outcome of a roll. 1.1 What is E(X)? 1.2 What is sd(X)? (Keep two decimal places.) 1.3 If we roll this die 100 times and find a sample mean of the outcomes as 3.46, what is the sampling error? 1.4 What is the sampling distribution of the sample means of outcomes from rolling this die 100 times? (a) Binomial with n = 6, p = } (b) Normal with mean = 0.35, o = 1.71 (c) Normal with mean u = 3.5, o = 1.71 (d) Normal with mean = 3.5, 0 = 0.171 1.5 What is the probability of the sample mean of the outcomes from 100 rolls being 3.8 or less? (Round your z score to two decimal places to find the probability in z table.) 2. Suppose ten items are randomly sampled from a normally distributed population with a mean of 125 and a standard deviation of 25. The ten values are 128 98 71 135 115 98 125 75 128 145 2.1 What is the sample mean of these ten values? (Keep the decimals.) 2.2 What are the mean and standard deviation of sample means for ten values randomly sampled from this population? (Keep two decimal places if necessary.) 2.3 Calculate the probability of getting a sample mean that is larger than the sample mean for the above ten values. (Round your z score to two decimal places to find the probability in z table.) 3. Consider a normal distribution with mean of 12 and standard deviation of 90. Calculate P(x 36) for each of the following sample sizes: 3.1 n = 1 (Round your z score to two decimal places to find the probability in z table.) 3.2 n = 9 3.3 n 16 (Round your z score to two decimal places to find the probability in z table.) 3.4 n = 25 (Round your z score to two decimal places to find the probability in z table.) 4. If we flip a fair coin 200 times: 4.1 What is the expected value of the proportion of heads in this 200 flips? 4.2 What is the standard deviation of the proportion of heads in 200 flips? (Keep three significant figures.) 4.3 Find the probability the proportion of heads in 200 flips will be between 0.47 and 0.51. (Round your z score to two decimal places to find the probability in z table.) 4.4 Referring to Question 4.3, what would the probability be if the sample size were 100?