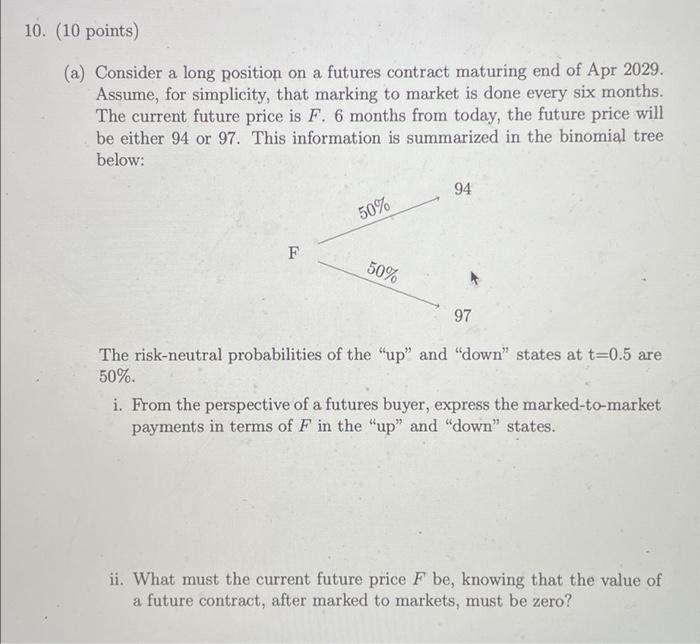
10. (10 points) (a) Consider a long position on a futures contract maturing end of Apr 2029. Assume, for simplicity, that marking to market is done every six months. The current future price is F. 6 months from today, the future price will be either 94 or 97. This information is summarized in the binomial tree below: 94 50% F 50% 97 The risk-neutral probabilities of the "up" and "down" states at t=0.5 are 50% i. From the perspective of a futures buyer, express the marked-to-market payments in terms of F in the "up" and "down" states. ii. What must the current future price F be, knowing that the value of a future contract, after marked to markets, must be zero? iii. How does your answer in part ii. change if the risk-neutral probability of the "up' state increases to 60% and the probability of the "down" state decreases to 40%, holding all else constant? 18 (b) We have seen in class that in choosing the cheapest bond to deliver, it is optimal for a futures seller to choose a bond with the smallest ratio of price to conversion factor. That is, the futures seller chooses to minimize the ratio of price to conversion factor; (b) We have seen in class that in choosing the cheapest bond to deliver, it is optimal for a futures seller to choose a bond with the smallest ratio of price to conversion factor. That is, the futures seller chooses to minimize the ratio of price to conversion factor: P(y) P(6%) where P() is the bond pricing function and y is the yield to maturity of the bond. Explain why in a low interest rate environment, the cheapest bonds to deliver tend to be bonds with low durations whereas in a high interest rate environment, high duration bonds are cheaper for delivery. (c) Consider two AAA-rated fixed income securities: A one-year zero coupon bond issued by Microsoft. A one-year zero coupon senior tranche CDO backed by a wide range of corporate bonds. The two securities have the same face value and the same expected credit loss. That is, both the timing as well as expected values of the payoffs are identical between the two securities. Should these two securities command the same prices? Very briefly explain your reasoning 10. (10 points) (a) Consider a long position on a futures contract maturing end of Apr 2029. Assume, for simplicity, that marking to market is done every six months. The current future price is F. 6 months from today, the future price will be either 94 or 97. This information is summarized in the binomial tree below: 94 50% F 50% 97 The risk-neutral probabilities of the "up" and "down" states at t=0.5 are 50% i. From the perspective of a futures buyer, express the marked-to-market payments in terms of F in the "up" and "down" states. ii. What must the current future price F be, knowing that the value of a future contract, after marked to markets, must be zero? iii. How does your answer in part ii. change if the risk-neutral probability of the "up' state increases to 60% and the probability of the "down" state decreases to 40%, holding all else constant? 18 (b) We have seen in class that in choosing the cheapest bond to deliver, it is optimal for a futures seller to choose a bond with the smallest ratio of price to conversion factor. That is, the futures seller chooses to minimize the ratio of price to conversion factor; (b) We have seen in class that in choosing the cheapest bond to deliver, it is optimal for a futures seller to choose a bond with the smallest ratio of price to conversion factor. That is, the futures seller chooses to minimize the ratio of price to conversion factor: P(y) P(6%) where P() is the bond pricing function and y is the yield to maturity of the bond. Explain why in a low interest rate environment, the cheapest bonds to deliver tend to be bonds with low durations whereas in a high interest rate environment, high duration bonds are cheaper for delivery. (c) Consider two AAA-rated fixed income securities: A one-year zero coupon bond issued by Microsoft. A one-year zero coupon senior tranche CDO backed by a wide range of corporate bonds. The two securities have the same face value and the same expected credit loss. That is, both the timing as well as expected values of the payoffs are identical between the two securities. Should these two securities command the same prices? Very briefly explain your reasoning