1.0 Part A (1st Order Differential Equations) 1.1 Introduction 1.1.1 Background of engineering application and field of study 1.1.2 Importance of the problem and solution 1.1.3 Objective 1.2 Mathematical formulation 1.2.1 Variables involved 1.2.2 Derivation of equations 1.2.3 Method of solution 1.2.4 General solution 1.2.5 Boundary/initial conditions 1.2.6 Particular solution 1.2.7 Sample calculations 1.3 Discussion of the results. Explain with the help of graphs etc. what do the results indicate and their significance.

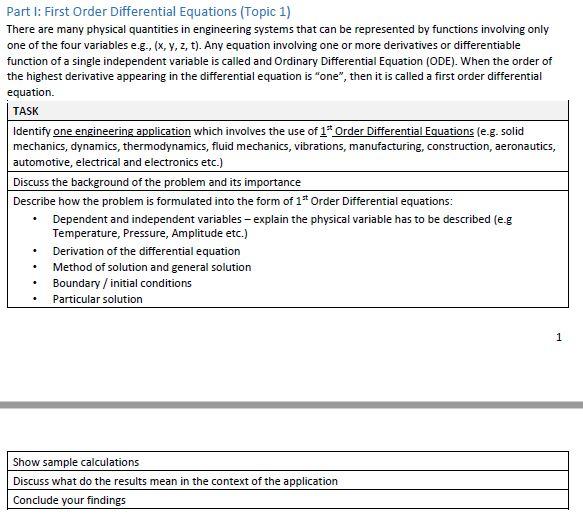
Part 1: First Order Differential Equations (Topic 1) There are many physical quantities in engineering systems that can be represented by functions involving only one of the four variables e.g., (X,Y, 2, t). Any equation involving one or more derivatives or differentiable function of a single independent variable is called and Ordinary Differential Equation (ODE). When the order of the highest derivative appearing in the differential equation is "one", then it is called a first order differential equation. TASK Identify one engineering application which involves the use of 1* Order Differential Equations (e.g. solid mechanics, dynamics, thermodynamics, fluid mechanics, vibrations, manufacturing, construction, aeronautics, automotive, electrical and electronics etc.) Discuss the background of the problem and its importance Describe how the problem is formulated into the form of 1* Order Differential equations: Dependent and independent variables explain the physical variable has to be described (eg Temperature, Pressure, Amplitude etc.) Derivation of the differential equation Method of solution and general solution Boundary / initial conditions Particular solution 1 Show sample calculations Discuss what do the results mean in the context of the application Conclude your findings Part 1: First Order Differential Equations (Topic 1) There are many physical quantities in engineering systems that can be represented by functions involving only one of the four variables e.g., (X,Y, 2, t). Any equation involving one or more derivatives or differentiable function of a single independent variable is called and Ordinary Differential Equation (ODE). When the order of the highest derivative appearing in the differential equation is "one", then it is called a first order differential equation. TASK Identify one engineering application which involves the use of 1* Order Differential Equations (e.g. solid mechanics, dynamics, thermodynamics, fluid mechanics, vibrations, manufacturing, construction, aeronautics, automotive, electrical and electronics etc.) Discuss the background of the problem and its importance Describe how the problem is formulated into the form of 1* Order Differential equations: Dependent and independent variables explain the physical variable has to be described (eg Temperature, Pressure, Amplitude etc.) Derivation of the differential equation Method of solution and general solution Boundary / initial conditions Particular solution 1 Show sample calculations Discuss what do the results mean in the context of the application Conclude your findings