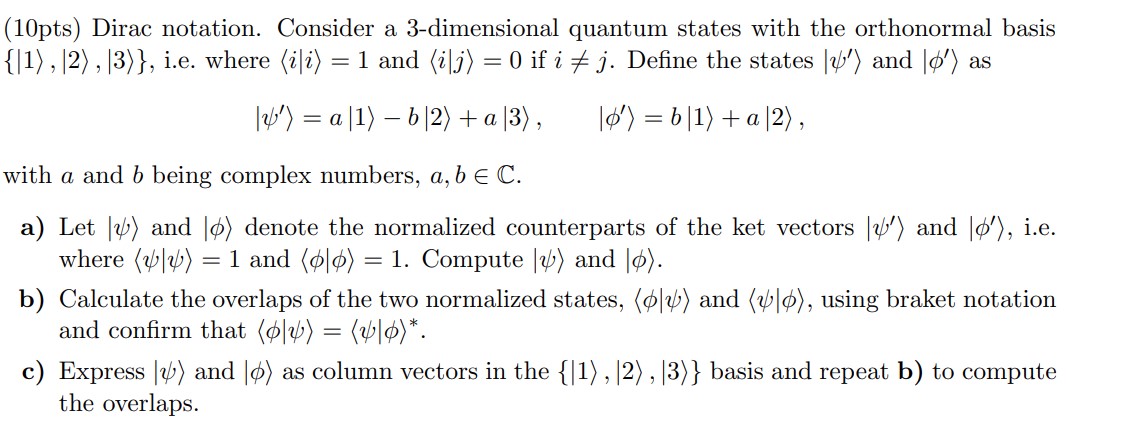
(10pts) Dirac notation. Consider a 3-dimensional quantum states with the orthonormal basis {1), 2), 3)}, i.e. where (ii) = = 1 and (ij) = 0 if i j. Define the states 4') and 0') as |') = a |1b|2) + a |3), |0')=b1a |2), with a and b being complex numbers, a, b = C. a) Let | and |) denote the normalized counterparts of the ket vectors (4) and '), i.e. where () = 1 and (0|) = 1. Compute [) and [). b) Calculate the overlaps of the two normalized states, (0|4) and (0), using braket notation and confirm that (0|4) = (4|6)*. c) Express) and |) as column vectors in the {|1), |2), |3)} basis and repeat b) to compute the overlaps.