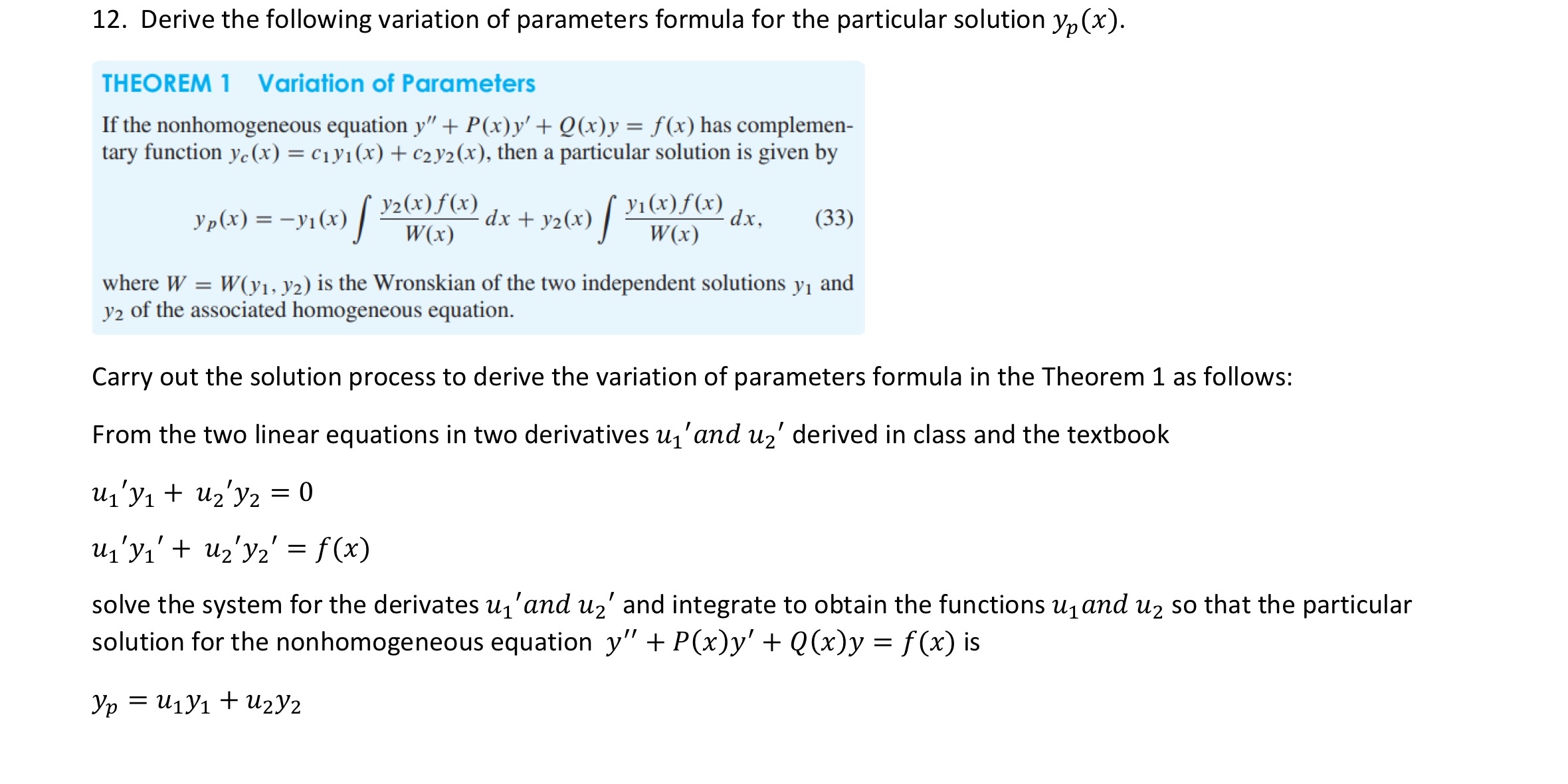
12. Derive the following variation of parameters formula for the particular solution y(x). THEOREM 1 Variation of Parameters If the nonhomogeneous equation y" + P(x)y' + Q(x)y = f(x) has complemen- tary function ye(x) = Cy1(x) + C2y2(x), then a particular solution is given by Yp(x) = y (x) y2(x)f(x) dx + y2(x) W(x) S y1(x) f(x) W(x) dx, (33) where W = W(y1, y2) is the Wronskian of the two independent solutions y2 of the associated homogeneous equation. and Carry out the solution process to derive the variation of parameters formula in the Theorem 1 as follows: From the two linear equations in two derivatives u' and u' derived in class and the textbook u'y1u2y2 = 0 u'y' + u'y' = f(x) solve the system for the derivates u'and u' and integrate to obtain the functions u and u so that the particular solution for the nonhomogeneous equation y" + P(x)y' + Q(x)y = f(x) is = U1Y1 +