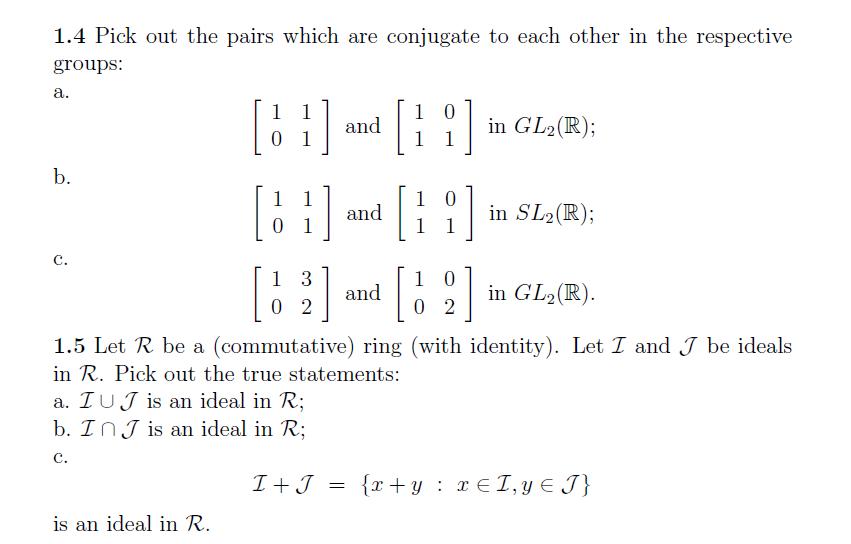
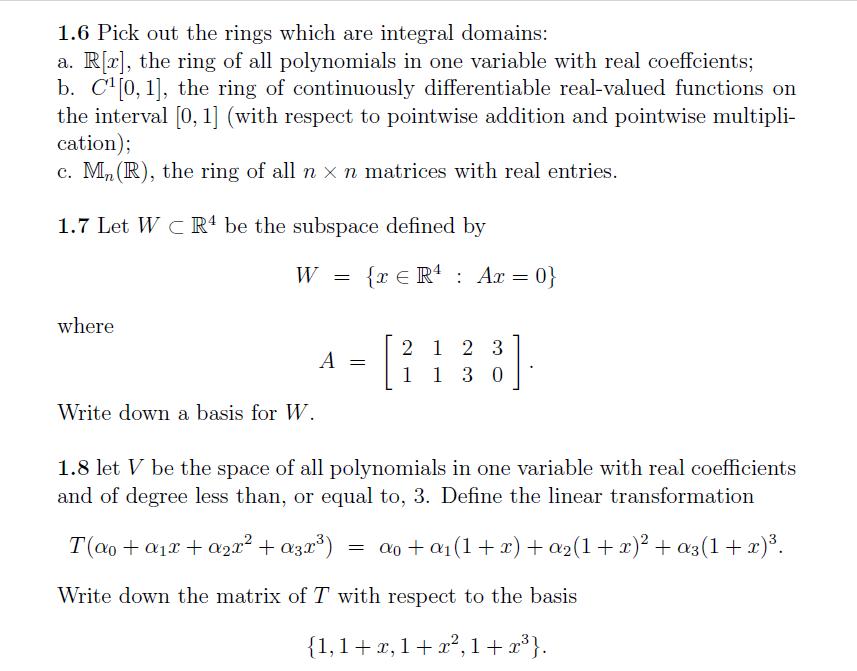
1.4 Pick out the pairs which are conjugate to each other in the respective groups: a. b. [1] and [] in GL2(R); 0 :] 0 1 C. 0 and in SL2(R); 0 1 1 0 in GL2(R). 02 3 [12] and [12] 02 1.5 Let R be a (commutative) ring (with identity). Let I and J be ideals in R. Pick out the true statements: a. IUJ is an ideal in R; b. In is an ideal in R; C. is an ideal in R. I+J = {x+yxel, y = J} 1.6 Pick out the rings which are integral domains: a. R[x], the ring of all polynomials in one variable with real coeffcients; b. C[0, 1], the ring of continuously differentiable real-valued functions on the interval [0, 1] (with respect to pointwise addition and pointwise multipli- cation); c. Mn(R), the ring of all n x n matrices with real entries. 1.7 Let WCR4 be the subspace defined by where W = {x R4 Ax = 0} A = 2 1 2 3 1 1 3 0 Write down a basis for W. 1.8 let V be the space of all polynomials in one variable with real coefficients and of degree less than, or equal to, 3. Define the linear transformation T(ao+a1x+a2x + 3x) = ao+a1(1+x) + a2(1+x) + 3 (1+x). Write down the matrix of T with respect to the basis {1, 1+x, 1+x2, 1+x}.