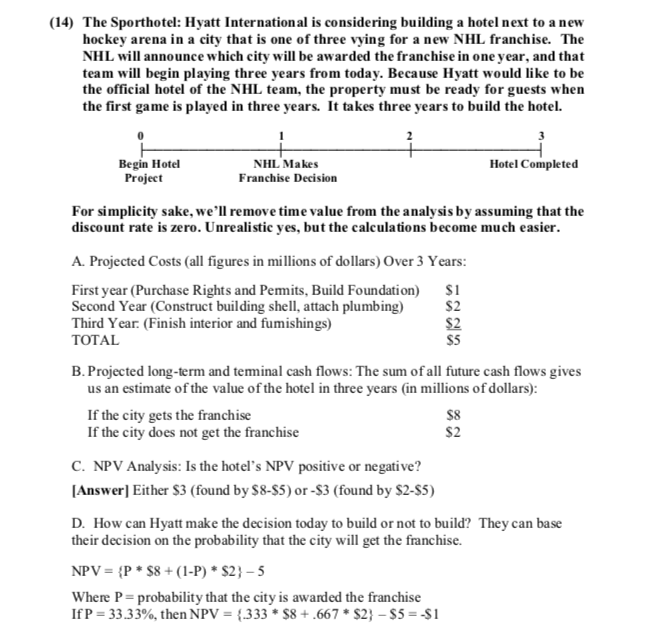
(14) The Sporthotel: Hyatt International is considering building a hotel next to a new hockey arena in a city that is one of three vying for a new NHL franchise. The NHL will announce which city will be awarded the franchise in one year, and that team will begin playing three years from today. Because Hyatt would like to be the official hotel of the NHL team, the property must be ready for guests when the first game is played in three years. It takes three years to build the hotel. Begin Hotel Project NHL Makes Franchise Decision Hotel Completed For simplicity sake, we'll remove time value from the analysis by assuming that the discount rate is zero. Unrealistic yes, but the calculations become much easier. A. Projected Costs (all figures in millions of dollars) Over 3 Years: $1 S2 First year (Purchase Rights and Pemits, Build Foundation) Second Year (Construct building shell, attach plumbing) Third Year: (Finish interior and fumishings) TOTAL B. Projected long-term and terminal cash flows: The sum of all future cash flows gives us an estimate of the value of the hotel in three years (in millions of dollars): S8 If the city gets the franchise If the city does not get the franchise C. NPV Analysis: Is the hotel's NPV positive or negative? [Answer) Either $3 (found by $8-$5) or -$3 (found by $2-$5) D. How can Hyatt make the decision today to build or not to build? They can base their decision on the probability that the city will get the franchise. NPV = {P * $8 + (1-P) * $2} - 5 Where P=probability that the city is awarded the franchise If P = 33.33%, then NPV = {333 * $8+.667 * $2} - $5=$1 If P=66.67%, then NPV = .667 * $8 + .333 * $2} -$5 = $1 If P = 50.00%, then NPV = {.500 * $8 +.500 * $2} -$5 = $0 E. We can also solve for the value of P that makes NPV = $0 P* ($8 - $5) + (1-P) * ($2 - $5)= $0 P*(3) + (1-P)*(-3)=0 3P - 3 + 3P = 0 6P = 3 and so P = 3/6 = 50% F. Conclusion from NPV Analysis: If Hyatt believes that the chances of being awarded the franchise are greater than 50%, it would be wise to build the hotel. If on the other hand their analysis leads them to believe that the chance of being awarded the franchise is less than 50%, they should reject the Sport Hotel. If they believe that the probability is exactly 50%, they should be indifferent. (16) The Sporthotel - Real Option Analysis: Suppose that our NPV analysis incorporates the right to abandon the hotel project should the city get bad news. Including abandonment, what probability of the city being awarded the franchise will make this project have a zero NPV? [Answer the probability falls to 25%. Here's how the abandonment option works. First, pay $1 million for all first year expenses to acquire the rights and plans and build the foundation. If after that first year the franchise is granted, then continue to build the hotel as it has a $3M NPV. On the other hand, if after one year the franchise is denied, Hyatt should abandon the hotel for a total loss of $1 million. The ability to abandon the project in the event of bad news is the "real option" in this project. Including the real option, what chance of getting the franchise would make NPV equal to zero? Since being awarded the franchise results in an NPV of $3 million, and abandoning the project if the franchise is not awarded results in an NPV of-$1, then: P* ($8 - $5) + (1-P) * (-$1) - $0 P*3 + (1-P)*- 1 = 0 3P-1 +P=0 4P = 1 and so P = /4 = 25% Thus, a franchise probability between 25% and 49% that was too low in the static NPV case is now sufficient to generate a positive NPV. Consider again the Sport Hotel example and Example 9.1. Suppose that if the franchise is accepted the value of the hotel is not $8 million but instead $8.50. Everything else, including first year expenses, is the same as shown in the example. Incorporating the real option, what probability of the franchise being granted would represent a "fair investment?" (that is, a probability such that any higher value would create a positive expected value) Place your answer in percentage form with at least 2 decimal places. For example, and answer of fifteen point four three percent would be entered 15.43. CHECK ANSWER (14) The Sporthotel: Hyatt International is considering building a hotel next to a new hockey arena in a city that is one of three vying for a new NHL franchise. The NHL will announce which city will be awarded the franchise in one year, and that team will begin playing three years from today. Because Hyatt would like to be the official hotel of the NHL team, the property must be ready for guests when the first game is played in three years. It takes three years to build the hotel. Begin Hotel Project NHL Makes Franchise Decision Hotel Completed For simplicity sake, we'll remove time value from the analysis by assuming that the discount rate is zero. Unrealistic yes, but the calculations become much easier. A. Projected Costs (all figures in millions of dollars) Over 3 Years: $1 S2 First year (Purchase Rights and Pemits, Build Foundation) Second Year (Construct building shell, attach plumbing) Third Year: (Finish interior and fumishings) TOTAL B. Projected long-term and terminal cash flows: The sum of all future cash flows gives us an estimate of the value of the hotel in three years (in millions of dollars): S8 If the city gets the franchise If the city does not get the franchise C. NPV Analysis: Is the hotel's NPV positive or negative? [Answer) Either $3 (found by $8-$5) or -$3 (found by $2-$5) D. How can Hyatt make the decision today to build or not to build? They can base their decision on the probability that the city will get the franchise. NPV = {P * $8 + (1-P) * $2} - 5 Where P=probability that the city is awarded the franchise If P = 33.33%, then NPV = {333 * $8+.667 * $2} - $5=$1 If P=66.67%, then NPV = .667 * $8 + .333 * $2} -$5 = $1 If P = 50.00%, then NPV = {.500 * $8 +.500 * $2} -$5 = $0 E. We can also solve for the value of P that makes NPV = $0 P* ($8 - $5) + (1-P) * ($2 - $5)= $0 P*(3) + (1-P)*(-3)=0 3P - 3 + 3P = 0 6P = 3 and so P = 3/6 = 50% F. Conclusion from NPV Analysis: If Hyatt believes that the chances of being awarded the franchise are greater than 50%, it would be wise to build the hotel. If on the other hand their analysis leads them to believe that the chance of being awarded the franchise is less than 50%, they should reject the Sport Hotel. If they believe that the probability is exactly 50%, they should be indifferent. (16) The Sporthotel - Real Option Analysis: Suppose that our NPV analysis incorporates the right to abandon the hotel project should the city get bad news. Including abandonment, what probability of the city being awarded the franchise will make this project have a zero NPV? [Answer the probability falls to 25%. Here's how the abandonment option works. First, pay $1 million for all first year expenses to acquire the rights and plans and build the foundation. If after that first year the franchise is granted, then continue to build the hotel as it has a $3M NPV. On the other hand, if after one year the franchise is denied, Hyatt should abandon the hotel for a total loss of $1 million. The ability to abandon the project in the event of bad news is the "real option" in this project. Including the real option, what chance of getting the franchise would make NPV equal to zero? Since being awarded the franchise results in an NPV of $3 million, and abandoning the project if the franchise is not awarded results in an NPV of-$1, then: P* ($8 - $5) + (1-P) * (-$1) - $0 P*3 + (1-P)*- 1 = 0 3P-1 +P=0 4P = 1 and so P = /4 = 25% Thus, a franchise probability between 25% and 49% that was too low in the static NPV case is now sufficient to generate a positive NPV. Consider again the Sport Hotel example and Example 9.1. Suppose that if the franchise is accepted the value of the hotel is not $8 million but instead $8.50. Everything else, including first year expenses, is the same as shown in the example. Incorporating the real option, what probability of the franchise being granted would represent a "fair investment?" (that is, a probability such that any higher value would create a positive expected value) Place your answer in percentage form with at least 2 decimal places. For example, and answer of fifteen point four three percent would be entered 15.43. CHECK