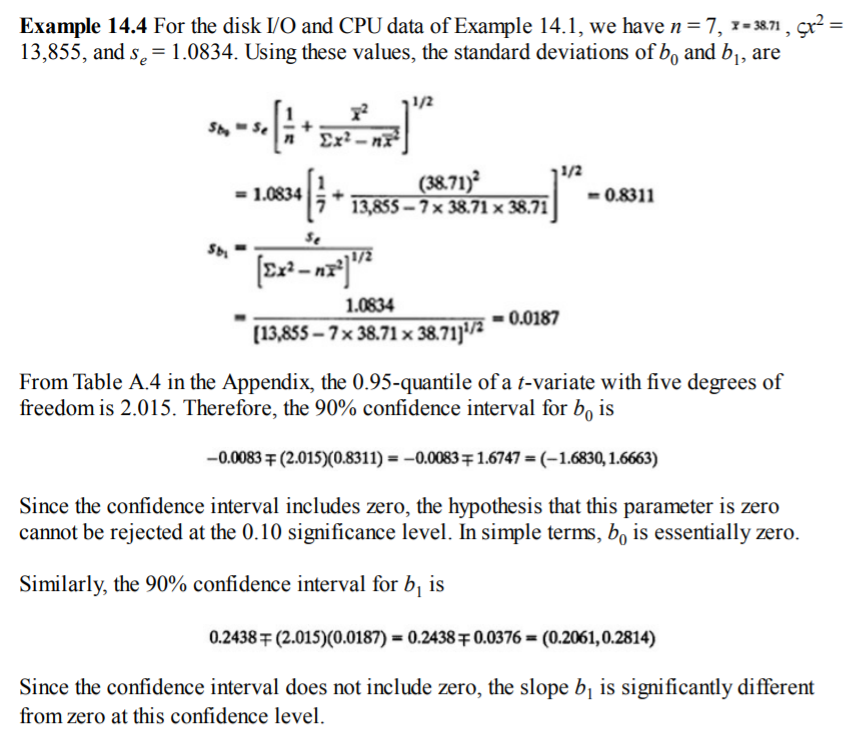
14.4 The designers of a database information system that allows its users to search backward for several days wanted to develop a formula to predict the time it would take to search. Actual elapsed time was measured for several different values of days. The measured data is shown in Table 14.4. Prepare a simple regression model for this data to predict elapsed time as a function of the number of days and interpret results. Example 14.4 For the disk I/O and CPU data of Example 14.1, we have n=7, += 38.71, x? = 855, and se = 1.0834. Using these values, the standard deviations of b, and b, are 1/2 (3871) 855 - 7x 38.71 x 38.71 -0.8311 1.0834 w2 -0.0187 (13,855 - 7x 38.71 x 38.71)/2 From Table A.4 in the Appendix, the 0.95-quantile of a t-variate with five degrees of freedom is 2.015. Therefore, the 90% confidence interval for b, is -0.0083 F (2.015)(0.8311) = -0.0083 7 1.6747 = (-1.6830, 1.6663) Since the confidence interval includes zero, the hypothesis that this parameter is zero cannot be rejected at the 0.10 significance level. In simple terms, b, is essentially zero. Similarly, the 90% confidence interval for b, is 0.2438 F (2.015)(0.0187) = 0.2438 +0.0376 = (0.2061,0.2814) Since the confidence interval does not include zero, the slope b, is significantly different from zero at this confidence level. 14.4 The designers of a database information system that allows its users to search backward for several days wanted to develop a formula to predict the time it would take to search. Actual elapsed time was measured for several different values of days. The measured data is shown in Table 14.4. Prepare a simple regression model for this data to predict elapsed time as a function of the number of days and interpret results. Example 14.4 For the disk I/O and CPU data of Example 14.1, we have n=7, += 38.71, x? = 855, and se = 1.0834. Using these values, the standard deviations of b, and b, are 1/2 (3871) 855 - 7x 38.71 x 38.71 -0.8311 1.0834 w2 -0.0187 (13,855 - 7x 38.71 x 38.71)/2 From Table A.4 in the Appendix, the 0.95-quantile of a t-variate with five degrees of freedom is 2.015. Therefore, the 90% confidence interval for b, is -0.0083 F (2.015)(0.8311) = -0.0083 7 1.6747 = (-1.6830, 1.6663) Since the confidence interval includes zero, the hypothesis that this parameter is zero cannot be rejected at the 0.10 significance level. In simple terms, b, is essentially zero. Similarly, the 90% confidence interval for b, is 0.2438 F (2.015)(0.0187) = 0.2438 +0.0376 = (0.2061,0.2814) Since the confidence interval does not include zero, the slope b, is significantly different from zero at this confidence level