Question
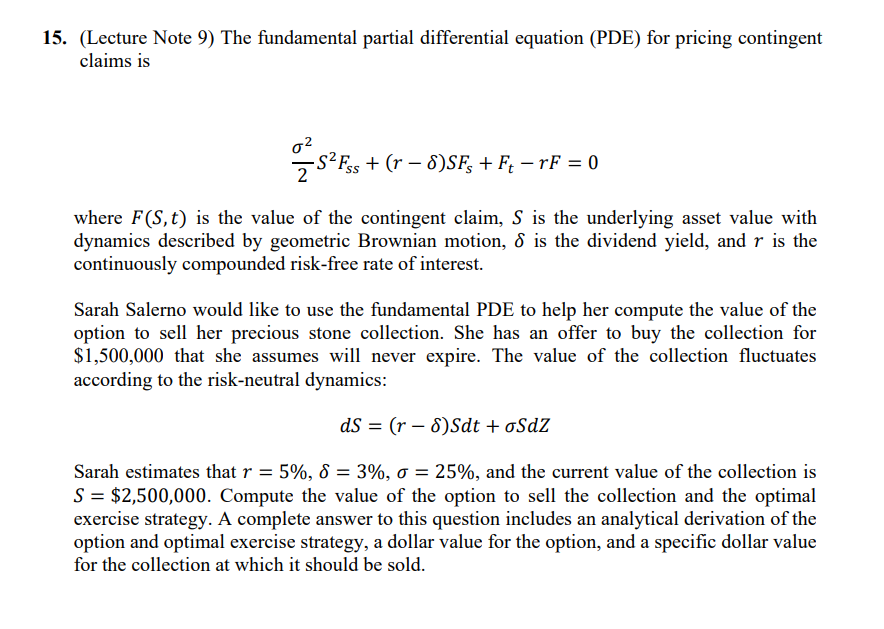
15. (Lecture Note 9) The fundamental partial differential equation (PDE) for pricing contingent claims is 9 2 2 2 + ( ) + = 0
15. (Lecture Note 9) The fundamental partial differential equation (PDE) for pricing contingent claims is 9 2 2 2 + ( ) + = 0 where (,) is the value of the contingent claim, is the underlying asset value with dynamics described by geometric Brownian motion, is the dividend yield, and is the continuously compounded risk-free rate of interest. Sarah Salerno would like to use the fundamental PDE to help her compute the value of the option to sell her precious stone collection. She has an offer to buy the collection for $1,500,000 that she assumes will never expire. The value of the collection fluctuates according to the risk-neutral dynamics: = ( ) + Sarah estimates that = 5%, = 3%, = 25%, and the current value of the collection is = $2,500,000. Compute the value of the option to sell the collection and the optimal exercise strategy. A complete answer to this question includes an analytical derivation of the option and optimal exercise strategy, a dollar value for the option, and a specific dollar value for the collection at which it should be sold.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


