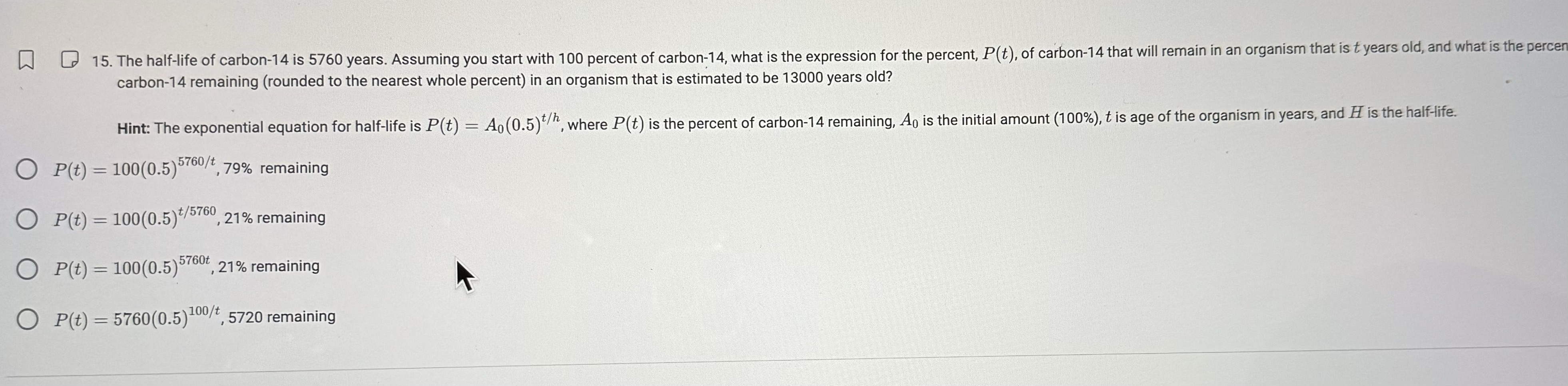
15. The half-life of carbon-14 is 5760 years. Assuming you start with 100 percent of carbon-14, what is the expression for the percent, P(t), of carbon-14 that will remain in an organism that is t years old, and what is the percen carbon-14 remaining (rounded to the nearest whole percent) in an organism that is estimated to be 13000 years old? Hint: The exponential equation for half-life is P(t) = A0 (0.5)t/h, where P(t) is the percent of carbon-14 remaining, A is the initial amount (100%), t is age of the organism in years, and H is the half-life. OP(t)=100(0.5)5 P(t) = 100(0.5)5760/t 79% remaining 100(0.5)*/5760,21% remaining OP(t)=100(0.5)* OP(t)=100(0.5) 5760, 21% remaining OP(t)=5760(0.5) 100/+, 5720 remaining