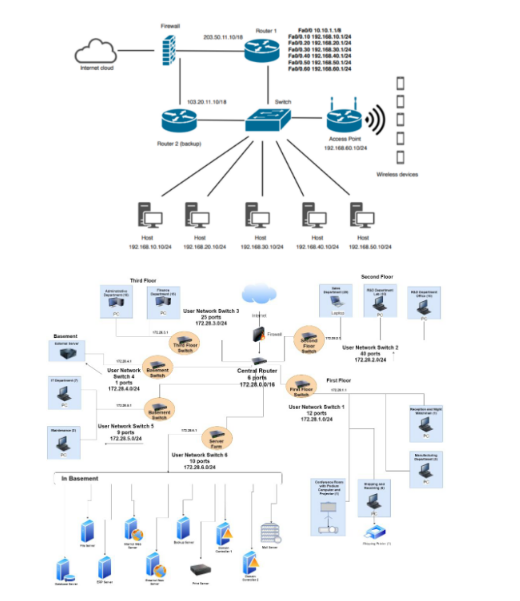
192.168.0.10/24 Basement User Network 172.28.4024 User Network Switch 5 3 ports 172.28.5.0/24 200.50 11.10/18 103 20.11.1918 User Network Switch 3 25 ports 172.28.3624 13 ports 172.30.0.0:34 180168 30 1024 Central Router ports 172.26.0.0/16 192.168.0.10/24192.168.0.10/24 Wireless devices User Network Switch 12 ports 172.28.1.0/24 OOOOO Second Floor UN Switch 2 40 ports 172.22.024 (20 points) Let S[n] be an array of n positive integers. We would like to design an efficient algorithm that returns false if there is at least one (non-empty) subset of numbers T from S[n] where the total sum of the elements in T is less than |T|: Otherwise, the algorithm should return true. For example, if S = [7,3, 11, 4] then the algorithm should return false since for the subset T = {3,4} : 3+4 < 2. On the other hand, if S = [15, 3, 16] then the algorithm should return true because none of the subsets of S satisfies the above inequality (1): For the subset T ={15}: For the subset T = {3}: For the subset T = {16}: For the subset T = {15,3}: For the subset T = {3,16}: For the subset T = {15,16}: For the subset T = {15,3, 16}: 151 31 161 t