1.In this problem, assume that the distribution of differences is approximately normal. Note: For degrees of freedom d.f. not in the Student's t table, use the closest d.f. that is smaller. In some situations, this choice of d.f. may increase the P-value by a small amount and therefore produce a slightly more "conservative" answer.

Are America's top chief executive officers (CEOs) really worth all that money? One way to answer this question is to look at row B, the annual company percentage increase in revenue, versus row A, the CEO's annual percentage salary increase in that same company. Suppose a random sample of companies yielded the following data:

B: Percent increase 24 21 27 18 21 37 for company A: Percent increase 21 23 27 14 -4 15 30 for CEO Do these data indicate that the population mean percentage increase in corporate revenue (row B) is different from the population mean percentage increase in CEO salary? Use a 5% level of significance. (Let d = B - A.) (a) What is the level of significance? State the null and alternate hypotheses. O Ho: Hg > 0; H1: Hd = 0 O Ho: Hd = 0; H1: Hd = 0 O Ho: Hg = 0; H1: Hd = 0 O Ho: Hd = 0; H1: Hd >0 O Ho: Hd = 0; H1: Hd 0.500 0.250 a, we reject Ho. The data are not statistically significant. O Since the P-value > a, we fail to reject Ho. The data are not statistically significant. O Since the P-value s a, we fail to reject Ho. The data are statistically significant. (e) Interpret your conclusion in the context of the application. O Fail to reject Ho. At the 5% level of significance, the evidence is insufficient to claim a difference in population mean percentage increases for corporate revenue and CEO salary. O Fail to reject Ho. At the 5% level of significance, the evidence is sufficient to claim a difference in population mean percentage increases for corporate revenue and CEO salary. O Reject Ho. At the 5% level of significance, the evidence is insufficient to claim a difference in population mean percentage increases for corporate revenue and CEO salary. O Reject Ho. At the 5% level of significance, the evidence is sufficient to claim a difference in population mean percentage increases for corporate revenue and CEO salary.A random sample of n1 = 10 regions in New England gave the following vio ent crime rates {per million population). x1: New England Crime Rate 3.3 3.9 4.2 4.1 3.3 4.1 1.8 4.8 2.9 3.1 Another random sample ofn2 = 12 regions in the Rocky Mountain states gave the following violent crime rates (per million population). x2: Rocky Mountain Crime Rate 3.7 4.1 4.7 5.5 3.3 4.8 3.5 2.4 3.1 3.5 5.2 2.8 lg use SALT Assume that the crime rate distribution is approximately normal in both regions. (a) Use a calculator to calculate E1, 51, E2, and 52. {Round your answers to four deCImal places.) (b) Do the data indicate that the violent crime rate in the Rocky Mountain region is higher than in New England? Use a = 0.01. (i) What is the level of signicance? State the null and alternate hypotheses. 0 Ho: 1'1 = \"2: H1: \"1 1 \"2 O HO: -\"1 _u2 (ii) What sampling distribution will you use? What assumptions are you making? 0 The standard normal. We assume that both population distributions are approximately normal with known standard deviations. O The Student's t. We assume that both population distributions are approximately normal with unknown standard deviations. O The Student's t. We assume that both population distributions are approximately normal with known standard deviations. O The standard normal. We assume that both population distributions are approximately normal with unknown standard deviations. What is the value of the sample test statistic? (Test the difference #1 - (2. Round your answer to three decimal places.) (iii) Find (or estimate) the P-value. O P-value > 0.250 O 0.125 P2 (b) What sampling distribution will you use? What assumptions are you making? O The Student's t. The number of trials is sufficiently large. O The standard normal. The number of trials is sufficiently large. O The standard normal. We assume the population distributions are approximately normal. O The Student's t. We assume the population distributions are approximately normal. What is the value of the sample test statistic? (Test the difference p, - p2. Do not use rounded values. Round your final answer to two decimal places.) (c) Find (or estimate) the P-value. (Round your answer to four decimal places.)Sketch the sampling distribution and show the area corresponding to the P-value. a b O-3 -2 -1 0 1 2 3 O-3 -2 -1 0 1 2 3 C O-3 -2 -1 0 1 2 3 O-3 -2 -1 0 1 2 (d) Based on your answers in parts (a) to (c), will you reject or fail to reject the null hypothesis? Are the data statistically significant at level a? At the a = 0.05 level, we reject the null hypothesis and conclude the data are not statistically significant. O At the a = 0.05 level, we fail to reject the null hypothesis and conclude the data are not statistically significant. O At the a = 0.05 level, we fail to reject the null hypothesis and conclude the data are statistically significant. O At the a = 0.05 level, we reject the null hypothesis and conclude the data are statistically significant. (e) Interpret your conclusion in the context of the application. Fail to reject the null hypothesis, there is sufficient evidence that the proportion of conservative voters favoring more tax dollars for the arts is less than the proportion of moderate voters. O Fail to reject the null hypothesis, there is insufficient evidence that the proportion of conservative voters favoring more tax dollars for the arts is less than the proportion of moderate voters. O Reject the null hypothesis, there is insufficient evidence that the proportion of conservative voters favoring more tax dollars for the arts is less than the proportion of moderate voters. O Reject the null hypothesis, there is sufficient evidence that the proportion of conservative voters favoring more tax dollars for the arts is less than the proportion of moderate voters.Education influences attitude and lifestyle. Differences in education are a big factor in the "generation gap." Is the younger generation really better educated? Large surveys of people age 65 and older were taken in n, = 30 U.S. cities. The sample mean for these cities showed that x1 = 15.2% of the older adults had attended college. Large surveys of young adults (age 25 - 34) were taken in n2 = 34 U.S. cities. The sample mean for these cities showed that X2 = 19.7% of the young adults had attended college. From previous studies, it is known that 1 = 6.8% and 2 = 4.8%. Does this information indicate that the population mean percentage of young adults who attended college is higher? Use a = 0.05. (a) What is the level of significance? State the null and alternate hypotheses. O Ho: H1 = H2i H1: H1 = H2 O Ho: M1 = H2; H1: H1 * H2 O HO: M1

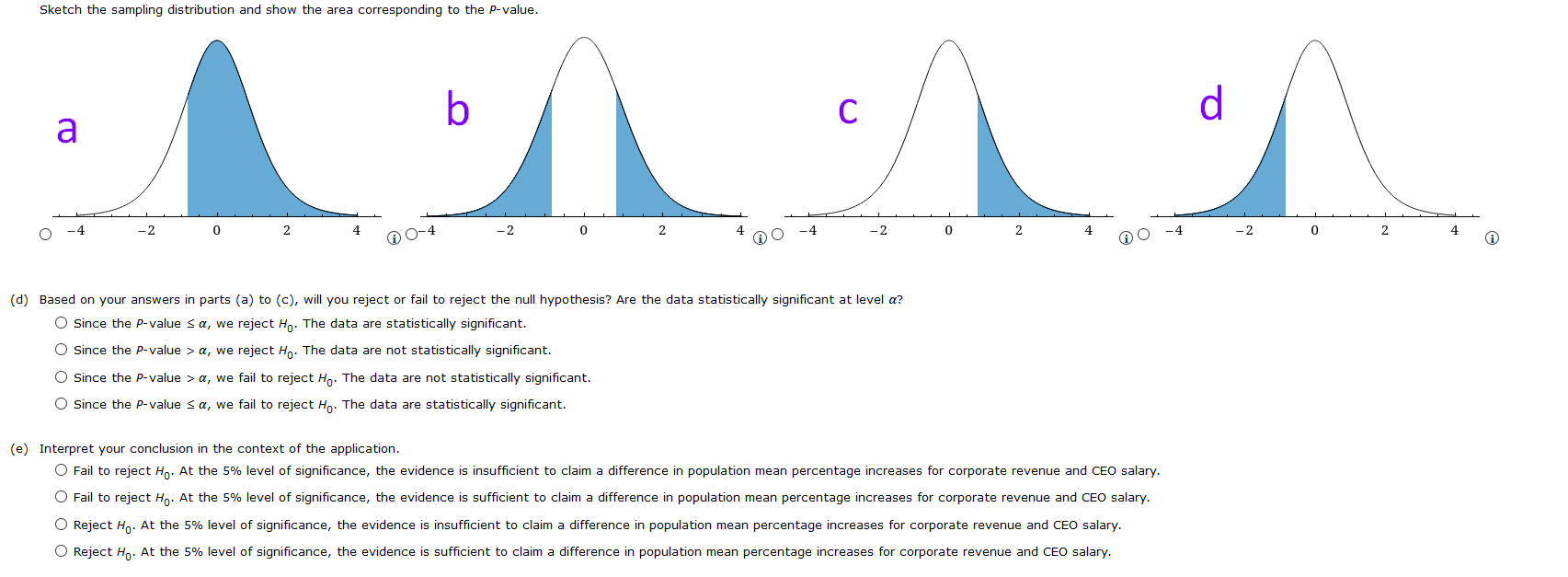
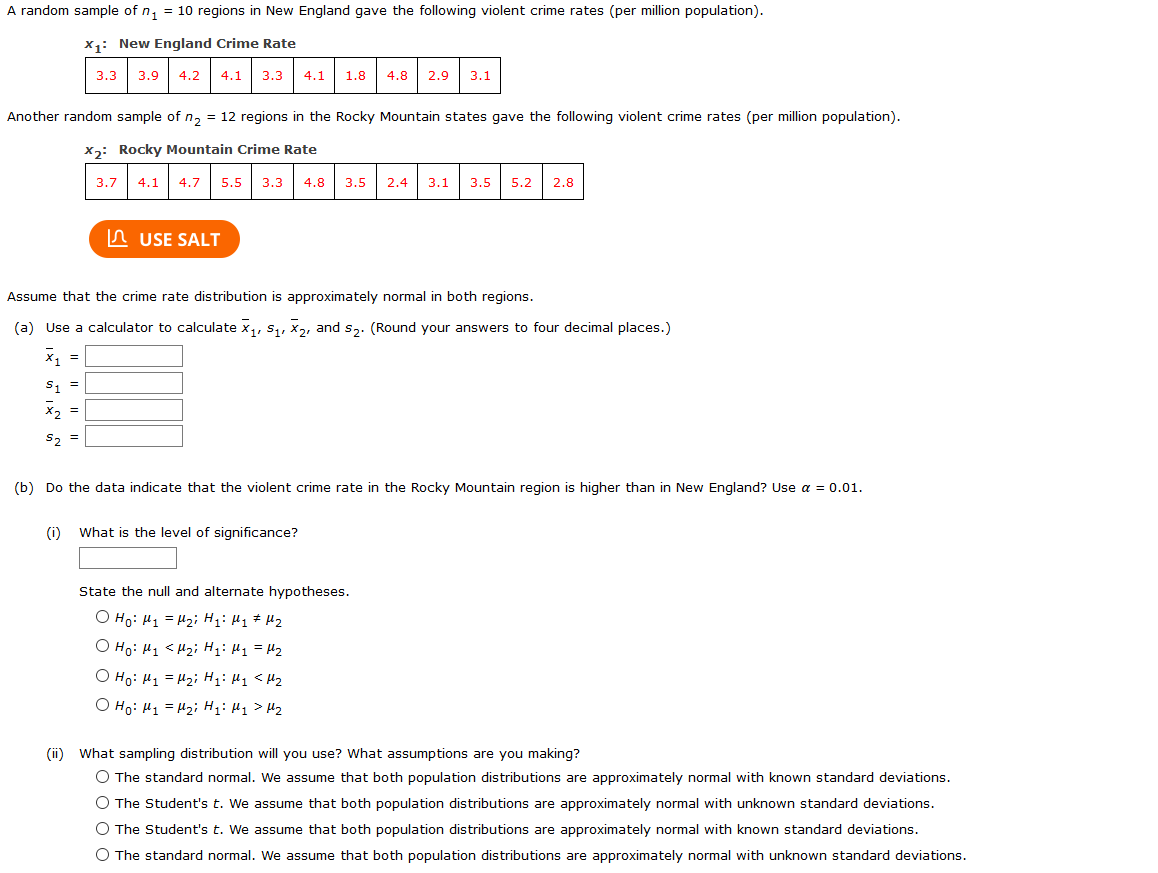
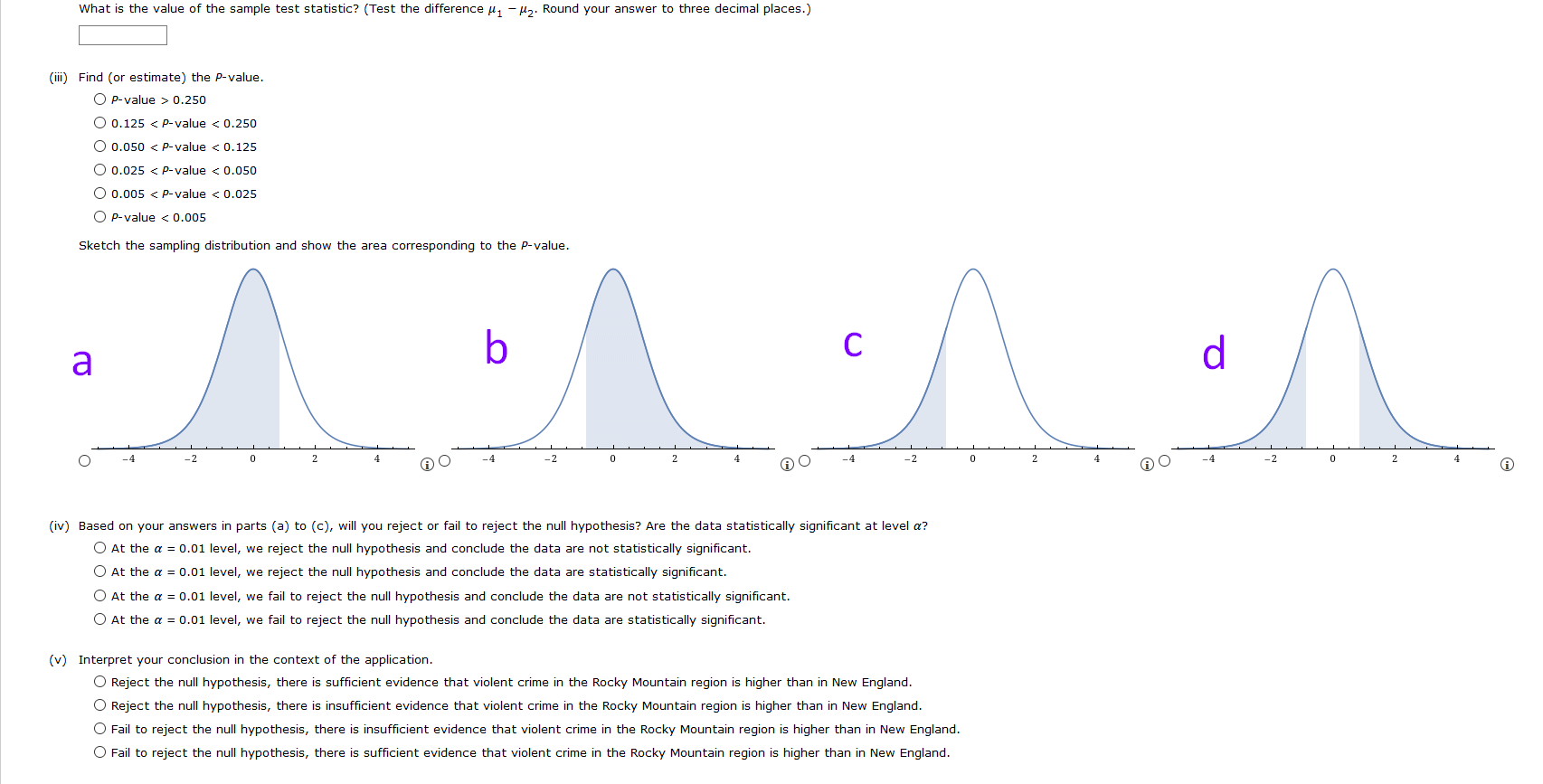
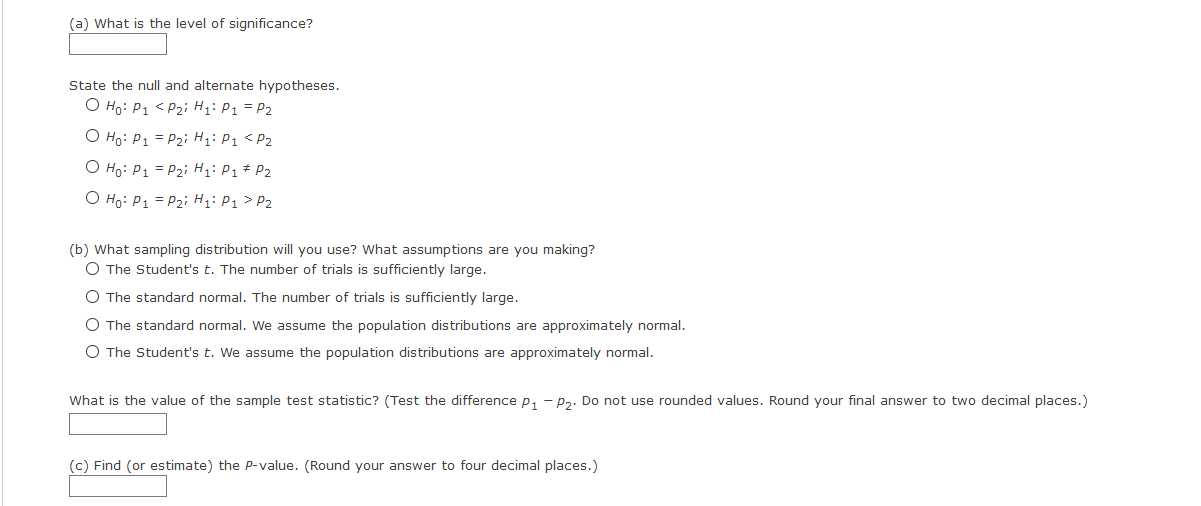
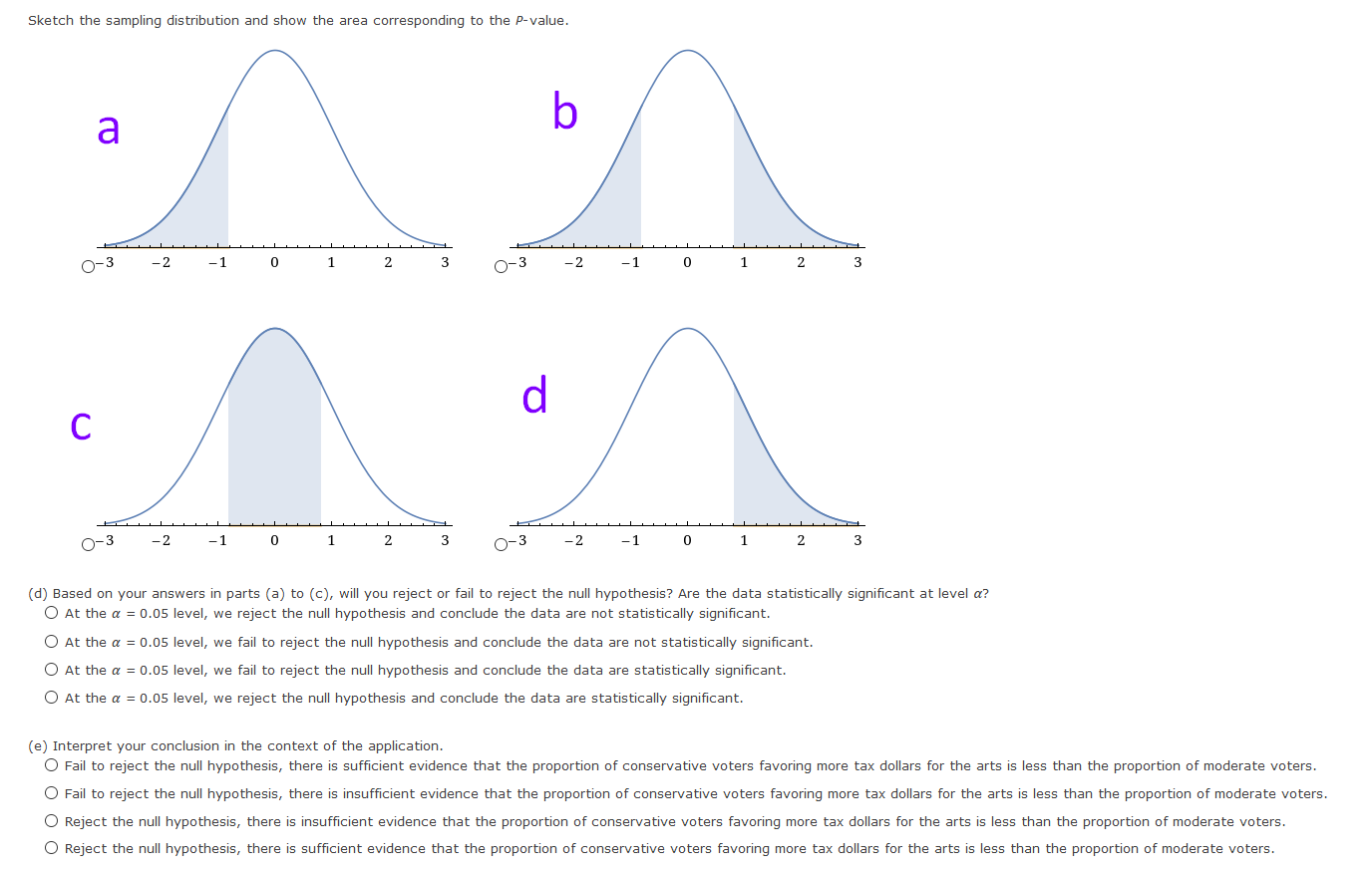
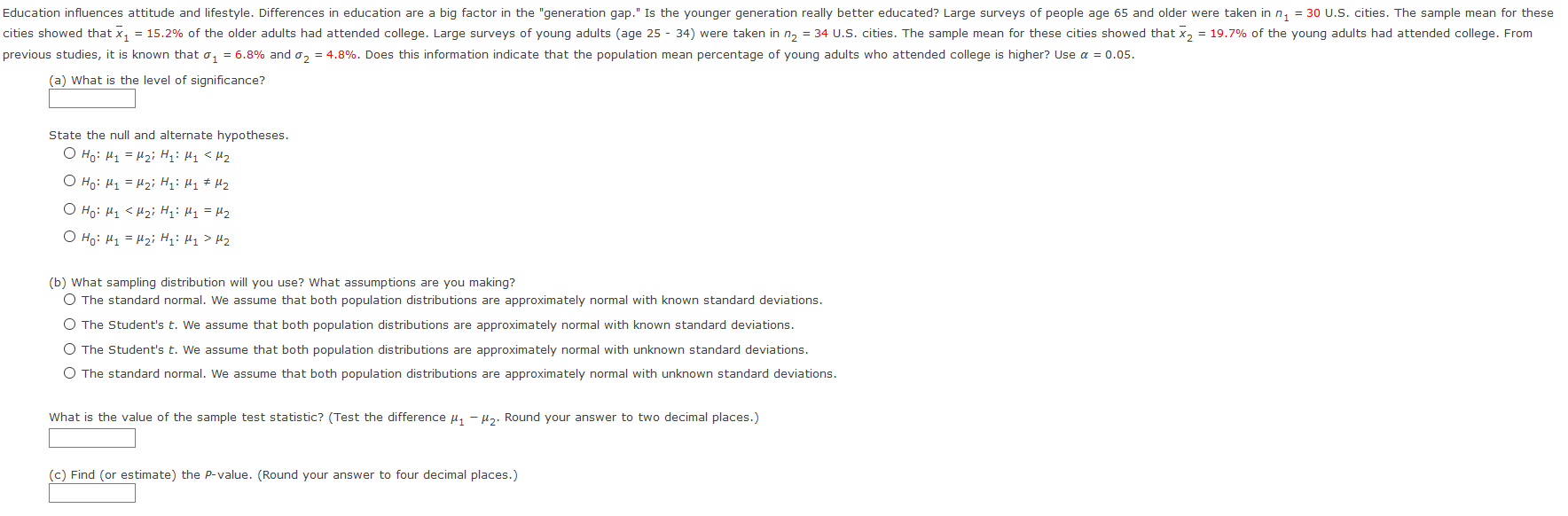
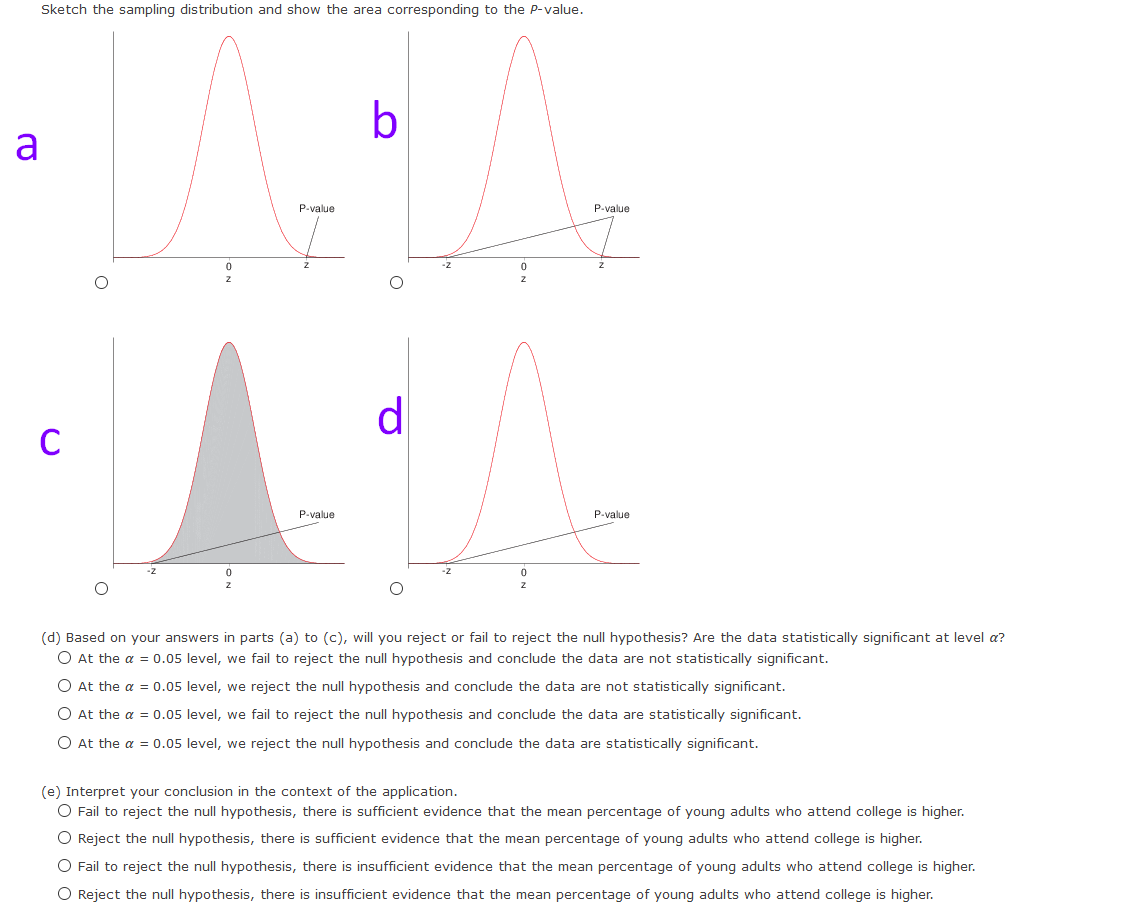
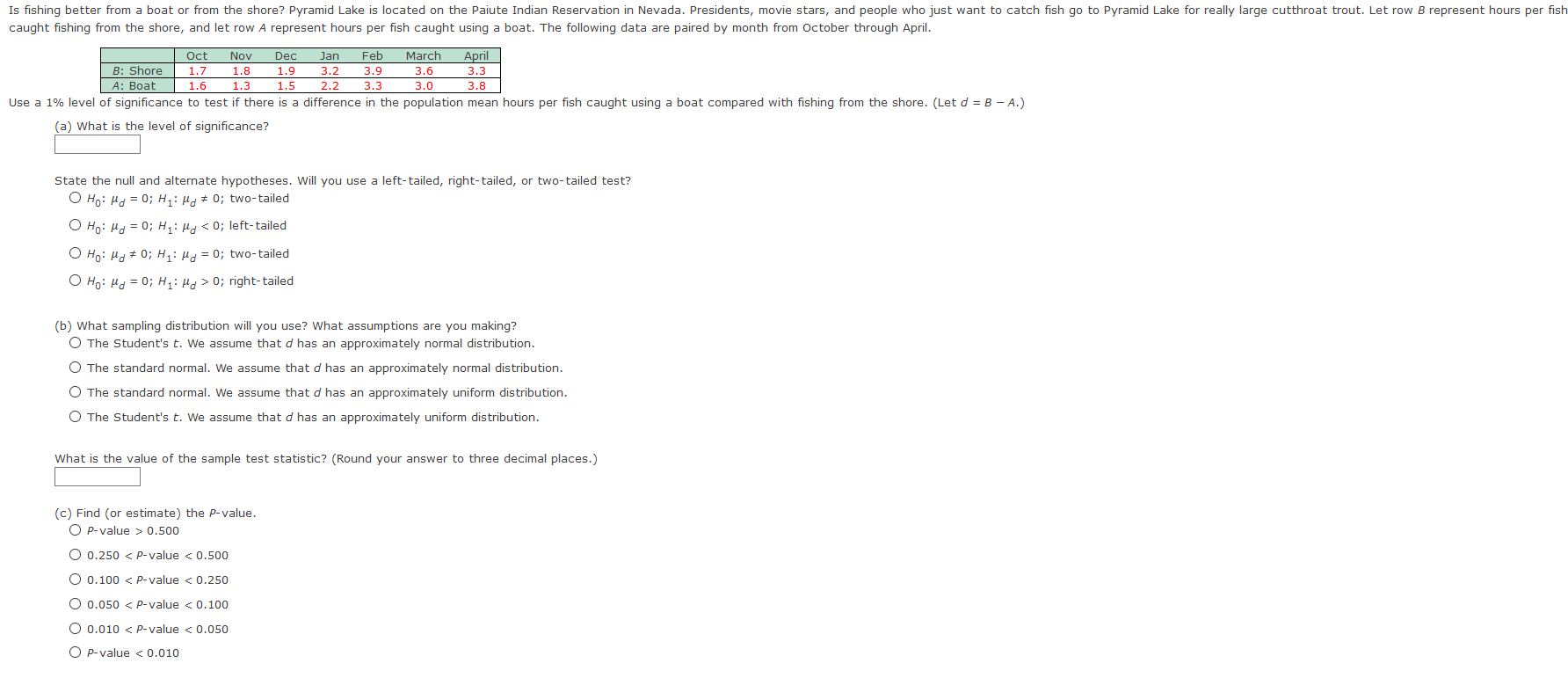
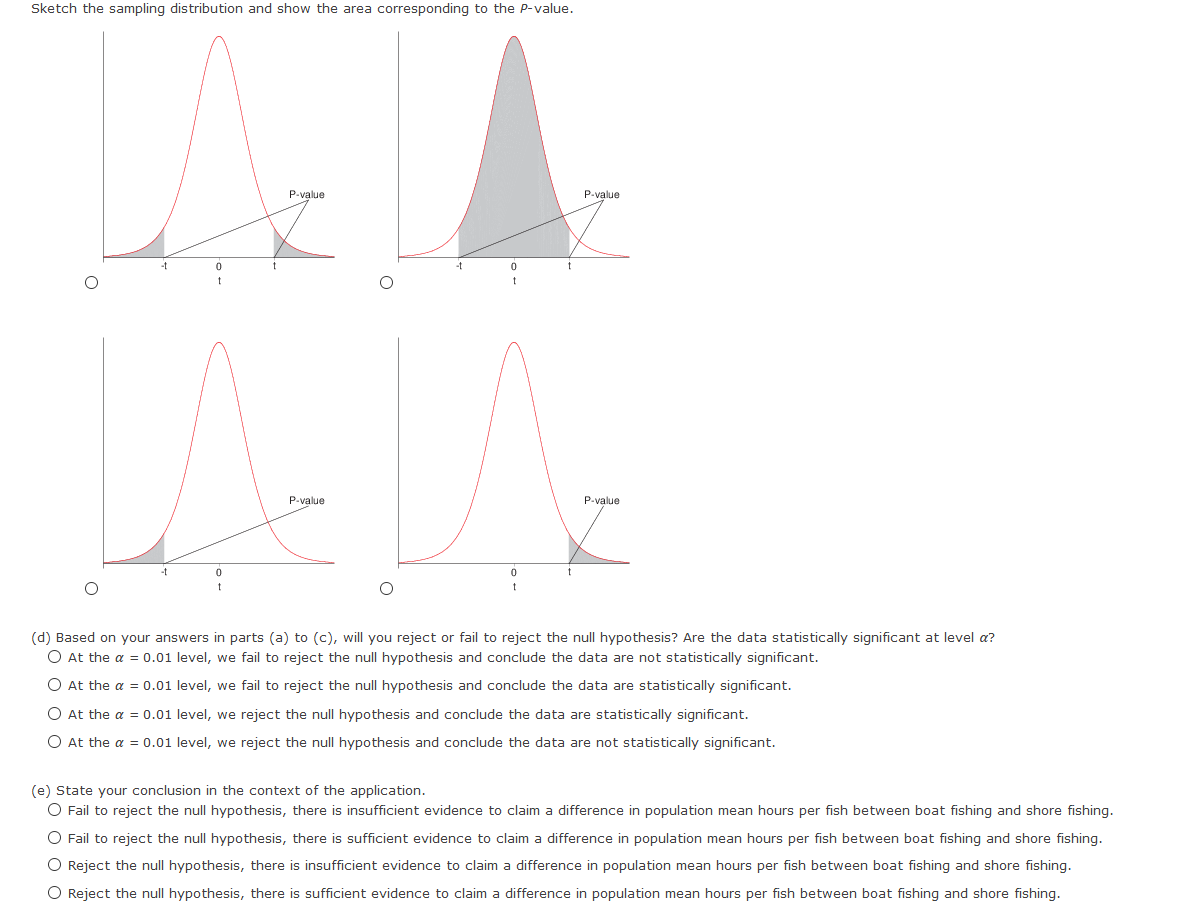
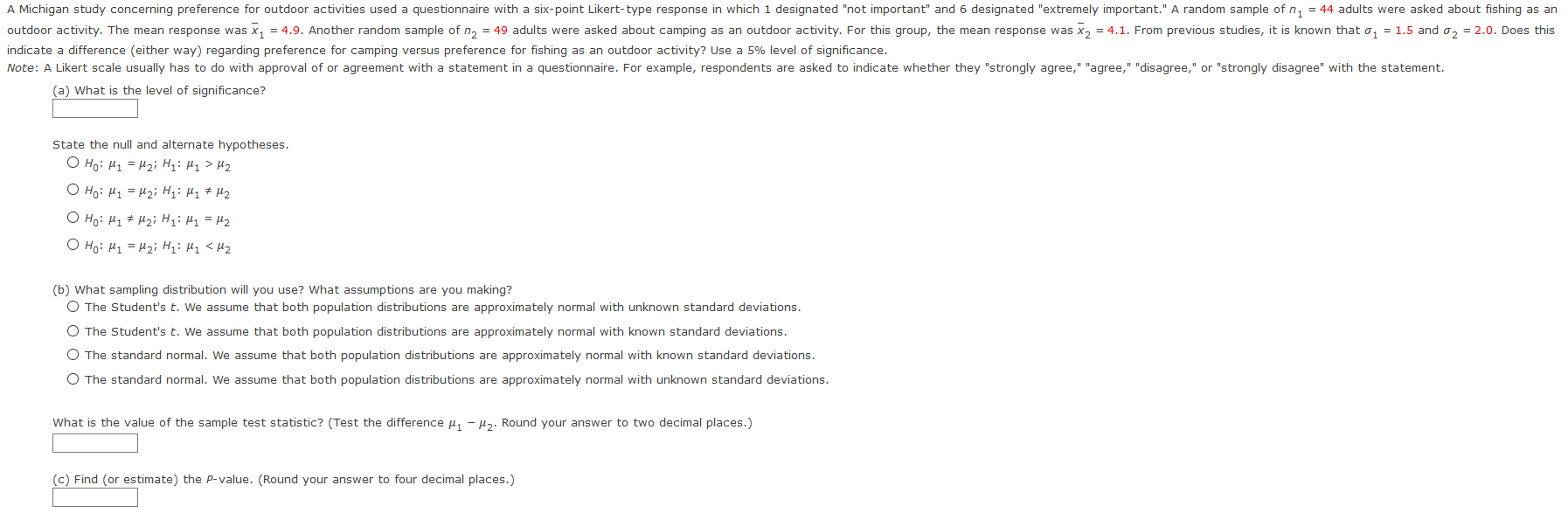
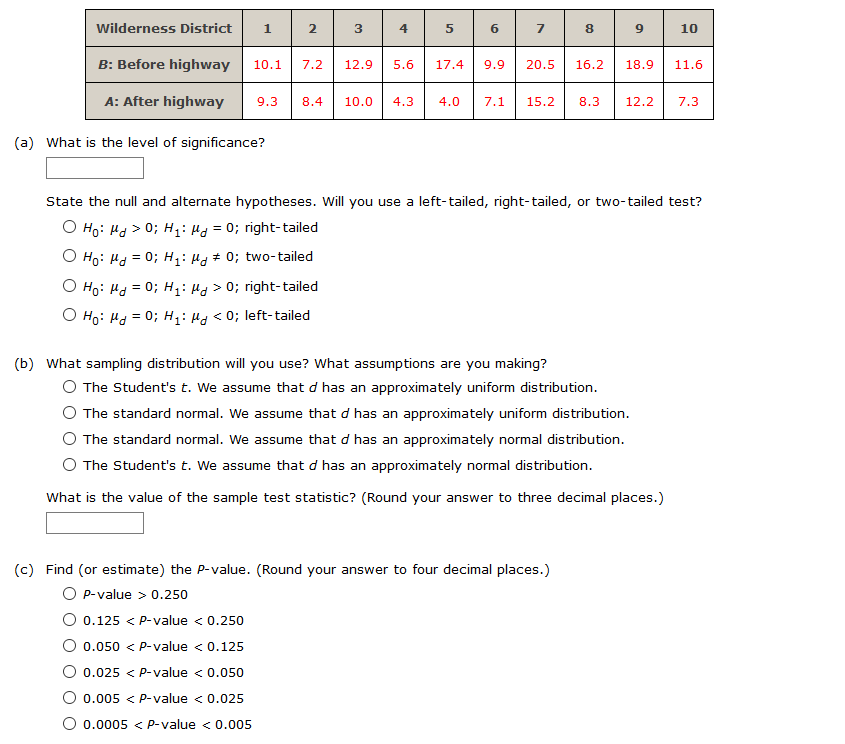
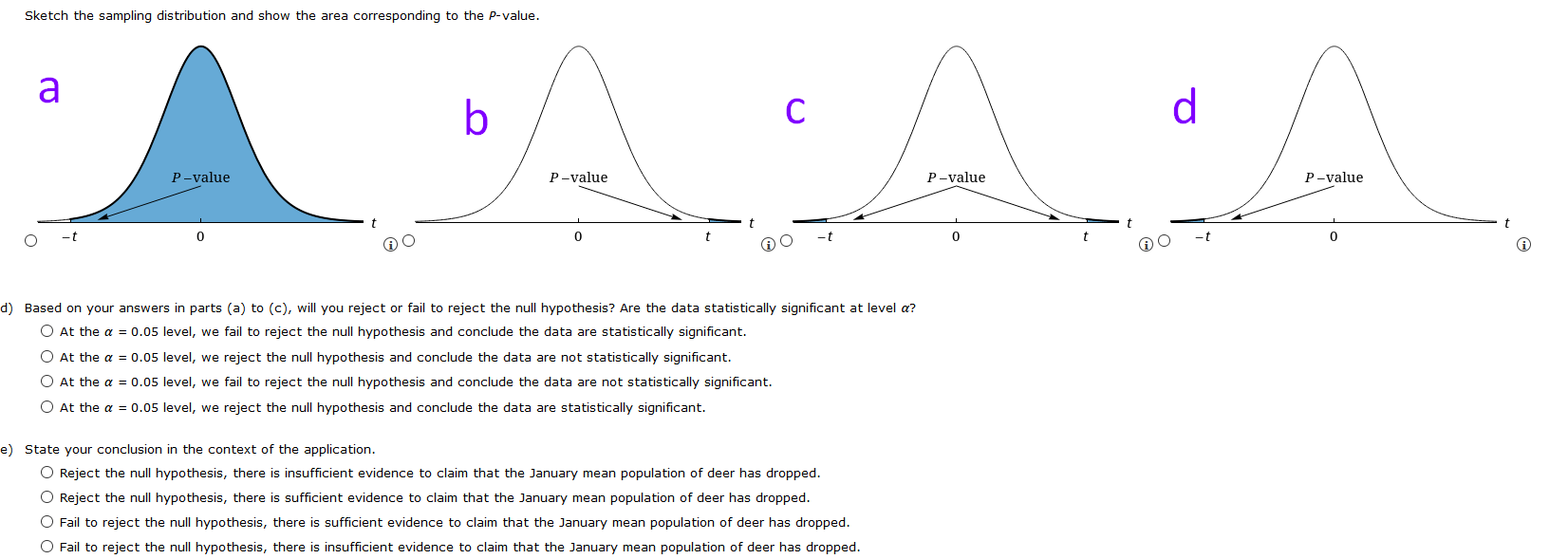
H2 (b) What sampling distribution will you use? What assumptions are you making? The standard normal. We assume that both population distributions are approximately normal with known standard deviations. O The Student's t. We assume that both population distributions are approximately normal with known standard deviations. O The Student's t. We assume that both population distributions are approximately normal with unknown standard deviations. O The standard normal. We assume that both population distributions are approximately normal with unknown standard deviations. What is the value of the sample test statistic? (Test the difference #1 - #2. Round your answer to two decimal places.) (c) Find (or estimate) the P-value. (Round your answer to four decimal places.)Sketch the sampling distribution and show the area corresponding to the P-value. b a P-value P-value -Z NO NO O O C P-value P-value NO NO (d) Based on your answers in parts (a) to (c), will you reject or fail to reject the null hypothesis? Are the data statistically significant at level a? O At the a = 0.05 level, we fail to reject the null hypothesis and conclude the data are not statistically significant. O At the a = 0.05 level, we reject the null hypothesis and conclude the data are not statistically significant. O At the a = 0.05 level, we fail to reject the null hypothesis and conclude the data are statistically significant. At the a = 0.05 level, we reject the null hypothesis and conclude the data are statistically significant. (e) Interpret your conclusion in the context of the application. O Fail to reject the null hypothesis, there is sufficient evidence that the mean percentage of young adults who attend college is higher. O Reject the null hypothesis, there is sufficient evidence that the mean percentage of young adults who attend college is higher. Fail to reject the null hypothesis, there is insufficient evidence that the mean percentage of young adults who attend college is higher. O Reject the null hypothesis, there is insufficient evidence that the mean percentage of young adults who attend college is higher.(a) Check Requirements: What distribution does the sample test statistic follow? Explain. O The standard normal. We assume that both population distributions are approximately normal with unknown standard deviations. The standard normal. We assume that both population distributions are approximately normal with known standard deviations. The Student's t. We assume that both population distributions are approximately normal with unknown standard deviations. O The Student's t. We assume that both population distributions are approximately normal with known standard deviations. (b) State the hypotheses. O Ho: H1 = #2i H1: H1 * Hz O Ho: H1 = #2i H1: H1 = Hz O Ho : H1 = #2; H1: H1 > Hz O Ho: H1 # Hzi H1: M1 = Hz (c) Compute X 1 - X2. * 1 - * 2 = Compute the corresponding sample distribution value. (Test the difference #1 - #2. Round your answer to two decimal places.) 'd) Find the P-value of the sample test statistic. (Round your answer to four decimal places.) (e) Conclude the test. O At the a = 0.01 level, we fail to reject the null hypothesis and conclude the data are not statistically significant. O At the a = 0.01 level, we reject the null hypothesis and conclude the data are not statistically significant. O At the a = 0.01 level, we fail to reject the null hypothesis and conclude the data are statistically significant. O At the a = 0.01 level, we reject the null hypothesis and conclude the data are statistically significant. (f) Interpret the results. O Fail to reject the null hypothesis, there is insufficient evidence that there is a difference between the population means. O Reject the null hypothesis, there is insufficient evidence that there is a difference between the population means. O Fail to reject the null hypothesis, there is sufficient evidence that there is a difference between the population means. O Reject the null hypothesis, there is sufficient evidence that there is a difference between the population means.Is fishing better from a boat or from the shore? Pyramid Lake is located on the Paiute Indian Reservation in Nevada. Presidents, movie stars, and people who just want to catch fish go to Pyramid Lake for really large cutthroat trout. Let row B represent hours per fish caught fishing from the shore, and let row A represent hours per fish caught using a boat. The following data are paired by month from October through April. Oct Nov Dec Jan Feb March April B: Shore 1.7 1.8 1.9 3.2 3.9 3.6 3.3 A: Boat 1.6 1.3 1.5 2.2 3.3 3.0 3.8 Use a 1% level of significance to test if there is a difference in the population mean hours per fish caught using a boat compared with fishing from the shore. (Let d = B - A.) (a) What is the level of significance? State the null and alternate hypotheses. Will you use a left-tailed, right-tailed, or two-tailed test? O Ho: Mg = 0; H1: My # 0; two-tailed O Ho: Mg = 0; H1: My 0; right- tailed (b) What sampling distribution will you use? What assumptions are you making? O The Student's t. We assume that d has an approximately normal distribution. O The standard normal. We assume that d has an approximately normal distribution. The standard normal. We assume that d has an approximately uniform distribution. O The Student's t. We assume that d has an approximately uniform distribution. What is the value of the sample test statistic? (Round your answer to three decimal places.) (c) Find (or estimate) the P-value. O P-value > 0.500 O 0.250 H2 O Ho: M1 = M2i H1: M1 * Hz O HO: H1 # Hzi H1: M1 = H2 O Ho: H1 = H2i H1: H1 = H2 (b) What sampling distribution will you use? What assumptions are you making? O The Student's t. We assume that both population distributions are approximately normal with unknown standard deviations. O The Student's t. We assume that both population distributions are approximately normal with known standard deviations. The standard normal. We assume that both population distributions are approximately normal with known standard deviations. The standard normal. We assume that both population distributions are approximately normal with unknown standard deviations. What is the value of the sample test statistic? (Test the difference uj - #2. Round your answer to two decimal places.) (c) Find (or estimate) the P-value. (Round your answer to four decimal places.)Sketch the sampling distribution and show the area corresponding to the P-value. a b O-3 -2 -1 0 1 2 3 O-3 -2 - 1 0 1 2 3 C O-3 -2 -1 2 O-3 -2 -1 0 1 2 (d) Based on your answers in parts (a) to (c), will you reject or fail to reject the null hypothesis? Are the data statistically significant at level a? O At the a = 0.05 level, we reject the null hypothesis and conclude the data are statistically significant. O At the a = 0.05 level, we fail to reject the null hypothesis and conclude the data are statistically significant. O At the a = 0.05 level, we reject the null hypothesis and conclude the data are not statistically significant. O At the a = 0.05 level, we fail to reject the null hypothesis and conclude the data are not statistically significant. (e) Interpret your conclusion in the context of the application. O Fail to reject the null hypothesis, there is sufficient evidence that there is a difference between mean response regarding preference for camping or fishing. O Reject the null hypothesis, there is insufficient evidence that there is a difference between mean response regarding preference for camping or fishing O Fail to reject the null hypothesis, there is insufficient evidence that there is a difference between mean response regarding preference for camping or fishing. O Reject the null hypothesis, there is sufficient evidence that there is a difference between mean response regarding preference for camping or fishing.Wilderness District 5 10 B: Before highway 10.1 7.2 12.9 5.6 17.4 9.9 20.5 16.2 18.9 11.6 A: After highway 9.3 8.4 10.0 7.3 4.0 7.1 15.2 8.3 12.2 7.3 (a) What is the level of significance? State the null and alternate hypotheses. Will you use a left-tailed, right-tailed, or two-tailed test? O Ho: My > 0; H1: My = 0; right- tailed O Ho: My = 0; H1: My # 0; two-tailed O Ho: My = 0; H1: My > 0; right- tailed O Ho: My = 0; H1: My 0.250 O 0.125