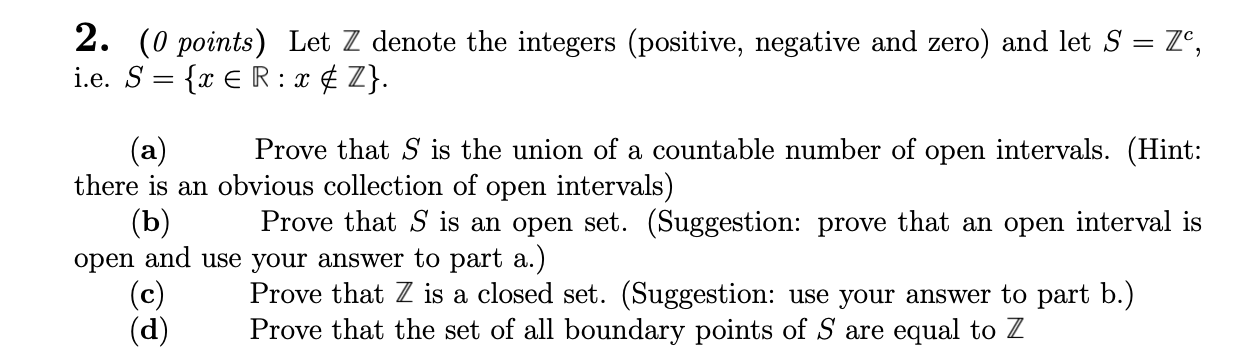
2. (0 points) Let Z denote the integers (positive, negative and zero) and let S = Z, i.e. S = {x R : x & Z}. (a) Prove that S is the union of a countable number of open intervals. (Hint: there is an obvious collection of open intervals) (b) Prove that S is an open set. (Suggestion: prove that an open interval is open and use your answer to part a.) (d) Prove that Z is a closed set. (Suggestion: use your answer to part b.) Prove that the set of all boundary points of S are equal to Z