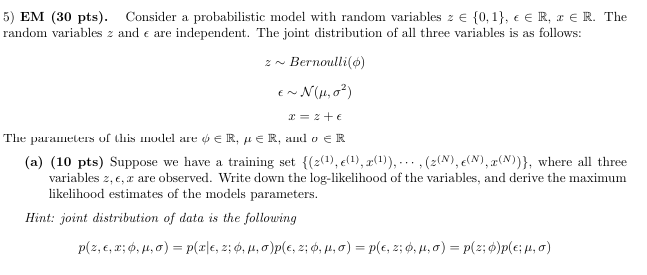
2 5) EM (30 pts). Consider a probabilistic model with random variables z = {0,1}, ER, 2 R. The random variables z and are independent. The joint distribution of all three variables is as follows: 2 ~ Bernoulli(6) E~N (1,0) x = x+ The parameters of this model are R, R, and o R (a) (10 pts) Suppose we have a training set {(2(1), e(), x(1)), , (2(N), (N), (N))}, where all three variables z, e, x are observed. Write down the log-likelihood of the variables, and derive the maximum likelihood estimates of the models parameters. Hint: joint distribution of data is the following p(, , x; ) = p(x|, 2; , , )p(, 2; , , ) = p(, 2; , ) = p(; )p(; , )