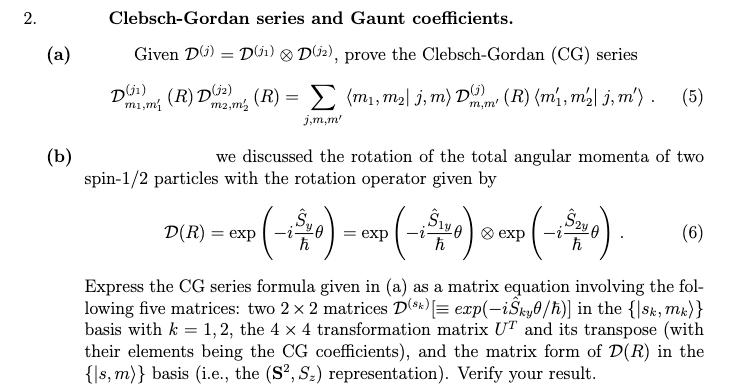
2. (a) (b) Clebsch-Gordan series and Gaunt coefficients. Given D)= D) D2), prove the Clebsch-Gordan (CG) series (R) D(2) DG) mi,m m2,m (R) = (m, m|j, m) Dom (R) (m, m|j, m'). (5) j,m,m' we discussed the rotation of the total angular momenta of two spin-1/2 particles with the rotation operator given by 1 (1820) - COT (SVO) CCP ( 1820). = exp exp D(R) = exp (6) Express the CG series formula given in (a) as a matrix equation involving the fol- lowing five matrices: two 2 x 2 matrices D(sk) [= exp(-iSky0/h)] in the {[sk, mk)} basis with k = 1, 2, the 4 x 4 transformation matrix UT and its transpose (with their elements being the CG coefficients), and the matrix form of D(R) in the {s, m)} basis (i.e., the (S, S) representation). Verify your result.