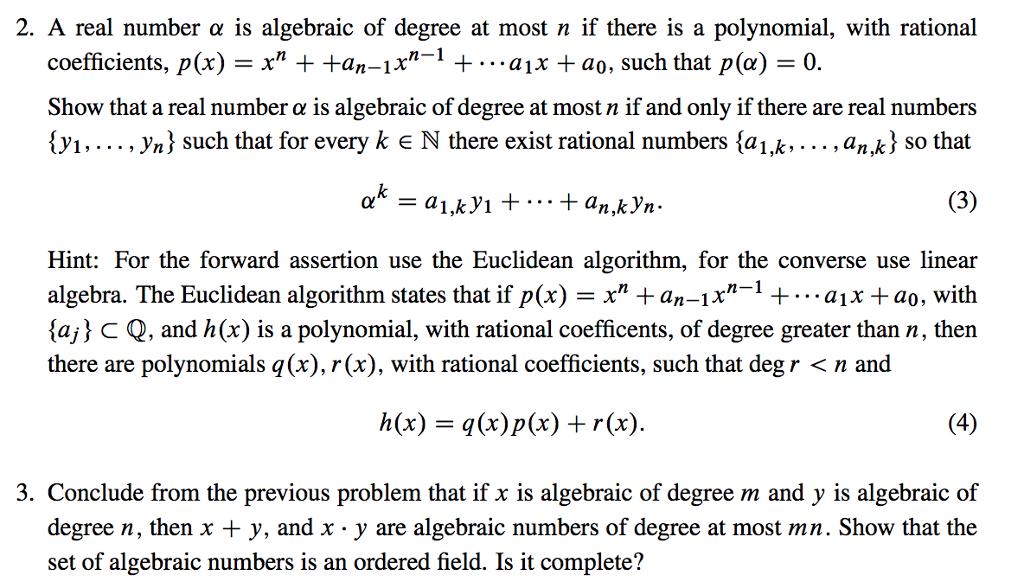
2. A real number is algebraic of degree at most n if there is a polynomial, with rational coefficients, p(x) = = x ++an1xn1 +...ax + ao, such that p(a) = 0. Show that a real number a is algebraic of degree at most n if and only if there are real numbers {y1, yn} such that for every k = N there exist rational numbers {a1,k, ak = a1,ky1 + ... +an,kyn. an,k} so that (3) Hint: For the forward assertion use the Euclidean algorithm, for the converse use linear algebra. The Euclidean algorithm states that if p(x) = x" + an1xn1 +... a1x+ao, with {a} CQ, and h(x) is a polynomial, with rational coefficents, of degree greater than n then there are polynomials q(x), r(x), with rational coefficients, such that deg r < n and h(x) = q(x)p(x)+r(x). (4) 3. Conclude from the previous problem that if x is algebraic of degree m and y is algebraic of degreen, then x + y, and x y are algebraic numbers of degree at most mn. Show that the set of algebraic numbers is an ordered field. Is it complete?