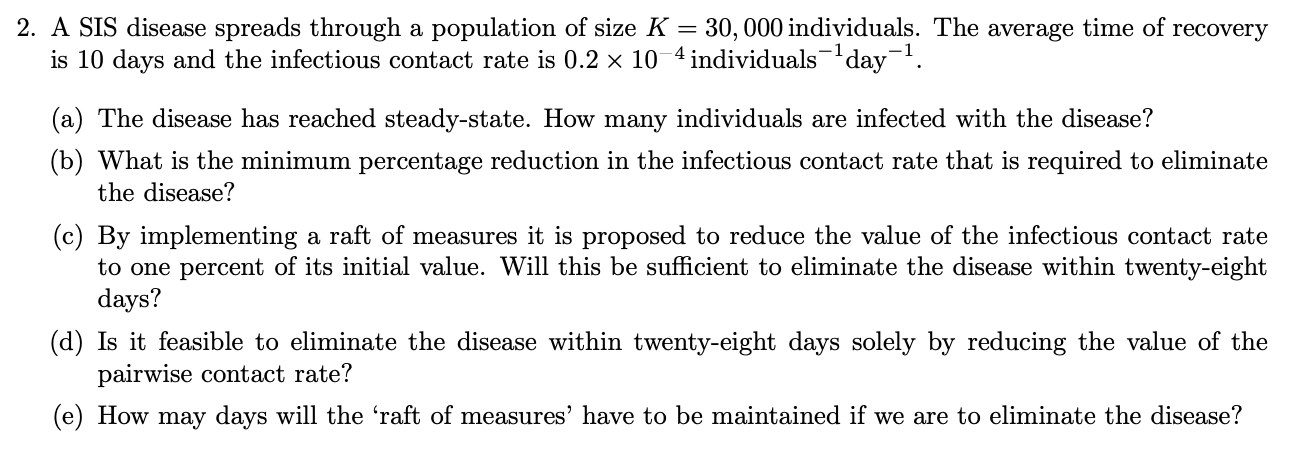
2. A SIS disease spreads through a population of size K = 30, 000 individuals. The average time of recovery is 10 days and the infectious contact rate is 0.2 104 individuals1 day1.

(a) The disease has reached steady-state. How many individuals are infected with the disease?

(b) What is the minimum percentage reduction in the infectious contact rate that is required to eliminate the disease?

(c) By implementing a raft of measures it is proposed to reduce the value of the infectious contact rate to one percent of its initial value. Will this be sufficient to eliminate the disease within twenty-eight days?

(d) Is it feasible to eliminate the disease within twenty-eight days solely by reducing the value of the pairwise contact rate?

(e) How may days will the raft of measures have to be maintained if we are to eliminate the disease?

2. A SIS disease spreads through a population of size K = 30,000 individuals. The average time of recovery is 10 days and the infectious contact rate is 0.2 x 10-4 individuals-day-1. (a) The disease has reached steady-state. How many individuals are infected with the disease? (b) What is the minimum percentage reduction in the infectious contact rate that is required to eliminate the disease? By implementing a raft of measures it is proposed to reduce the value of the infectious contact rate to one percent of its initial value. Will this be sufficient to eliminate the disease within twenty-eight days? (d) Is it feasible to eliminate the disease within twenty-eight days solely by reducing the value of the pairwise contact rate? (e) How may days will the raft of measures' have to be maintained if we are to eliminate the disease? 2. A SIS disease spreads through a population of size K = 30,000 individuals. The average time of recovery is 10 days and the infectious contact rate is 0.2 x 10-4 individuals-day-1. (a) The disease has reached steady-state. How many individuals are infected with the disease? (b) What is the minimum percentage reduction in the infectious contact rate that is required to eliminate the disease? By implementing a raft of measures it is proposed to reduce the value of the infectious contact rate to one percent of its initial value. Will this be sufficient to eliminate the disease within twenty-eight days? (d) Is it feasible to eliminate the disease within twenty-eight days solely by reducing the value of the pairwise contact rate? (e) How may days will the raft of measures' have to be maintained if we are to eliminate the disease