Question
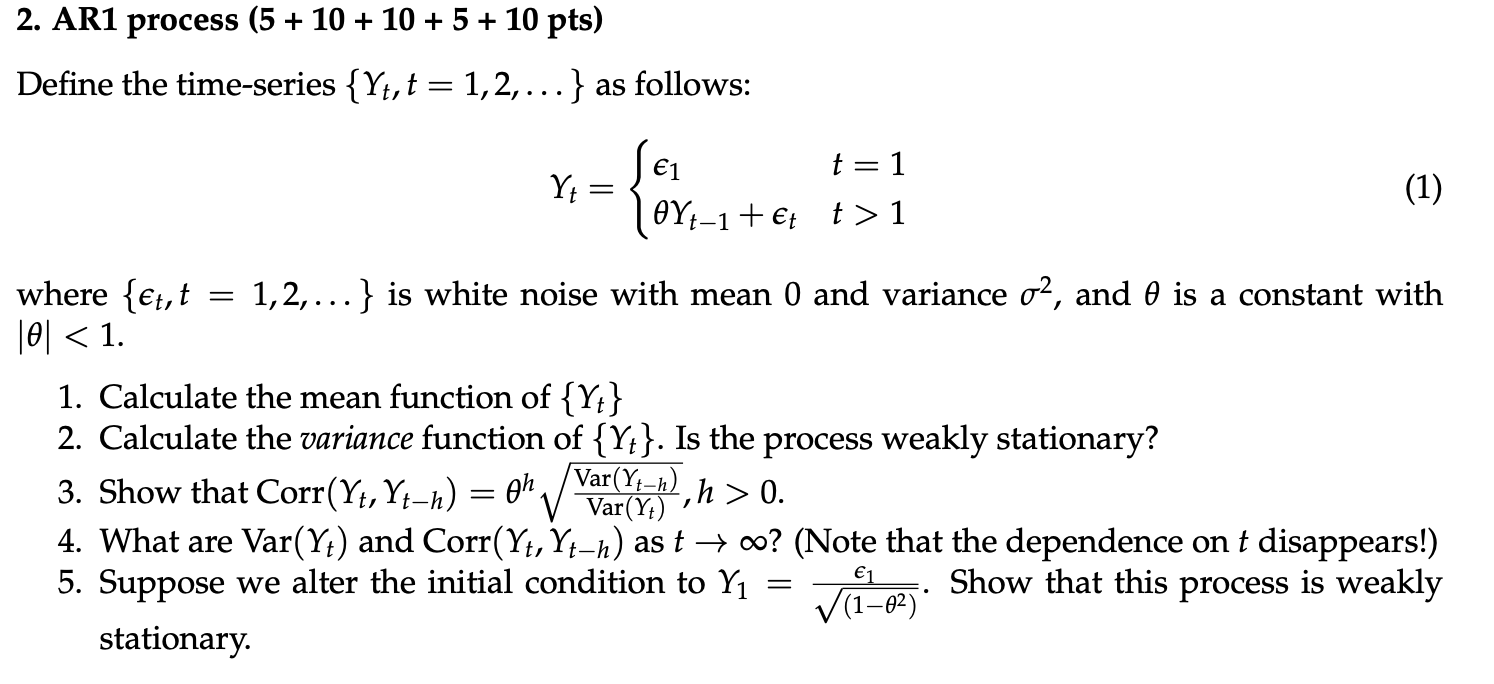
2. AR1 process (5 + 10 + 10 + 5 + 10 pts) Define the time-series {Yt , t = 1, 2, . . .
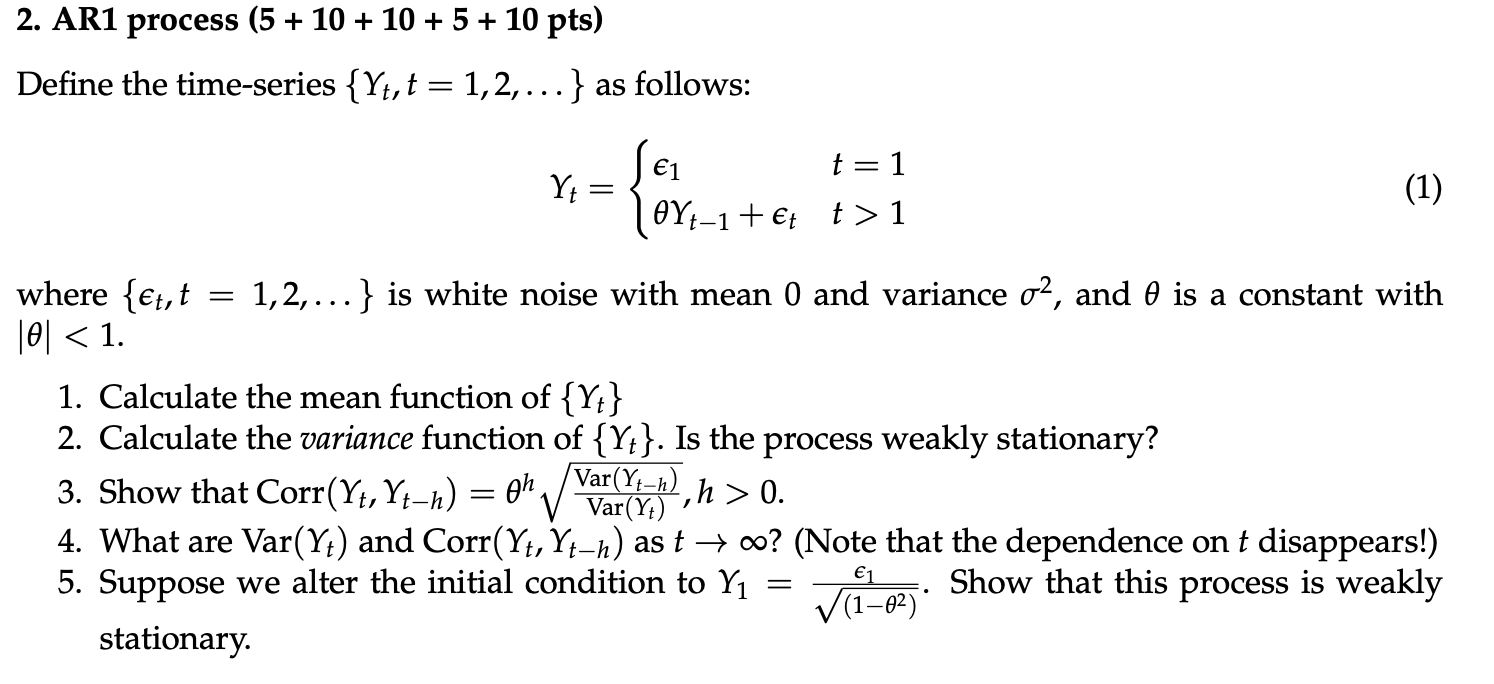
2. AR1 process (5 + 10 + 10 + 5 + 10 pts) Define the time-series {Yt , t = 1, 2, . . . } as follows: Yt = ( e1 t = 1 ?Yt?1 + et t > 1 (1) where {et , t = 1, 2, . . . } is white noise with mean 0 and variance ? 2 , and ? is a constant with |?|
1. Calculate the mean function of {Yt}
2. Calculate the variance function of {Yt}. Is the process weakly stationary?
3. Show that Corr(Yt ,Yt?h) = ? h qVar(Yt?h) Var(Yt) , h > 0. 4. What are Var(Yt) and Corr(Yt ,Yt?h) as t ? ?? (Note that the dependence on t disappears!) 5. Suppose we alter the initial condition to Y1 = ? e1 (1?? 2) . Show that this process is weakly stationary
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


