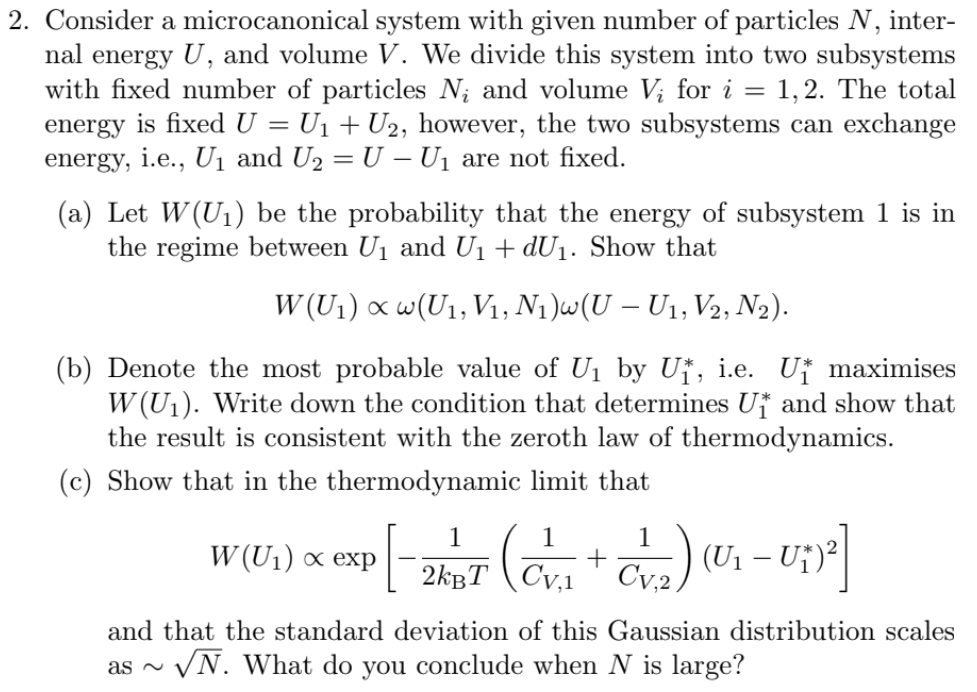
2. Consider a microcanonical system with given number of particles N, inter- nal energy U, and volume V. We divide this system into two subsystems with fixed number of particles N; and volume V for i = 1, 2. The total energy is fixed U = U + U, however, the two subsystems can exchange energy, i.e., U and U = U - U are not fixed. (a) Let W(U) be the probability that the energy of subsystem 1 is in the regime between U and U + dU. Show that W (U) x w(U, V, N)w(U U, V2, N). (b) Denote the most probable value of U by U, i.e. U maximises W(U). Write down the condition that determines U and show that the result is consistent with the zeroth law of thermodynamics. (c) Show that in the thermodynamic limit that W(U) exp 1 1 1 [ (Cv + OV) (U - U)] 2KBT CV.1 Cv.2 and that the standard deviation of this Gaussian distribution scales as ~ N. What do you conclude when N is large?