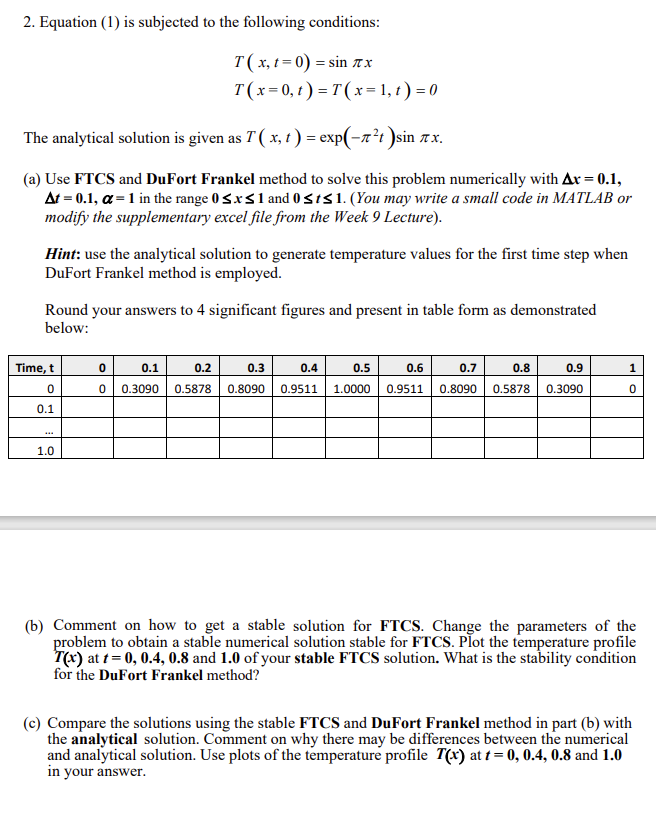
2. Equation (1) is subjected to the following conditions: T(x, t= 0) = sin 7x T(x=0, t) = T(x= 1,t) = 0 The analytical solution is given as T ( x, t) = exp(-7t )sin 7x. (a) Use FTCS and DuFort Frankel method to solve this problem numerically with Ax = 0.1, At = 0.1, = 1 in the range 0x1 and 0 t1. (You may write a small code in MATLAB or modify the supplementary excel file from the Week 9 Lecture). Hint: use the analytical solution to generate temperature values for the first time step when DuFort Frankel method is employed. Round your answers to 4 significant figures and present in table form as demonstrated below: Time, t 0 0.1 *** 1.0 0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 0 0.3090 0.5878 0.8090 0.9511 1.0000 0.9511 0.8090 0.5878 0.3090 1 0 (b) Comment on how to get a stable solution for FTCS. Change the parameters of the problem to obtain a stable numerical solution stable for FTCS. Plot the temperature profile T(x) at t=0, 0.4, 0.8 and 1.0 of your stable FTCS solution. What is the stability condition for the DuFort Frankel method? (c) Compare the solutions using the stable FTCS and DuFort Frankel method in part (b) with the analytical solution. Comment on why there may be differences between the numerical and analytical solution. Use plots of the temperature profile T(x) at t= 0, 0.4, 0.8 and 1.0 in your answer.