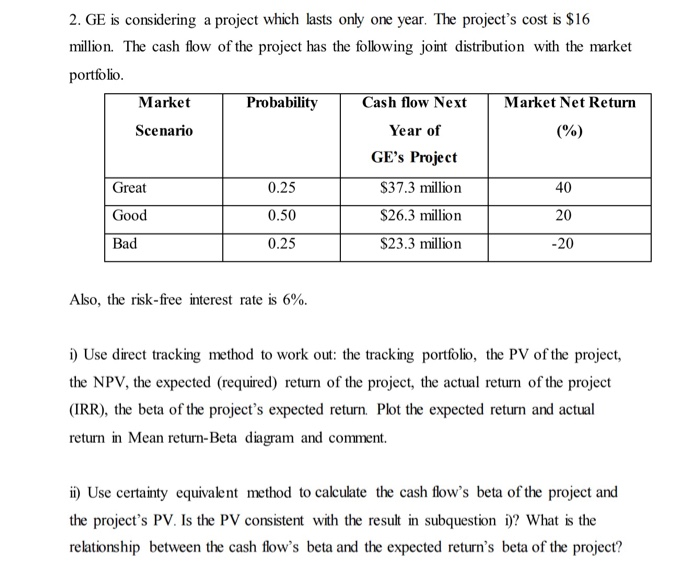
2. GE is considering a project which lasts only one year. The project's cost is $16 million. The cash flow of the project has the following joint distribution with the market portfolio Market Probability Cash flow Next Year of GE's Project $37.3 million $26.3 million $23.3 illion Market Net Return Scenario Great Good Bad 0.25 0.50 0.25 40 20 -20 Also, the risk-free interest rate is 6%. i) Use direct tracking method to work out: the tracking portfolio, the PV of the project, the NPV, the expected (required) return of the project, the actual return of the project (IRR), the beta of the project's expected return. Plot the expected return and actual return in Mean return-Beta diagram and comment i) Use certainty equivalent method to calculate the cash flow's beta of the project and the project's PV. Is the PV consistent with the result in subquestion i)? What is the relationship between the cash flow's beta and the expected return's beta of the project? ii) Based on the expected return's beta you have worked out in subquestion i), use risk- adjusted discount rate method to compute the PV again and verify that the result by this approach is consistent with the ones by the previous two approaches. 2. GE is considering a project which lasts only one year. The project's cost is $16 million. The cash flow of the project has the following joint distribution with the market portfolio Market Probability Cash flow Next Year of GE's Project $37.3 million $26.3 million $23.3 illion Market Net Return Scenario Great Good Bad 0.25 0.50 0.25 40 20 -20 Also, the risk-free interest rate is 6%. i) Use direct tracking method to work out: the tracking portfolio, the PV of the project, the NPV, the expected (required) return of the project, the actual return of the project (IRR), the beta of the project's expected return. Plot the expected return and actual return in Mean return-Beta diagram and comment i) Use certainty equivalent method to calculate the cash flow's beta of the project and the project's PV. Is the PV consistent with the result in subquestion i)? What is the relationship between the cash flow's beta and the expected return's beta of the project? ii) Based on the expected return's beta you have worked out in subquestion i), use risk- adjusted discount rate method to compute the PV again and verify that the result by this approach is consistent with the ones by the previous two approaches