Question: 2. In a three-dimensional vector space, consider the operator whose matrix in an orthornormal basis (1), 2), 3)} is 0 0 A=0 -1 0

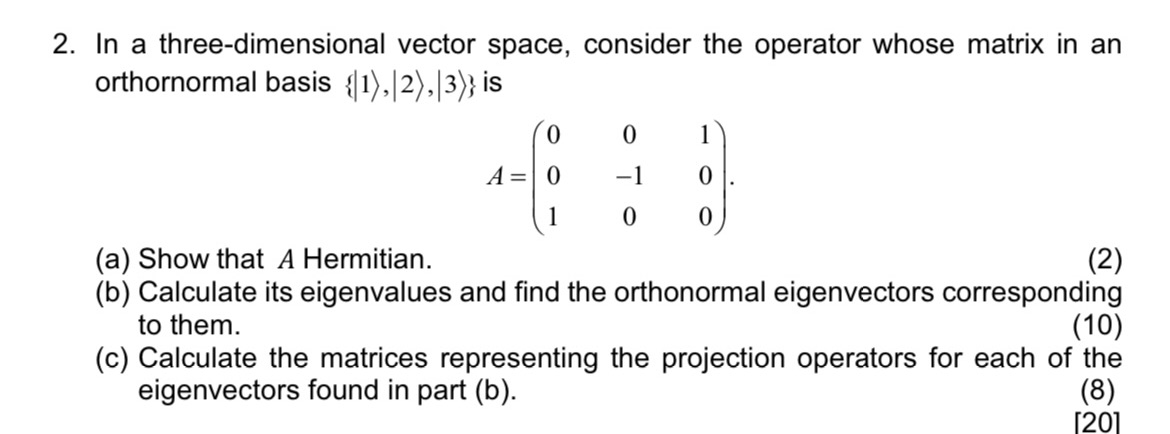
2. In a three-dimensional vector space, consider the operator whose matrix in

2. In a three-dimensional vector space, consider the operator whose matrix in an orthornormal basis (1), 2), 3)} is 0 0 A=0 -1 0 1 0 0 19 (a) Show that A Hermitian. (2) (b) Calculate its eigenvalues and find the orthonormal eigenvectors corresponding to them. (10) (c) Calculate the matrices representing the projection operators for each of the eigenvectors found in part (b). (8) [201

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Physics Questions!

Tesla uses a perpetual inventory system to make inventory decisions about a replacement part for a Model X . Annual demand has been forecast to be 2 , 1 0 0 units and the business operates 3 0 0 days...

CANMNMM January of this year. (a) Each item will be held in a record. Describe all the data structures that must refer to these records to implement the required functionality. Describe all the...

QUIZ... Let D be a poset and let f : D D be a monotone function. (i) Give the definition of the least pre-fixed point, fix (f), of f. Show that fix (f) is a fixed point of f. [5 marks] (ii) Show that...

Consider the following NLP problem: Maximize 2Xi + X2 - 2X3 + 3XiX2 + 1X subject to the constraints (a) Set up and solve the model by using Solver. Use a starting value of zero for each decision...

Discuss the rationale for regulating the presentation and disclosure of the income statement. Describe the differences you would expect to notice in an income statement prepared for an entity...

=+1. Determine the annual depreciation for each of the five years of estimated useful life of the equipment, the accumulated depreciation at the end of each year, and the book value of the equipment...

3. If you want students to become better writers, give many opportunities for writing and rewriting.

In business circles, one frequently hears references to the old boy network. Many women in professional firms complain that their gender precludes them from becoming a member of the old boy network...

If you invested $100,000 today in a fund that earns 12% compounded annually, to what amount will the investment grow in 5 years?

State University has planned six special catered events for the Saturday of its homecoming football game. The events include an alumni brunch, a parents brunch, a booster club luncheon, a postgame...

Use the information Table 7.2 for Polaris Corporation to answer the following question(s). Polaris is taking out a $5,000,000 two-year loan at a variable rate of LIBOR plus 1.00%. The LIBOR rate will...

huck and his employees have discussed redesigning the process to improve efficiency. Suppose they can increase the rate to 320 boxes per day. What will be their new productivity

Free-Response Questions 1. Suppose there are 100 residents on the island of Sunshine. Whenever someone purchases a palm tree to plant on the island, everyone on the island receives the same benefits...

manufacturing has its challenges, especially when the product is high production quantities at low margins. This has made quality inspection more common place in manufacturing processes. Which points...

Refer to Step 3.3. In the "Constrained" or "Long Only" version of the optimal risky portfolio, what is the portfolio standard deviation? answer as a percentage, with no percentage symbol ("%"),...

As a security operative for a Fortune 1000 company, how would I protect my organization's assets?

A folder is a collection of files. ( 1 point ) True False

Use this circle graph to answer following Exercises. 1. What fraction of areas maintained by the National Park Service are designated as National Recreation Areas? 2. What fraction of areas...

Of the atoms with Z 10, which have ground states of odd parity?

For 1, 3-butadiene: (a) How many canonical covalent VB structures are there for the electrons? (b) Draw these structures. (c) Draw the 12 individual singly polar ionic structures for the electrons.

Evaluate (a) (b) 147 325 3112 1462 5062 2865

Childrens perceptions of their neighborhood. In Health Education Research (Feb. 2005), nutrition scientists at Deakin University (Australia) investigated childrens perceptions of their environments....

Consider the following 2 * 3 (i.e., r = 2 and c = 3) contingency table. a. Specify the null and alternative hypotheses that should be used in testing the independence of the row and column...

Test the null hypothesis of independence of the two classifications A and B of the 3 * 3 contingency table shown here. Use a = .05. B B B B3 A1 A Az Az 482 40 72 42 63 53 31 38 228 70 30