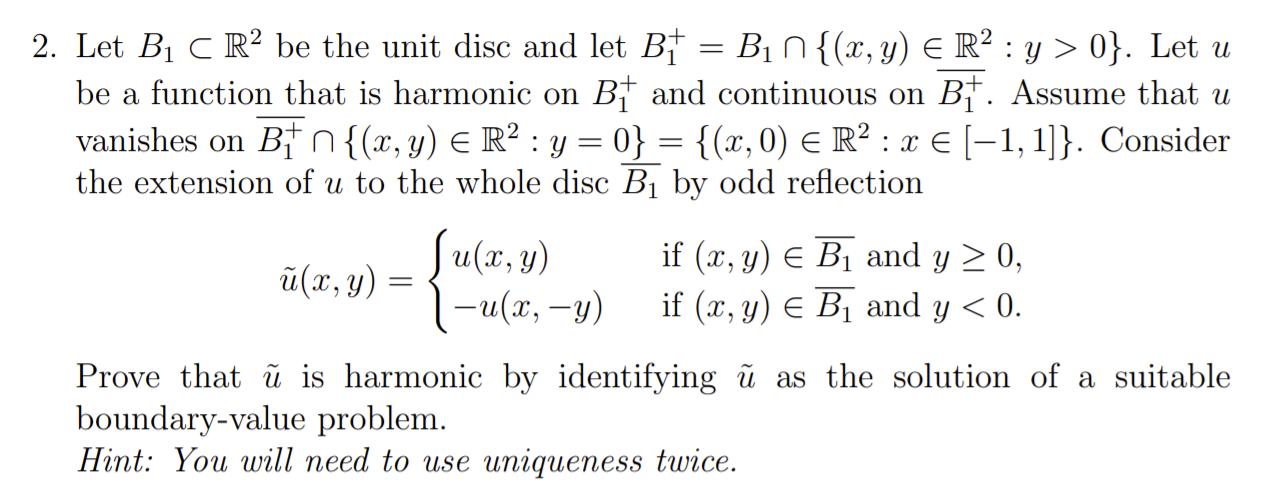
2. Let B1 C R? be the unit disc and let B = B1n {(x, y) E R : y > 0}. Let u be a function that is harmonic on B and continuous on B. Assume that u vanishes on Bn{(x,y) E R : y = 0} = {(x,0) E R : x E [-1, 1]}. Consider the extension of u to the whole disc B1 by odd reflection Su(x, y) 1-u(x, -y) if (x, y) E B1 and y 2 0, if (x, y) E B1 and y < 0. (x, y) Prove that u is harmonic by identifying as the solution of a suitable boundary-value problem. Hint: You will need to use uniqueness twice.