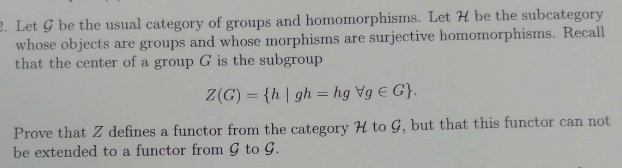
2. Let G be the usual category of groups and homomorphisms. Let H be the subcategory whose objects are groups and whose morphisms are surjective homomorphisms. Recall that the center of a group G is the subgroup Z(G)= {h ghhg Vg G}. Prove that Z defines a functor from the category H to G, but that this functor can not be extended to a functor from G to G.